PHY2403H Quantum Field Theory. Lecture 9: Unbroken and spontaneously broken symmetries, Higgs Lagrangian, scale invariance, Lorentz invariance, angular momentum quantization. Taught by Prof. Erich Poppitz

October 11, 2018 phy2403 , , , , , , , , , , , , , , ,

[Click here for a PDF of this post with nicer formatting (and a Mathematica listing that I didn’t include in this blog post’s latex export)]

DISCLAIMER: Very rough notes from class, with some additional side notes.

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

Last time

We followed a sequence of operations

  1. Noether’s theorem
  2. \( \rightarrow \) conserved currents
  3. \( \rightarrow \) charges (classical)
  4. \( \rightarrow \) “correspondence principle”
  5. \( \rightarrow \hatQ \)
  • Hermitian operators
  • “generators of symmetry”
    \begin{equation}\label{eqn:qftLecture9:20}
    \hatU(\alpha) = e^{i \alpha \hatQ}
    \end{equation}
    We found
    \begin{equation}\label{eqn:qftLecture9:40}
    \hatU(\alpha) \phihat \hatU^\dagger(\alpha) = \phihat + i \alpha \antisymmetric{\hatQ}{\phihat} + \cdots
    \end{equation}

Example: internal symmetries:

(non-spacetime), such as \( O(N)\) or \( U(1) \).

In QFT internal symmetries can have different “\underline{modes of realization}”.

    [I]

  1. “Wigner mode”. These are also called “unbroken symmetries”.
    \begin{equation}\label{eqn:qftLecture9:60}
    \hatQ \ket{0} = 0
    \end{equation}
    i.e. \( \hatU(\alpha) \ket{0} = 0 \).
    Ground state invariant. Formally \( :\hatQ: \) annihilates \( \ket{0} \).
    \( \antisymmetric{\hatQ}{\hatH} = 0 \) implies that all eigenstates are eigenstates of \( \hatQ \) in \( U(1) \). Example from HW 1
    \begin{equation}\label{eqn:qftLecture9:80}
    \hatQ = \text{“charge” under \( U(1) \)}.
    \end{equation}
    All states have definite charge, just live in QU.
  2. “Nambu-Goldstone mode” (Landau-ginsburg). This is also called a “spontaneously broken symmetry”\footnote{
    First encounter example (HWII, \( SU(2) \times SU(2) \rightarrow SU(2) \)). Here a \( U(1) \) spontaneous broken symmetry.}.
    \( H \) or \( L \) is invariant under symmetry, but ground state is not.

fig. 1. Mexican hat potential.

fig. 2. Degenerate Mexican hat potential ( v = 0)

Example:
\begin{equation}\label{eqn:qftLecture9:100}
\LL = \partial_\mu \phi^\conj \partial^\mu \phi – V(\Abs{\phi}),
\end{equation}
where
\begin{equation}\label{eqn:qftLecture9:120}
V(\Abs{\phi}) = m^2 \phi^\conj \phi + \frac{\lambda}{4} \lr{ \phi^\conj \phi }^2.
\end{equation}
When \( m^2 > 0 \) we have a Wigner mode, but when \( m^2 < 0 \) we have an issue: \( \phi = 0 \) is not a minimum of potential.
When \( m^2 < 0 \) we write
\begin{equation}\label{eqn:qftLecture9:140}
\begin{aligned}
V(\phi)
&= – m^2 \phi^\conj \phi + \frac{\lambda}{4} \lr{ \phi^\conj \phi}^2 \\
&= \frac{\lambda}{4} \lr{
\lr{ \phi^\conj \phi}^2 – \frac{4}{\lambda} m^2 } \\
&= \frac{\lambda}{4} \lr{
\phi^\conj \phi – \frac{2}{\lambda} m^2 }^2 – \frac{4 m^4}{\lambda^2},
\end{aligned}
\end{equation}
or simply
\begin{equation}\label{eqn:qftLecture9:780}
V(\phi)
=
\frac{\lambda}{4} \lr{ \phi^\conj \phi – v^2 }^2 + \text{const}.
\end{equation}
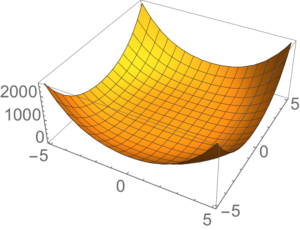
The potential (called the Mexican hat potential) is illustrated in fig. 1 for non-zero \( v \), and in
fig. 2 for \( v = 0 \).
We choose to expand around some point on the minimum ring (it doesn’t matter which one).
When there is no potential, we call the field massless (i.e. if we are in the minimum ring).
We expand as
\begin{equation}\label{eqn:qftLecture9:160}
\phi(x) = v \lr{ 1 + \frac{\rho(x)}{v} } e^{i \alpha(x)/v },
\end{equation}
so
\begin{equation}\label{eqn:qftLecture9:180}
\begin{aligned}
\frac{\lambda}{4}
\lr{\phi^\conj \phi – v^2}^2
&=
\lr{
v^2 \lr{ 1 + \frac{\rho(x)}{v} }^2
– v^2
}^2 \\
&=
\frac{\lambda}{4}
v^4 \lr{ \lr{ 1 + \frac{\rho(x)}{v} }^2 – 1 } \\
&=
\frac{\lambda}{4}
v^4
\lr{
\frac{2 \rho}{v} + \frac{\rho^2}{v^2}
}^2.
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture9:200}
\partial_\mu \phi =
\lr{
v \lr{ 1 + \frac{\rho(x)}{v} } \frac{i}{v} \partial_\mu \alpha
+ \partial_\mu \rho
} e^{i \alpha}
\end{equation}

so
\begin{equation}\label{eqn:qftLecture9:220}
\begin{aligned}
\LL
&= \Abs{\partial \phi^\conj}^2 – \frac{\lambda}{4} \lr{ \Abs{\phi^\conj}^2 – v^2 }^2 \\
&=
\partial_\mu \rho \partial^\mu \rho + \partial_\mu \alpha \partial^\mu \alpha \lr{ 1 + \frac{\rho}{v} }
–
\frac{\lambda v^4}{4} \frac{ 4\rho^2}{v^2} + O(\rho^3) \\
&=
\partial_\mu \rho \partial^\mu \rho
– \lambda v^2\rho^2
+
\partial_\mu \alpha \partial^\mu \alpha \lr{ 1 + \frac{\rho}{v} }.
\end{aligned}
\end{equation}
We have two fields, \( \rho \) : a massive scalar field, the “Higgs”, and a massless field \( \alpha \) (the Goldstone Boson).

\( U(1) \) symmetry acts on \( \phi(x) \rightarrow e^{i \omega } \phi(x) \) i.t.o \( \alpha(x) \rightarrow \alpha(x) + v \omega \).
\( U(1) \) global symmetry (broken) acts on the Goldstone field \( \alpha(x) \) by a constant shift. (\(U(1)\) is still a symmetry of the Lagrangian.)

The current of the \( U(1) \) symmetry is:
\begin{equation}\label{eqn:qftLecture9:240}
j_\mu = \partial_\mu \alpha \lr{ 1 + \text{higher dimensional \( \rho \) terms} }.
\end{equation}

When we quantize
\begin{equation}\label{eqn:qftLecture9:260}
\alpha(x) =
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} e^{i \omega_p t – i \Bp \cdot \Bx} \hat{a}_\Bp^\dagger +
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} e^{-i \omega_p t + i \Bp \cdot \Bx} \hat{a}_\Bp
\end{equation}
\begin{equation}\label{eqn:qftLecture9:280}
j^\mu(x) = \partial^\mu \alpha(x) =
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} \lr{ i \omega_\Bp – i \Bp } e^{i \omega_p t – i \Bp \cdot \Bx} \hat{a}_\Bp^\dagger +
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} \lr{ -i \omega_\Bp + i \Bp } e^{-i \omega_p t + i \Bp \cdot \Bx} \hat{a}_\Bp.
\end{equation}

\begin{equation}\label{eqn:qftLecture9:300}
j^\mu(x) \ket{0} \ne 0,
\end{equation}
instead it creates a single particle state.

Examples of symmetries

In particle physics, examples of Wigner vs Nambu-Goldstone, ignoring gravity the only exact internal symmetry in the standard module is
\( (B\# – L\#) \), believed to be a \( U(1) \) symmetry in Wigner mode.

Here \(B\#\) is the Baryon number, and \( L\# \) is the Lepton number. Examples:

  • \( B(p) = 1 \), proton.
  • \( B(q) = 1/3 \), quark
  • \( B(e) = 1 \), electron
  • \( B(n) = 1 \), neutron.
  • \( L(p) = 1 \), proton.
  • \( L(q) = 0 \), quark.
  • \( L(e) = 0 \), electron.

The major use of global internal symmetries in the standard model is as “approximate” ones. They become symmetries when one neglects some effect( “terms in \( \LL \)”).
There are other approximate symmetries (use of group theory to find the Balmer series).

Example from HW2:

QCD in limit
\begin{equation}\label{eqn:qftLecture9:320}
m_u = m_d = 0.
\end{equation}
\( m_u m_d \ll m_p \) (the products of the up-quark mass and the down-quark mass are much less than a composite one (name?)).
\( SU(2)_L \times SU(2)_R \rightarrow SU(2)_V \)

EWSB (Electro-Weak-Symmetry-Breaking) sector

When the couplings \( g_2, g_1 = 0 \). (\( g_2 \in SU(2), g_1 \in U(1) \)).

Scale invariance

\begin{equation}\label{eqn:qftLecture9:340}
\begin{aligned}
x &\rightarrow e^{\lambda} x \\
\phi &\rightarrow e^{-\lambda} \phi \\
A_\mu &\rightarrow e^{-\lambda} A_\mu
\end{aligned}
\end{equation}
Any unitary theory which is scale invariant is also \underline{conformal} invariant. Conformal invariance means that angles are preserved.
The point here is that there is more than scale invariance.

We have classical internal global continuous symmetries.
These can be either

  1. “unbroken” (Wigner mode)
    \begin{equation}\label{eqn:qftLecture9:360}
    \hatQ\ket{0} = 0.
    \end{equation}
  2. “spontaneously broken”
    \begin{equation}\label{eqn:qftLecture9:380}
    j^\mu(x) \ket{0} \ne 0
    \end{equation}
    (creates Goldstone modes).
  3. “anomalous”. Classical symmetries are not a symmetry of QFT.
    Examples:

    • Scale symmetry (to be studied in QFT II), although this is not truly internal.
    • In QCD again when \( \omega_\Bq = 0 \), a \( U(1\) symmetry (chiral symmetry) becomes exact, and cannot be preserved in QFT.
    • In the standard model (E.W sector), the Baryon number and Lepton numbers are not symmetries, but their difference \( B\# – L\# \) is a symmetry.

Lorentz invariance.

We’d like to study the action of Lorentz symmetries on quantum states. We are going to “go by the book”, finding symmetries, currents, quantize, find generators, and so forth.

Under a Lorentz transformation
\begin{equation}\label{eqn:qftLecture9:400}
x^\mu \rightarrow {x’}^\mu = {\Lambda^\mu}_\nu x^\nu,
\end{equation}
We are going to consider infinitesimal Lorentz transformations
\begin{equation}\label{eqn:qftLecture9:420}
{\Lambda^\mu}_\nu \approx
{\delta^\mu}_\nu + {\omega^\mu}_\nu
,
\end{equation}
where \( {\omega^\mu}_\nu \) is small.
A Lorentz transformation \( \Lambda \) must satisfy \( \Lambda^\T G \Lambda = G \), or
\begin{equation}\label{eqn:qftLecture9:800}
g_{\mu\nu} = {{\Lambda}^\alpha}_\mu g_{\alpha \beta} {{\Lambda}^\beta}_\nu,
\end{equation}
into which we insert the infinitesimal transformation representation
\begin{equation}\label{eqn:qftLecture9:820}
\begin{aligned}
0
&=
– g_{\mu\nu} +
\lr{ {\delta^\alpha}_\mu + {\omega^\alpha}_\mu }
g_{\alpha \beta}
\lr{ {\delta^\beta}_\nu + {\omega^\beta}_\nu } \\
&=
– g_{\mu\nu} +
\lr{
g_{\mu \beta}
+
\omega_{\beta\mu}
}
\lr{ {\delta^\beta}_\nu + {\omega^\beta}_\nu } \\
&=
– g_{\mu\nu} +
g_{\mu \nu}
+
\omega_{\nu\mu}
+
\omega_{\mu\nu}
+
\omega_{\beta\mu}
{\omega^\beta}_\nu.
\end{aligned}
\end{equation}
The quadratic term can be ignored, leaving just
\begin{equation}\label{eqn:qftLecture9:840}
0 =
\omega_{\nu\mu}
+
\omega_{\mu\nu},
\end{equation}
or
\begin{equation}\label{eqn:qftLecture9:860}
\omega_{\nu\mu} = – \omega_{\mu\nu}.
\end{equation}
Note that \( \omega \) is a completely antisymmetric tensor, and like \( F_{\mu\nu} \) this has only 6 elements.
This means that the
infinitesimal transformation of the coordinates is
\begin{equation}\label{eqn:qftLecture9:440}
x^\mu \rightarrow {x’}^\mu \approx x^\mu + \omega^{\mu\nu} x_\nu,
\end{equation}
the field transforms as
\begin{equation}\label{eqn:qftLecture9:460}
\phi(x) \rightarrow \phi'(x’) = \phi(x)
\end{equation}
or
\begin{equation}\label{eqn:qftLecture9:760}
\phi'(x^\mu + \omega^{\mu\nu} x_\nu) =
\phi'(x) + \omega^{\mu\nu} x_\nu \partial_\mu\phi(x) = \phi(x),
\end{equation}
so
\begin{equation}\label{eqn:qftLecture9:480}
\delta \phi = \phi'(x) – \phi(x) =
-\omega^{\mu\nu} x_\nu \partial_\mu \phi.
\end{equation}

Since \( \LL \) is a scalar
\begin{equation}\label{eqn:qftLecture9:500}
\begin{aligned}
\delta \LL
&=
-\omega^{\mu\nu} x_\nu \partial_\mu \LL \\
&=
–
\partial_\mu \lr{
\omega^{\mu\nu} x_\nu \LL
}
+
(\partial_\mu x_\nu) \omega^{\mu\nu} \LL \\
&=
\partial_\mu \lr{
–
\omega^{\mu\nu} x_\nu \LL
},
\end{aligned}
\end{equation}
since \( \partial_\nu x_\mu = g_{\nu\mu} \) is symmetric, and \( \omega \) is antisymmetric.
Our current is
\begin{equation}\label{eqn:qftLecture9:520}
J^\mu_\omega
=
–
\omega^{\mu\nu} x_\mu \LL
.
\end{equation}
Our Noether current is
\begin{equation}\label{eqn:qftLecture9:540}
\begin{aligned}
j^\nu_{\omega^{\mu\rho}}
&= \PD{\phi_{,\nu}}{\LL} \delta \phi – J^\mu_\omega \\
&=
\partial^\nu \phi\lr{ – \omega^{\mu\rho} x_\rho \partial_\mu \phi } + \omega^{\nu \rho} x_\rho \LL \\
&=
\omega^{\mu\rho}
\lr{
\partial^\nu \phi\lr{ – x_\rho \partial_\mu \phi } + {\delta^{\nu}}_\mu x_\rho \LL
} \\
&=
\omega^{\mu\rho} x_\rho
\lr{
-\partial^\nu \phi \partial_\mu \phi + {\delta^{\nu}}_\mu \LL
}
\end{aligned}
\end{equation}
We identify
\begin{equation}\label{eqn:qftLecture9:560}
–
{T^\nu}_\mu =
-\partial^\nu \phi \partial_\mu \phi + {\delta^{\nu}}_\mu \LL,
\end{equation}
so the current is
\begin{equation}\label{eqn:qftLecture9:580}
\begin{aligned}
j^\nu_{\omega_{\mu\rho}}
&=
-\omega^{\mu\rho} x_\rho
{T^\nu}_\mu \\
&=
-\omega_{\mu\rho} x^\rho
T^{\nu\mu}
.
\end{aligned}
\end{equation}
Define
\begin{equation}\label{eqn:qftLecture9:600}
j^{\nu\mu\rho} = \inv{2} \lr{ x^\rho T^{\nu\mu} – x^{\mu} T^{\nu\rho} },
\end{equation}
which retains the antisymmetry in \( \mu \rho \) yet still drops the parameter \( \omega^{\mu\rho} \).
To check that this makes sense, we can contract
\( j^{\nu\mu\rho} \) with \( \omega_{\rho\mu} \)
\begin{equation}\label{eqn:qftLecture9:880}
\begin{aligned}
j^{\nu\mu\rho} \omega_{\rho\mu}
&= -\inv{2} \lr{ x^\rho T^{\nu\mu} – x^{\mu} T^{\nu\rho} }
\omega_{\mu\rho} \\
&=
-\inv{2} x^\rho T^{\nu\mu}
\omega_{\mu\rho}
– \inv{2} x^{\mu} T^{\nu\rho}
\omega_{\rho\mu} \\
&=
-\inv{2} x^\rho T^{\nu\mu}
\omega_{\mu\rho}
– \inv{2} x^{\rho} T^{\nu\mu}
\omega_{\mu\rho} \\
&=
– x^{\rho} T^{\nu\mu}
\omega_{\mu\rho},
\end{aligned}
\end{equation}
which matches \ref{eqn:qftLecture9:580} as desired.

Example. Rotations \( \mu\rho = ij \)

\begin{equation}\label{eqn:qftLecture9:620}
\begin{aligned}
J^{0 i j} \epsilon_{ijk}
&=
\inv{2} \lr{ x^i T^{0j} – x^{j} T^{0i} } \epsilon_{ijk} \\
&=
x^i T^{0j} \epsilon_{ijk}.
\end{aligned}
\end{equation}
Observe that this has the structure of \( (\Bx \cross \Bp)_k \), where \( \Bp \) is the momentum density of the field.
Let
\begin{equation}\label{eqn:qftLecture9:640}
L_k \equiv Q_k = \int d^3 x J^{0ij} \epsilon_{ijk}.
\end{equation}
We can now quantize and build a generator
\begin{equation}\label{eqn:qftLecture9:660}
\begin{aligned}
\hatU(\Balpha)
&= e^{i \Balpha \cdot \hat{\BL}} \\
&= \exp\lr{i \alpha_k
\int d^3 x x^i \hat{T}^{0j} \epsilon_{ijk}
}
\end{aligned}
\end{equation}
From \ref{eqn:qftLecture9:560} we can quantize with \( T^{0j} = \partial^0 \phi \partial^j \phi \rightarrow \hat{\pi} \lr{\spacegrad \phihat}_j\), or
\begin{equation}\label{eqn:qftLecture9:900}
\begin{aligned}
\hatU(\Balpha)
&=
\exp\lr{i \alpha_k
\int d^3 x x^i \hat{\pi} (\spacegrad \phihat)_j \epsilon_{ijk}
} \\
&=
\exp\lr{i \Balpha \cdot
\int d^3 x \hat{\pi} \spacegrad \phihat \cross \Bx
}
\end{aligned}
\end{equation}
(up to a sign in the exponent which doesn’t matter)
\begin{equation}\label{eqn:qftLecture9:680}
\begin{aligned}
\phihat(\By) \rightarrow \hatU(\alpha) \phihat(\By) \hatU^\dagger(\alpha)
&\approx
\phihat(\By) +
i \Balpha \cdot
\antisymmetric{
\int d^3 x \hat{\pi}(\Bx) \spacegrad \phihat(\Bx) \cross \Bx
}
{
\phihat(\By)
} \\
&=
\phihat(\By) +
i \Balpha \cdot
\int d^3 x
(-i) \delta^3(\Bx – \By)
\spacegrad \phihat(\Bx) \cross \Bx \\
&=
\phihat(\By) +
\Balpha \cdot \lr{ \spacegrad \phihat(\By ) \cross \By}
\end{aligned}
\end{equation}
Explicitly, in coordinates, this is
\begin{equation}\label{eqn:qftLecture9:700}
\begin{aligned}
\phihat(\By)
&\rightarrow
\phihat(\By) +
\alpha^i
\lr{
\partial^j \phihat(\By) y^k \epsilon_{jki}
} \\
&=
\phihat(\By) –
\epsilon_{ikj} \alpha^i y^k \partial^j \phihat \\
&=
\phihat( y^j – \epsilon^{ikj} \alpha^i y^k ).
\end{aligned}
\end{equation}
This is a rotation. To illustrate, pick \( \Balpha = (0, 0, \alpha) \), so \( y^j \rightarrow y^j – \epsilon^{ikj} \alpha y^k \delta_{i3} = y^j – \epsilon^{3kj} \alpha y^k \), or
\begin{equation}\label{eqn:qftLecture9:n}
\begin{aligned}
y^1 &\rightarrow y^1 – \epsilon^{3k1} \alpha y^k = y^1 + \alpha y^2 \\
y^2 &\rightarrow y^2 – \epsilon^{3k2} \alpha y^k = y^2 – \alpha y^1 \\
y^3 &\rightarrow y^3 – \epsilon^{3k3} \alpha y^k = y^3,
\end{aligned}
\end{equation}
or in matrix form
\begin{equation}\label{eqn:qftLecture9:720}
\begin{bmatrix}
y^1 \\
y^2 \\
y^3 \\
\end{bmatrix}
\rightarrow
\begin{bmatrix}
1 & \alpha & 0 \\
-\alpha & 1 & 0 \\
0 & 0 & 1
\end{bmatrix}
\begin{bmatrix}
y^1 \\
y^2 \\
y^3 \\
\end{bmatrix}.
\end{equation}

PHY2403H Quantum Field Theory. Lecture 7: Symmetries, translation currents, energy momentum tensor. Taught by Prof. Erich Poppitz

October 3, 2018 phy2403 , , , , , ,

[Click here for a PDF of this post with nicer formatting]

DISCLAIMER: Very rough notes from class, with some additional side notes.

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

Symmetries

Given the complexities of the non-linear systems we want to investigate, examination of symmetries gives us simpler problems that we can solve.

  • “internal” symmetries. This means that the symmetries do not act on space time \( (\Bx, t) \). An example is
    \begin{equation}\label{eqn:qftLecture7:20}
    \phi^i =
    \begin{bmatrix}
    \psi_1 \\
    \psi_2 \\
    \vdots \\
    \psi_N \\
    \end{bmatrix}
    \end{equation}
    If we map \( \phi^i \rightarrow O^i_j \phi^j \) where \( O^\T O = 1 \), then we call this an internal symmetry.
    The corresponding Lagrangian density might be something like
    \begin{equation}\label{eqn:qftLecture7:40}
    \LL = \inv{2} \partial_\mu \Bphi \cdot \partial^\mu \Bphi – \frac{m^2}{2} \Bphi \cdot \Bphi – V(\Bphi \cdot \Bphi)
    \end{equation}

  • spacetime symmetries: Translations, rotations, boosts, dilatations. We will consider continuous symmetries, which can be defined as a succession of infinitesimal transformations.
    An example from \(O(2)\) is a rotation
    \begin{equation}\label{eqn:qftLecture7:60}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2 \\
    \end{bmatrix}
    \rightarrow
    \begin{bmatrix}
    \cos\alpha & \sin\alpha \\
    -\sin\alpha & \cos\alpha \\
    \end{bmatrix}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2
    \end{bmatrix},
    \end{equation}
    or if \( \alpha \sim 0 \)
    \begin{equation}\label{eqn:qftLecture7:80}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2 \\
    \end{bmatrix}
    \rightarrow
    \begin{bmatrix}
    1 & \alpha \\
    -\alpha & 1\\
    \end{bmatrix}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2
    \end{bmatrix}
    =
    \begin{bmatrix}
    \phi^1 \\
    \phi^2
    \end{bmatrix}
    +
    \alpha
    \begin{bmatrix}
    \phi^2 \\
    -\phi^1
    \end{bmatrix}
    \end{equation}
    In index notation we write
    \begin{equation}\label{eqn:qftLecture7:100}
    \phi^i \rightarrow \phi^i + \alpha e^{ij} \phi^j,
    \end{equation}
    where \( \epsilon^{12} = +1, \epsilon^{21} = -1 \) is the completely antisymmetric tensor. This can be written in more general form as
    \begin{equation}\label{eqn:qftLecture7:120}
    \phi^i \rightarrow \phi^i + \delta \phi^i,
    \end{equation}
    where \( \delta \phi^i \) is considered to be an infinitesimal transformation.

Definition: Symmetry

A symmetry means that there is some transformation
\begin{equation*}
\phi^i \rightarrow \phi^i + \delta \phi^i,
\end{equation*}
where \( \delta \phi^i \) is an infinitesimal transformation, and the equations of motion are invariant under this transformation.

Theorem: Noether’s theorem (1st).

If the equations of motion re invariant under \( \phi^\mu \rightarrow \phi^\mu + \delta \phi^\mu \), then there exists a conserved current \( j^\mu \) such that \( \partial_\mu j^\mu = 0 \).

Noether’s first theorem applies to global symmetries, where the parameters are the same for all \( (\Bx, t)\). Gauge symmetries are not examples of such global symmetries.

Given a Lagrangian density \( \LL(\phi(x), \phi_{,\mu}(x)) \), where \( \phi_{,\mu} \equiv \partial_\mu \phi \). The action is
\begin{equation}\label{eqn:qftLecture7:160}
S = \int d^d x \LL.
\end{equation}
EOMs are invariant if under \( \phi(x) \rightarrow \phi'(x) = \phi(x) + \delta_\epsilon \phi(x)\), we have
\begin{equation}\label{eqn:qftLecture7:180}
\LL(\phi) \rightarrow \LL'(\phi’) = \LL(\phi) + \partial_\mu J_\epsilon^\mu(\phi) + O(\epsilon^2).
\end{equation}
Then there exists a conserved current. In QFT we say that the E.O.M’s are “on shell”. Note that \ref{eqn:qftLecture7:180} is a symmetry since we have added a total derivative to the Lagrangian which leaves the equations of motion of unchanged.

In general, the change of action under arbitrary variation of \( \delta \phi\) of the fields is
\begin{equation}\label{eqn:qftLecture7:200}
\begin{aligned}
\delta S
&=
\int d^d x \delta \LL(\phi, \partial_\mu \phi) \\
&=
\int d^d x \lr{
\PD{\phi}{\LL} \delta \phi
+
\PD{(\partial_\mu \phi)}{\LL} \delta \partial_\mu \phi
} \\
&=
\int d^d x \lr{
\partial_\mu \lr{ \PD{(\partial_\mu \phi)}{\LL} } \delta \phi
+
\PD{(\partial_\mu \phi)}{\LL} \partial_\mu \delta \phi
} \\
&=
\int d^d x
\partial_\mu \lr{ \frac{\delta \LL}{\delta(\partial_\mu \phi)} \delta \phi }
\end{aligned}
\end{equation}
However from \ref{eqn:qftLecture7:180}
\begin{equation}\label{eqn:qftLecture7:220}
\delta_\epsilon \LL = \partial_\mu J_\epsilon^\mu(\phi, \partial_\mu \phi),
\end{equation}
so after equating these variations we fine that
\begin{equation}\label{eqn:qftLecture7:240}
\delta S = \int d^d x \delta_\epsilon \LL = \int d^d x \partial_\mu J_\epsilon^\mu,
\end{equation}
or
\begin{equation}\label{eqn:qftLecture7:260}
0 = \int d^d x
\partial_\mu \lr{ \frac{\delta \LL}{\delta(\partial_\mu \phi)} \delta \phi – J_\epsilon^\mu },
\end{equation}
or \( \partial_\mu j^\mu = 0 \) provided
\begin{equation}\label{eqn:qftLecture7:280}
\boxed{
j^\mu =
\frac{\delta \LL}{\delta(\partial_\mu \phi)} \delta_\epsilon \phi – J_\epsilon^\mu.
}
\end{equation}

Integrating the divergence of the current over a space time volume, perhaps that of cylinder (time up, space out) is also zero. That is
\begin{equation}\label{eqn:qftLecture7:300}
\begin{aligned}
0
&=
\int d^4 x \, \partial_\mu j^\mu \\
&=
\int d^3 \Bx dt \, \partial_\mu j^\mu \\
&=
\int d^3 \Bx dt \, \partial_t j^0 –
\int d^3 \Bx dt \spacegrad \cdot \Bj \\
&=
\int d^3 \Bx dt \, \partial_t j^0 ,
\end{aligned}
\end{equation}
where the spatial divergence is zero assuming there’s no current leaving the volume on the infinite boundary
(no \(\Bj\) at spatial infinity.)

We write
\begin{equation}\label{eqn:qftLecture7:560}
Q = \int d^3x \partial_t j^0,
\end{equation}
and call this the on-shell charge associated with the symmetry.

Spacetime translation.

A spacetime translation has the form
\begin{equation}\label{eqn:qftLecture7:320}
x^\mu \rightarrow {x’}^\mu = x^\mu + a^\mu,
\end{equation}
\begin{equation}\label{eqn:qftLecture7:340}
\phi(x) \rightarrow \phi'(x’) = \phi(x)
\end{equation}
(contrast this to a Lorentz transformation that had the form \( x^\mu \rightarrow {x’}^\mu = {\Lambda^\mu}_\nu x^\nu \)).

If \(\phi'(x + a) = \phi(x) \), then
\begin{equation}\label{eqn:qftLecture7:360}
\phi'(x) + a^\mu \partial_\mu \phi'(x) =
\phi'(x) + a^\mu \partial_\mu \phi(x) =
\phi(x),
\end{equation}
so
\begin{equation}\label{eqn:qftLecture7:380}
\phi'(x)
= \phi(x) – a^\mu \partial_\mu \phi'(x)
= \phi(x) + \delta_a \phi(x),
\end{equation}
or
\begin{equation}\label{eqn:qftLecture7:580}
\delta_a \phi(x) = – a^\mu \partial_\mu \phi(x).
\end{equation}
Under \( \phi \rightarrow \phi – a^\mu \partial_\mu \phi \), we have
\begin{equation}\label{eqn:qftLecture7:400}
\LL(\phi) \rightarrow \LL(\phi) – a^\mu \partial_\mu \LL.
\end{equation}
Let’s calculate this with our scalar theory Lagrangian
\begin{equation}\label{eqn:qftLecture7:420}
\LL = \inv{2} \partial_\mu \phi \partial^\mu \phi – \frac{m^2}{2} \phi^2 – V(\phi)
\end{equation}
The Lagrangian variation is
\begin{equation}\label{eqn:qftLecture7:440}
\begin{aligned}
\evalbar{\delta \LL}{\phi \rightarrow \phi + \delta \phi, \delta\phi = – a^\mu \partial_\mu \phi}
&=
(\partial_\mu \phi) \delta (\partial^\mu \phi) – m^2 \phi \delta \phi – \PD{\phi}{V} \delta \phi \\
&=
(\partial_\mu \phi)(-a^\nu \partial_\nu \phi \partial^\mu \phi) + m^2 \phi a^\nu \partial_\nu \phi + \PD{\phi}{V} a^\nu \partial_\nu \phi \\
&=
– a^\nu \partial_\nu \lr{ \inv{2} \partial_\mu \partial^\mu \phi – \frac{m^2}{2} \phi^2 – V(\phi) } \\
&=
– a^\nu \partial_\nu \LL.
\end{aligned}
\end{equation}

So the current is
\begin{equation}\label{eqn:qftLecture7:600}
\begin{aligned}
j^\mu
&=
(\partial^\mu \phi) (-a^\nu \partial_\nu \phi) + a^\nu \LL \\
&=
-a^\nu \lr{ \partial^\mu \phi \partial_\nu \phi – \LL }
\end{aligned}
\end{equation}

We really have a current for each \( \nu \) direction and can make that explicit writing
\begin{equation}\label{eqn:qftLecture7:460}
\begin{aligned}
\delta_\nu \LL
&= -\partial_\nu \LL \\
&= – \partial_\mu \lr{ {\delta^\mu}_\nu \LL } \\
&= \partial_\mu {J^\mu}_\nu
\end{aligned}
\end{equation}
we write
\begin{equation}\label{eqn:qftLecture7:480}
{j^\mu}_\nu = \PD{x_\mu}{\phi} \lr{ – \PD{x^\nu}{\phi} } + {\delta^\mu}_\nu \LL,
\end{equation}
where \( \nu \) are labels which coordinates are translated:
\begin{equation}\label{eqn:qftLecture7:500}
\begin{aligned}
\partial_\nu \phi &= – \partial_\nu \phi \\
\partial_\nu \LL &= – \partial_\nu \LL.
\end{aligned}
\end{equation}
We call the conserved quantities elements of the energy-momentum tensor, and write it as
\begin{equation}\label{eqn:qftLecture7:520}
\boxed{
{T^\mu}_\nu = -\PD{x_\mu}{\phi} \PD{x^\nu}{\phi} + {\delta^\mu}_\nu \LL.
}
\end{equation}

Incidentally, we picked a non-standard sign convention for the tensor, as an explicit expansion of \( T^{00} \), the energy density component, shows
\begin{equation}\label{eqn:qftLecture7:540}
\begin{aligned}
{T^0}_0
&=
-\PD{t}{\phi}
\PD{t}{\phi}
+\inv{2}
\PD{t}{\phi}
\PD{t}{\phi}
– \inv{2} (\spacegrad \phi) \cdot (\spacegrad \phi)
– \frac{m^2}{2} \phi^2 – V(\phi) \\
&=
-\inv{2} \PD{t}{\phi} \PD{t}{\phi}
– \inv{2} (\spacegrad \phi) \cdot (\spacegrad \phi)
– \frac{m^2}{2} \phi^2 – V(\phi).
\end{aligned}
\end{equation}
Had we translated by \( -a^\mu \) we’d have a positive definite tensor instead.

Complex Klein-Gordon field: dead ends take III (or more?)

September 29, 2018 phy2403

Working on a problem from Peskin and Schroeder (and Zee too actually), I’ve hit dead ends in many different ways. The last dead end looks impressive just sitting there on the table where I abandoned it:

… however, after getting results that weren’t expected a number of different ways, I think I finally understand the fundamental assumption that I had wrong.  Take >= IV.

The identity ideologues are pushing hard from york region primary schools

September 28, 2018 Incoherent ramblings , ,

York region district school board is preparing to roll out their “Every Student Counts Survey” for primary grades (to be answered with parental assistance) or by oneself in grade 7-12 that’s packed full of the extreme identity politics that is discussed so widely in podcasts these days.

Here’s a link to a sample copy of the survey for kindergarden to grade 6, and one for higher grades.  The one for the little kids is particularly absurd, asking the kids or parents to pick from gender identities of male, female or one of the following 7 additional options:

  • Gender Fluid (Of, relating to, or being a person whose gender identity or expression changes or shifts along thegender spectrum.)
  • Gender Nonconforming (Not being in line with the cultural associations made in a given society about a person’s
    sex assigned at birth.)
  • Non‐Binary (Refers to a person whose gender identity does not align with the binary concept of gender such as
    man or woman.)
  • Questioning (Refers to a person who is unsure about their own gender identity.)
  • Transgender (Refers to a person whose gender identity differs from the one associated with their birth‐assigned
    sex.)
  • Two‐Spirit (An Indigenous person whose gender identity, spiritual identity or sexual orientation includes
    masculine, feminine or non‐binary spirits.)
  • A gender identity not listed above (please specify):…

as constrained by the footnote: “A person’s internal and deeply felt sense of being a man, a woman, both, neither, or having another identity on the gender spectrum. A person’s gender identity may be different from the sex assigned at birth (for example, female, intersex, male).”

These are questions being asked of kids that are potentially still many years away from puberty!  Why on earth is there such pressure to have the kids pick from or even try to understand these host of made up categories?

When I was in grade 7 I got teased about being gay because I was too shy to ask a girl on a date (or accept a date, in one instance).  Heck, I was a virgin until I was in my early twenties.  If this level of gender propaganda was being pushed when I was a kid, I’d have to have started wearing a lipstick and blouses just to fit it.  All because I was introverted and shy.  My sexual identity had nothing to do with these plethora of current gender categories, but just because I hadn’t clued in that you had to communicate to the opposite sex if you wanted to make any progress towards sexual goals.  Time is required to figure this stuff out, and having to choose prematurely, seems, to be blunt, completely stupid.

There’s some other stuff in this survey that is just bizarre.  The desire to label is so severe that it appears they are also making up new races:

Latino/Latina/Latinx

Googling Latinx, which I hadn’t heard of, and isn’t defined in any of the “helpful” footnotes, it appears that they are pushing gender politics into race too.  Wikipedia says of this: “Latinx (la-teen-ex) is a gender-neutral term sometimes used in lieu of Latino or Latina”.

Here’s some samples of the gobbledegook that this survey is packed full of:

  • People can be treated differently based on their religion, or perceived religion, which can lead to negative impacts and unequal outcomes. Islamophobia and antisemitism are examples of the way religion can be racialized. People can experience racism not only based on skin colour but also other perceived characteristics that are associated with religion.
  • Bullying is an ongoing misuse of power in relationships through repeated verbal, physical and/or social behaviour that causes physical and/or psychological harm. It can involve an individual or a group misusing their power over one or more persons. Bullying can happen in person or online, and it can be obvious (overt) or hidden (covert).
  • Discrimination is being treated negatively because of your gender, racial background, ethnic origin, religion, socioeconomic background, special education needs, sexual orientation, or other factors. Discrimination can be intentional or unintentional.
  • Harassment is engaging in a course of vexatious [annoying or provoking] comment or conduct which is known or
    ought reasonably to be known to be unwelcome.
  • Race is a social construct that groups people on the basis of perceived common ancestry and characteristics and affects how some people are perceived and treated. Race is often confused with ethnicity (a group of people who share a particular cultural heritage or background); there may be several ethnic groups within a racialized group.

I wonder how much money York region is paying to roll out and process this survey?  As well as injecting chaos and insanity into the school system in liew of actual content, it seems like a pointless waste of time and money that will provide little useful information.

If you want to make progress in education, how about stripping out some of the crap.  There’s no shortage of that.  Let’s not confuse kids with four different pictoral multiplication algorithms, so that they’ll give up and end up using calculators.  How about not injecting terminology into math education like “commutative” in grade 5 when you won’t see non-commutative (i.e. matrix) multiplication until university (since linear algebra seems to have been dropped from the high school curriculum for all intents and purposes).  Arg!

PHY2403H Quantum Field Theory. Lecture 6: Canonical quantization, Simple Harmonic Oscillators, Symmetries. Taught by Prof. Erich Poppitz

September 26, 2018 phy2403 , , ,

[Click here for a PDF of this post with nicer formatting]

DISCLAIMER: Very rough notes from class, with some additional side notes (QM SHO review, …).

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

Quantization of Field Theory

We are engaging in the “canonical” or Hamiltonian method of quantization. It is also possible to quantize using path integrals, but it is hard to prove that operators are unitary doing so. In fact, the mechanism used to show unitarity from path integrals is often to find the Lagrangian and show that there is a Hilbert space (i.e. using canonical quantization). Canonical quantization essentially demands that the fields obey a commutator relation of the following form
\begin{equation}\label{eqn:qftLecture6:20}
\antisymmetric{\pi(\Bx, t)}{\phi(\By, t)} = -i \delta^3(\Bx – \By).
\end{equation}
We assumed that the quantized fields obey the Hamiltonian relations
\begin{equation}\label{eqn:qftLecture6:160}
\begin{aligned}
\ddt{\phi} &= i \antisymmetric{H}{\phi} \\
\ddt{\pi} &= i \antisymmetric{H}{\pi}.
\end{aligned}
\end{equation}

We were working with the Hamiltonian density
\begin{equation}\label{eqn:qftLecture6:40}
\mathcal{H} =
\inv{2} (\pi(\Bx, t))^2
+
\inv{2} (\spacegrad \phi(\Bx, t))^2
+
\frac{m^2}{2} \phi^2
+
\frac{\lambda}{4} \phi^4,
\end{equation}
which included a mass term \( m \) and a potential term (\(\lambda\)). We will expand all quantities in Taylor series in \( \lambda \) assuming they have a structure such as
\begin{equation}\label{eqn:qftLecture6:180}
\begin{aligned}
f(\lambda) =
c_0 \lambda^0
+ c_1 \lambda^1
+ c_2 \lambda^2
+ c_3 \lambda^3
+ \cdots
\end{aligned}
\end{equation}
We will stop this \underline{perturbation theory} approach at \( O(\lambda^2) \), and will ignore functions such as \( e^{-1/\lambda} \).

Within perturbation theory, to leaving order, set \( \lambda = 0 \), so that \( \phi \) obeys the Klein-Gordon equation (if \( m = 0 \) we have just a d’Lambertian (wave equation)).

We can write our field as a Fourier transform
\begin{equation}\label{eqn:qftLecture6:60}
\phi(\Bx, t) = \int \frac{d^3 p}{(2\pi)^3} e^{i \Bp \cdot \Bx} \tilde{\phi}(\Bp, t),
\end{equation}
and due to a Hermitian assumption (i.e. real field) this implies
\begin{equation}\label{eqn:qftLecture6:80}
\tilde{\phi}^\conj(\Bp, t) = \tilde{\phi}(-\Bp, t).
\end{equation}

We found that the Klein-Gordon equation implied that the momentum space representation obey Harmonic oscillator equations
\begin{equation}\label{eqn:qftLecture6:100}
\begin{aligned}
\ddot{\tilde{\phi}}(\Bp, t) &= – \omega_\Bp \tilde{\phi}(\Bp, t) \\
\omega_\Bp &= \sqrt{\Bp^2 + m^2}.
\end{aligned}
\end{equation}
We may represent the solution to this equation as
\begin{equation}\label{eqn:qftLecture6:120}
\tilde{\phi}(\Bq, t) = \inv{\sqrt{2 \omega_\Bq}} \lr{
e^{-i \omega_\Bq t} a_\Bq
+
e^{i \omega_\Bq t} b_\Bq^\conj
}.
\end{equation}
This is a general solution, but imposing \( a_\Bq = b_{-\Bq} \) ensures \ref{eqn:qftLecture6:80} is satisfied.
This leaves us with
\begin{equation}\label{eqn:qftLecture6:140}
\tilde{\phi}(\Bq, t) = \inv{\sqrt{2 \omega_\Bq}} \lr{
e^{-i \omega_\Bq t} a_\Bq
+
e^{i \omega_\Bq t} a_{-\Bq}^\conj
}.
\end{equation}
We want to show that iff
\begin{equation}\label{eqn:qftLecture6:200}
\antisymmetric{a_\Bq}{a^\dagger_\Bp} = \lr{ 2 \pi }^3 \delta^3(\Bp – \Bq),
\end{equation}
then
\begin{equation}\label{eqn:qftLecture6:220}
\antisymmetric{\pi(\By, t)}{\phi(\Bx, t)} = -i \delta^3(\Bx – \By)
\end{equation}
where everything else commutes (i.e. \(
\antisymmetric{a_\Bp}{a_\Bq} =
\antisymmetric{a_\Bp^\dagger}{a_\Bq^\dagger} = 0 \)).
We will only show one direction, but you can go the other way too.

\begin{equation}\label{eqn:qftLecture6:240}
\phi(\Bx, t)
=
\int \frac{d^3 p}{(2\pi)^3 \sqrt{2 \omega_\Bp}} e^{i \Bp \cdot \Bx}
\lr{
e^{-i \omega_\Bp t} a_\Bp
+
e^{i \omega_\Bp t} a_{-\Bp}^\dagger
}
\end{equation}
\begin{equation}\label{eqn:qftLecture6:260}
\pi(\Bx, t) = \dot{\phi}
=
i \int \frac{d^3 q}{(2\pi)^3 \sqrt{2 \omega_\Bq}} \omega_\Bq e^{i \Bq \cdot \Bx}
\lr{
-e^{-i \omega_\Bq t} a_\Bq
+
e^{i \omega_\Bq t} a_{-\Bq}^\dagger
}
\end{equation}
The commutator is
\begin{equation}\label{eqn:qftLecture6:280}
\begin{aligned}
\antisymmetric{\pi(\By, t)}{\phi(\Bx, t)}
&=
i \int \frac{d^3 p}{(2\pi)^3
\sqrt{2 \omega_\Bp}}
\frac{d^3 q}{(2\pi)^3 \sqrt{2 \omega_\Bq}}
\omega_\Bq
e^{i \Bp \cdot \By + i \Bq \cdot \Bx}
\antisymmetric
{
-e^{-i \omega_\Bq t} a_\Bq
+
e^{i \omega_\Bq t} a_{-\Bq}^\dagger
}
{
e^{-i \omega_\Bp t} a_\Bp
+
e^{i \omega_\Bp t} a_{-\Bp}^\dagger
} \\
&=
i \int \frac{d^3 p}{(2\pi)^3
\sqrt{2 \omega_\Bp}}
\frac{d^3 q}{(2\pi)^3 \sqrt{2 \omega_\Bq}}
\omega_\Bq
e^{i \Bp \cdot \By + i \Bq \cdot \Bx}
\lr{
– e^{i (\omega_\Bp -\omega_\Bq) t}
\antisymmetric { a_\Bq } { a_{-\Bp}^\dagger }
+
e^{i (\omega_\Bq -\omega_\Bp) t}
\antisymmetric{a_{-\Bq}^\dagger}{ a_\Bp }
} \\
&=
i \int \frac{d^3 p}{(2\pi)^3
\sqrt{2 \omega_\Bp}}
\frac{d^3 q}{(2\pi)^3 \sqrt{2 \omega_\Bq}}
\omega_\Bq (2\pi)^3
e^{i \Bp \cdot \By + i \Bq \cdot \Bx}
\lr{
– e^{i (\omega_\Bp -\omega_\Bq) t} \delta^3(\Bq + \Bp)
– e^{i (\omega_\Bq -\omega_\Bp) t} \delta^3(-\Bq -\Bp)
} \\
&=
– 2 i \int \frac{d^3 p}{(2\pi)^3
2 \omega_\Bp}
\omega_\Bp
e^{i \Bp \cdot (\By – \Bx)} \\
&=
-i \delta^3(\By – \Bx),
\end{aligned}
\end{equation}
which is what we wanted to prove.

Free Hamiltonian

We call the \( \lambda = 0 \) case the “free” Hamiltonian.

\begin{equation}\label{eqn:qftLecture6:300}
\begin{aligned}
H
&= \int d^3 x \lr{ \inv{2} \pi^2 + \inv{2} (\spacegrad \phi)^2 + \frac{m^2}{2} \phi^2 } \\
&=
\inv{2} \int d^3 x
\frac{d^3 p}{(2\pi)^3}
\frac{d^3 q}{(2\pi)^3}
\frac{
e^{i (\Bp + \Bq)\cdot \Bx}
}{\sqrt{2 \omega_\Bp}
\sqrt{2 \omega_\Bq}}
\lr{
–
(\omega_\Bp)
(\omega_\Bq)
\lr{
-e^{-i \omega_\Bp t} a_\Bp
+
e^{i \omega_\Bp t} a_{-\Bp}^\dagger
}
\lr{
-e^{-i \omega_\Bq t} a_\Bq
+
e^{i \omega_\Bq t} a_{-\Bq}^\dagger
}
}
–
\Bp \cdot \Bq
\lr{
e^{-i \omega_\Bp t} a_\Bp
+
e^{i \omega_\Bp t} a_{-\Bp}^\dagger
}
\lr{
e^{-i \omega_\Bq t} a_\Bq
+
e^{i \omega_\Bq t} a_{-\Bq}^\dagger
}
+
m^2
\lr{
e^{-i \omega_\Bp t} a_\Bp
+
e^{i \omega_\Bp t} a_{-\Bp}^\dagger
}
\lr{
e^{-i \omega_\Bq t} a_\Bq
+
e^{i \omega_\Bq t} a_{-\Bq}^\dagger
}.
\end{aligned}
\end{equation}
An immediate simplification is possible by identifying a delta function factor \( \int d^3 x e^{i(\Bp + \Bq) \cdot \Bx}/(2\pi)^3 = \delta^3(\Bp + \Bq) \), so
\begin{equation}\label{eqn:qftLecture6:1060}
\begin{aligned}
H
&=
\inv{2}
\int
\frac{d^3 p}{(2\pi)^3}
\frac{1
}{2 \omega_\Bp}
\lr{
–
(\omega_\Bp)^2
\lr{
-e^{-i \omega_\Bp t} a_\Bp
+
e^{i \omega_\Bp t} a_{-\Bp}^\dagger
}
\lr{
-e^{-i \omega_\Bp t} a_{-\Bp}
+
e^{i \omega_\Bp t} a_{\Bp}^\dagger
}
}
+
(\Bp^2 + m^2)
\lr{
e^{-i \omega_\Bp t} a_\Bp
+
e^{i \omega_\Bp t} a_{-\Bp}^\dagger
}
\lr{
e^{-i \omega_\Bp t} a_{-\Bp}
+
e^{i \omega_\Bp t} a_{\Bp}^\dagger
} \\
&=
\inv{2} \int \frac{d^3 p}{(2 \pi)^3} \inv{2 \omega_\Bp}
\lr{
a_\Bp a_{-\Bp}
\lr{
{-\omega_\Bp^2 e^{-2 i \omega_\Bp t}}
+
{\omega_\Bp^2 e^{-2 i \omega_\Bp t}}
}
+
a_{-\Bp}^\dagger a_{\Bp}^\dagger
\lr{
-{\omega_\Bp^2 e^{2 i \omega_\Bp t}}
+
{\omega_\Bp^2 e^{2 i \omega_\Bp t}}
}
+
a_\Bp a^\dagger_{\Bp} \omega^2_\Bp(1 + 1)
+
a^\dagger_{-\Bp} a_{-\Bp} \omega^2_\Bp(1 + 1)
}
\end{aligned}
\end{equation}
When all is said and done we are left with
\begin{equation}\label{eqn:qftLecture6:320}
H =
\int \frac{d^3 p}{(2 \pi)^3} \frac{\omega_\Bp}{2} \lr{
a^\dagger_{-\Bp}
a_{-\Bp}
+
a_{\Bp}
a^\dagger_{\Bp}
}
\end{equation}
and finally with a \( \Bp \rightarrow -\Bp \) transformation (\( \iiint_{-R}^R d^3 p \rightarrow (-1)^3 \iiint_R^{-R} d^3 p’ = (-1)^6 \iiint_{-R}^R d^3 p’ \)) in the first integral our free Hamiltonian (\( \lambda = 0 \)) is
\begin{equation}\label{eqn:qftLecture6:340}
\boxed{
H_0 =
\int \frac{d^3 p}{(2 \pi)^3} \frac{\omega_\Bp}{2} \lr{
a^\dagger_{\Bp}
a_{\Bp}
+
a_{\Bp}
a^\dagger_{\Bp}
}
}
\end{equation}

From the commutator relationship \ref{eqn:qftLecture6:200} we can write
\begin{equation}\label{eqn:qftLecture6:360}
a_\Bp a^\dagger_\Bq
=
a^\dagger_\Bq
a_\Bp
+ (2 \pi)^3 \delta^3(\Bp – \Bq),
\end{equation}
so

\begin{equation}\label{eqn:qftLecture6:380}
H_0 =
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp
\lr{
a^\dagger_{\Bp}
a_{\Bp}
+
\inv{2} (2 \pi)^3 \delta^3(0)
}
\end{equation}
The delta function term can be interpreted using
\begin{equation}\label{eqn:qftLecture6:400}
(2 \pi)^3 \delta^3(\Bq)
= \int d^3 x e^{i \Bq \cdot \Bx},
\end{equation}
so when \( \Bq = 0 \)
\begin{equation}\label{eqn:qftLecture6:420}
(2 \pi)^3 \delta^3(0) = \int d^3 x = V.
\end{equation}

We can write the Hamiltonian now in terms of the volume
\begin{equation}\label{eqn:qftLecture6:440}
\boxed{
H_0 =
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp
a^\dagger_{\Bp}
a_{\Bp}
+ V_3
\int \frac{d^3 p}{(2 \pi)^3} \frac{\omega_\Bp }{2} \times 1
}
\end{equation}

QM SHO review

In units with \( m = 1 \) the non-relativistic QM SHO has the Hamiltonian
\begin{equation}\label{eqn:qftLecture6:460}
H
= \inv{2} p^2 + \frac{\omega^2}{2} q^2.
\end{equation}

If we define a position operator with a time-domain Fourier representation given by
\begin{equation}\label{eqn:qftLecture6:480}
q = \inv{\sqrt{2\omega}} \lr{ a e^{-i \omega t} + a^\dagger e^{i \omega t} },
\end{equation}
where the Fourier coefficients \( a, a^\dagger \) are operator valued, then the momentum operator is
\begin{equation}\label{eqn:qftLecture6:500}
p = \dot{q} =
\frac{i\omega}{\sqrt{2\omega}} \lr{ -a e^{-i \omega t} + a^\dagger e^{i \omega t} },
\end{equation}
or inverting for \( a, a^\dagger \)
\begin{equation}\label{eqn:qftLecture6:1080}
\begin{aligned}
a &= \sqrt{\frac{\omega}{2}} \lr{ q – \inv{i \omega} p } e^{-i \omega t} \\
a^\dagger &= \sqrt{\frac{\omega}{2}} \lr{ q + \inv{i \omega} p } e^{i \omega t}.
\end{aligned}
\end{equation}
By inspection it is apparent that the product \( a^\dagger a \) will be related to the Hamiltonian (i.e. a difference of squares). That product is
\begin{equation}\label{eqn:qftLecture6:1120}
\begin{aligned}
a^\dagger a
&=
\frac{\omega}{2}
\lr{ q + \inv{i \omega} p }
\lr{ q – \inv{i \omega} p } \\
&=
\frac{\omega}{2} \lr{ q^2 + \inv{\omega^2} p^2 – \inv{i \omega} \antisymmetric{q}{p} } \\
&= \inv{2 \omega} \lr{ p^2 + \omega^2 q^2 – \omega },
\end{aligned}
\end{equation}
or
\begin{equation}\label{eqn:qftLecture6:1140}
H = \omega \lr{ a^\dagger a + \inv{2} }.
\end{equation}
We can glean some of the properties of \( a, a^\dagger \) by computing the commutator of \( p, q \), since that has a well known value
\begin{equation}\label{eqn:qftLecture6:520}
\begin{aligned}
i
&= \antisymmetric{q}{p} \\
&=
\frac{i\omega}{2 \omega} \antisymmetric
{ a e^{-i \omega t} + a^\dagger e^{i \omega t} }
{ -a e^{-i \omega t} + a^\dagger e^{i \omega t} } \\
&=
\frac{i}{2} \lr{
\antisymmetric{a}{a^\dagger} –
\antisymmetric{a^\dagger}{a} } \\
&=
i
\antisymmetric{a}{a^\dagger},
\end{aligned}
\end{equation}
so
\begin{equation}\label{eqn:qftLecture6:1100}
\antisymmetric{a}{a^\dagger} = 1.
\end{equation}
The operator \( a^\dagger a \) is the workhorse of the Hamiltonian and worth studying independently. In particular, assume that we have a set of states \( \ket{n} \) that are eigenstates of \( a^\dagger a \) with eigenvalues \( \lambda_n \), that is
\begin{equation}\label{eqn:qftLecture6:1160}
a^\dagger a \ket{n} = \lambda_n \ket{n}.
\end{equation}
The action of \( a^\dagger a \) on \( a^\dagger \ket{n} \) is easy to compute
\begin{equation}\label{eqn:qftLecture6:1180}
\begin{aligned}
a^\dagger a a^\dagger \ket{n}
&=
a^\dagger \lr{ a^\dagger a + 1 } \ket{n} \\
&=
\lr{ \lambda_n + 1 } a^\dagger \ket{n},
\end{aligned}
\end{equation}
so \( \lambda_n + 1 \) is an eigenvalue of \( a^\dagger \ket{n} \). The state \( a^\dagger \ket{n} \) has an energy eigenstate that is one unit of energy larger than \( \ket{n} \). For this reason we called \( a^\dagger \) the raising (or creation) operator.
Similarly,
\begin{equation}\label{eqn:qftLecture6:1200}
\begin{aligned}
a^\dagger a a \ket{n}
&=
\lr{ a a^\dagger – 1 } a \ket{n} \\
&=
(\lambda_n – 1) a \ket{n},
\end{aligned}
\end{equation}
so \( \lambda_n – 1 \) is the energy eigenvalue of \( a \ket{n} \), having one less unit of energy than \( \ket{n} \).
We call \( a \) the annihilation (or lowering) operator.
If we argue that there is a lowest energy state, perhaps designated as \( \ket{0} \) then we must have
\begin{equation}\label{eqn:qftLecture6:560}
a\ket{0} = 0,
\end{equation}
by the assumption that there are no energy eigenstates with less energy than \( \ket{0} \).
We can think of higher order states being constructed from the ground state from using the raising operator \( a^\dagger \)
\begin{equation}\label{eqn:qftLecture6:580}
\ket{n} = \frac{(a^\dagger)^n}{\sqrt{n!}} \ket{0}
\end{equation}

Discussion

We’ve diagonalized in the Fourier representation for the momentum space fields. For every value of momentum \( \Bp \) we have a quantum SHO.

For our field space we call our space the Fock vacuum and
\begin{equation}\label{eqn:qftLecture6:620}
a_\Bp\ket{0} = 0,
\end{equation}
and call \( a_\Bp \) the “annihilation operator”, and
call \( a^\dagger_\Bp \) the “creation operator”.
We say that \( a^\dagger_\Bp \ket{0} \) is the creation of a state of a single particle of momentum \( \Bp \) by \( a^\dagger_\Bp \).

We are discarding the volume term, a procedure called “normal ordering”. We define
\begin{equation}\label{eqn:qftLecture6:640}
\frac{a^\dagger a + a a^\dagger}{2}
:\equiv
a^\dagger a
\end{equation}
We are essentially forgetting the vacuum energy as some sort of unobservable quantity, leaving us with the free Hamiltonian of
\begin{equation}\label{eqn:qftLecture6:660}
H_0 =
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp
a^\dagger_{\Bp}
a_{\Bp}
\end{equation}
Consider
\begin{equation}\label{eqn:qftLecture6:680}
\begin{aligned}
H_0
a^\dagger_\Bq \ket{0}
&=
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp
a^\dagger_{\Bp}
a_{\Bp}
a^\dagger_\Bq
\ket{0} \\
&=
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp
a^\dagger_{\Bp}
\lr{
a^\dagger_\Bq a_\Bp + (2 \pi)^3 \delta^3(\Bp – \Bq)
}
\ket{0} \\
&=
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp
a^\dagger_{\Bp} \lr{
a^\dagger_\Bq {a_\Bp \ket{0}}
+ (2 \pi)^3 \delta^3(\Bp – \Bq) \ket{0}
} \\
&=
\omega_\Bq a^\dagger_\Bq \ket{0}.
\end{aligned}
\end{equation}

Question:

Is it possible to modify the Lagrangian or Hamiltonian that we start with so that this vacuum ground state is eliminated? Answer: Only by imposing super-symmetric constraints (that pairs this (Bosonic) Hamiltonian to a Fermonic system in a way that there is exact cancellation).

We will see that the momentum operator has the form
\begin{equation}\label{eqn:qftLecture6:700}
\mathcal{P}
=
\int \frac{d^3 p}{(2 \pi)^3} \Bp a^\dagger_\Bp a_\Bp.
\end{equation}
We say that \( a^\dagger_\Bp a^\dagger_\Bq \ket{0} \) a two particle space with energy \( \omega_\Bp + \omega_q\), and \(
(a^\dagger_\Bp)^m (a^\dagger_\Bq)^n \ket{0} \equiv
(a^\dagger_\Bp)^m \ket{0} \otimes (a^\dagger_\Bq)^n \ket{0} \), a \( m + n \) particle space.

There is a connection to statistical mechanics that is of interest

\begin{equation}\label{eqn:qftLecture6:720}
\begin{aligned}
\expectation{E}
&= \inv{Z} \sum_n E_n e^{-E_n/\kB T} \\
&= \inv{Z} \sum_n \bra{n} e^{-\hat{H}/\kB T} \hat{H} \ket{n},
\end{aligned}
\end{equation}
so for a SHO Hamiltonian system
\begin{equation}\label{eqn:qftLecture6:740}
\begin{aligned}
\expectation{E}
&= \inv{Z} \sum_n e^{-E_n/\kB T} \bra{n} \hat{H} \ket{n} \\
&= \inv{Z} \sum_n e^{-E_n/\kB T} \bra{n} \omega a^\dagger a \ket{n} \\
&= \frac{\omega}{e^{\omega/\kB T} – 1 } \\ \\
&= \expectation{ \omega a^\dagger a }_{\kB T}
\end{aligned}
\end{equation}
the \( \kB T \) ensemble average energy for a SHO system. Note that this sum was evaluated by noting that \( \bra{n} a^\dagger a \ket{n} = n \) which leaves sums of the form
\begin{equation}\label{eqn:qftLecture6:1220}
\begin{aligned}
\frac{\sum_{n = 0}^\infty n a^n }{\sum_{n = 0}^\infty a^n}
&=
a \frac{\sum_{n = 1}^\infty n a^{n-1} }{\sum_{n = 0}^\infty a^n} \\
&=
a (1 – a) \frac{d}{da} \lr{ \inv{1 – a} } \\
&=
\frac{a}{1 – a}.
\end{aligned}
\end{equation}

If we consider a real scalar field of mass \( m \) we have \( \omega_\Bp = \sqrt{ \Bp^2 + m^2 } \), but for a Maxwell field \( \BE, \BB \) where \( m = 0 \), our dispersion relation is \( \omega_\Bp = \Abs{\Bp} \).

We will see that for a free Maxwell field (no charges or currents) the Hamiltonian is
\begin{equation}\label{eqn:qftLecture6:760}
H_{\text{Maxwell}} =
\sum_{i = 1}^2
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp {a^i}^\dagger_\Bp {a^i}_\Bp,
\end{equation}
where \( i \) is a polarization index.

We expect that we can evaluate an average such as \ref{eqn:qftLecture6:740} for our field, and operate using the analogy

\begin{equation}\label{eqn:qftLecture6:780}
\begin{aligned}
a a^\dagger &= a^\dagger a + 1 \\
a_\Bp a_\Bp^\dagger &= a_\Bp^\dagger a_\Bp + V_3.
\end{aligned}
\end{equation}
so if we rescale by \( \sqrt{V_3} \)
\begin{equation}\label{eqn:qftLecture6:800}
a_\Bp = \sqrt{V_3} \tilde{a}_\Bp
\end{equation}
Then we have commutator relations like standard QM
\begin{equation}\label{eqn:qftLecture6:820}
\tilde{a} \tilde{a}^\dagger = \tilde{a}^\dagger \tilde{a} + 1.
\end{equation}

So we can immediately evaluate the energy expectation for our quantized fields
\begin{equation}\label{eqn:qftLecture6:840}
\begin{aligned}
\expectation{H_0}
&=
\expectation{
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp a_\Bp^\dagger a_\Bp
} \\
&=
\int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp V_3 \expectation{ \tilde{a}^\dagger_\Bp a_\Bp } \\
&=
V_3
\int \frac{d^3 p}{(2 \pi)^3} \frac{\omega_\Bp}{e^{\omega_\Bp/\kB T} – 1}.
\end{aligned}
\end{equation}
Using this with the Maxwell field, we have a factor of two from polarization
\begin{equation}\label{eqn:qftLecture6:860}
U^{\text{Maxwell}} = 2 V_3
\int \frac{d^3 p}{(2 \pi)^3} \frac{\Abs{\Bp}}{e^{\omega_\Bp/\kB T} – 1},
\end{equation}
which is Planck’s law describing the blackbody energy spectrum.

Switching gears: Symmetries.

The question is how to apply the CCR results to moving frames, which is done using Lorentz transformations. Just like we know that the exponential of the Hamiltonian (times time) represents time translations, we will examine symmetries that relate results in different frames.

Examples.

For scalar field(s) with action
\begin{equation}\label{eqn:qftLecture6:880}
S = \int d^d x \LL(\phi^i, \partial_\mu \phi^i).
\end{equation}
For example, we’ve been using our massive (Boson) real scalar field with Lagrangian density
\begin{equation}\label{eqn:qftLecture6:900}
\LL = \inv{2} \partial_\mu \phi\partial^\mu \phi – \frac{m^2}{2} \phi^2 – V(\phi).
\end{equation}

Internal symmetry example

\begin{equation}\label{eqn:qftLecture6:920}
H = J \sum_{\expectation{n, n’}} \BS_n \cdot \BS_{n’},
\end{equation}
where the sum means the sum over neighbouring indexes \( n, n’ \) as sketched in

fig. 1. Neighbouring spin cells.

Such a Hamiltonian is left invariant by the transformation \( \BS_n \rightarrow -\BS_n \) since the Hamiltonian is quadratic.

Suppose that \( \phi \rightarrow -\phi\) is a symmetry (it leaves the Lagrangian unchanged). Example

\begin{equation}\label{eqn:qftLecture6:940}
\phi =
\begin{bmatrix}
\phi^1 \\
\phi^2 \\
\vdots
\phi^n \\
\end{bmatrix}
\end{equation}

the Lagrangian
\begin{equation}\label{eqn:qftLecture6:960}
\LL = \inv{2} \partial_\mu \phi^\T \partial^\mu \phi – \frac{m^2}{2} \phi^\T \phi – V(\phi^\T \phi).
\end{equation}
If \( O \) is any \( n \times n \) orthogonal matrix, then it is symmetry since
\begin{equation}\label{eqn:qftLecture6:980}
\phi^\T \phi \rightarrow \phi^\T O^\T O \phi = \phi^\T \phi.
\end{equation}

O(2) model, HW, problem 2. Example for complex \( \phi \)
\begin{equation}\label{eqn:qftLecture6:1000}
\phi \rightarrow e^{i \phi} \phi,
\end{equation}
\begin{equation}\label{eqn:qftLecture6:1020}
\phi = \frac{\psi_1 + i \psi_2}{\sqrt{2}}
\end{equation}

\begin{equation}\label{eqn:qftLecture6:1040}
\begin{bmatrix}
\psi_1 \\
\psi_2
\end{bmatrix}
\rightarrow
\begin{bmatrix}
\cos\alpha & \sin\alpha \\
-\sin\alpha & \cos\alpha
\end{bmatrix}
\begin{bmatrix}
\psi_1 \\
\psi_2
\end{bmatrix}
\end{equation}