phy2403

PHY2403H Quantum Field Theory. Lecture 11: Momentum matrix elements, spacelike surfaces, microcausality, Lorentz invariant measure, wave function Green’s function, retarded time contour, advanced time contour. Taught by Prof. Erich Poppitz

October 20, 2018 phy2403 , , , , , ,

[Click here for a PDF of this post with nicer formatting]

DISCLAIMER: Very rough notes from class, with some additional side notes.

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

Relativistic normalization.

We will continue looking at the generator of spacetime translation \( \hatU(\Lambda) \), which has the property
\begin{equation}\label{eqn:qftLecture11:40}
\hatU(\Lambda) \ket{0} = \ket{0},
\end{equation}

That is
\begin{equation}\label{eqn:qftLecture11:760}
\hatU(\Lambda) = \mathbf{1} + \text{operators that anhillate the vacuum state}.
\end{equation}

The action on a field was
\begin{equation}\label{eqn:qftLecture11:60}
\hatU(\Lambda)
\phihat(x) \hatU^\dagger(\Lambda)
= \phihat(\Lambda x),
\end{equation}
and the action on the anhillation operator was
\begin{equation}\label{eqn:qftLecture11:300}
\hatU(\Lambda)
\sqrt{ 2 \omega_\Bp } \hat{a}_\Bp
\hatU^\dagger(\Lambda)
=
\sqrt{ 2 \omega_{\Lambda \Bp} } \hat{a}_{\Lambda \Bp}.
\end{equation}

If \( \ket{\Bp_1} \) is the one particle state with momentum \( \Bp_1 \), then that momentum state can be generated from the ground state with the following normalized creation operation
\begin{equation}\label{eqn:qftLecture11:780}
\ket{\Bp_1} = \sqrt{ 2 \omega_{\Bp_1} } \hat{a}_{\Bp_1}^\dagger \ket{0}.
\end{equation}

We can compute the matrix element between two matrix states using the creation operator representation
\begin{equation}\label{eqn:qftLecture11:80}
\begin{aligned}
\braket{\Bp_2}{\Bp_1}
&=
\sqrt{ 2 \omega_{\Bp_1} }
\sqrt{ 2 \omega_{\Bp_2} }
\bra{0}
\hat{a}_{\Bp_2}
\hat{a}_{\Bp_1}^\dagger
\ket{0} \\
&=
\sqrt{ 2 \omega_{\Bp_1} }
\sqrt{ 2 \omega_{\Bp_2} }
\bra{0}
\lr{
\hat{a}_{\Bp_1}^\dagger
\hat{a}_{\Bp_2}
+
i (2 \pi)^3 \delta^3(\Bp – \Bq)
} \\
&=
\sqrt{ 2 \omega_{\Bp_1} }
\sqrt{ 2 \omega_{\Bp_2} }
(2 \pi)^3 \delta^3(\Bp_1 – \Bp_2) \\
&=
2 \omega_{\Bp_1}
(2 \pi)^3 \delta^3(\Bp_1 – \Bp_2).
\end{aligned}
\end{equation}

Spacelike surfaces.

fig. 0. Constant spacelike surface.

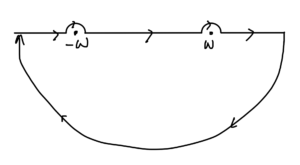
If \( x^\mu, p^\mu \) are four vectors, then \( p^\mu x_\mu = \text{invariant} = {p’}^\mu x’_\mu \). The light cone is the surface \( p_0^2 = \Bp^2 \), whereas timelike four-momentum form a parabaloid surface \( p_0^2 – \Bp^2 = m^2 \) (i.e. \( E = \sqrt{ m^2 c^4 + \Bp^2 c^2 } \)).
The surface for constant spacelike points (i.e. all related by a Lorentz transformation) is illustrated in fig. 0. A boost moves a point up or down that surface along the energy axis. It is therefore possible to use a sequence of boost and rotation to transform a point \( (E, \Bp) \rightarrow (-E, \Bp) \rightarrow (-E, -\Bp) \). That is, any spacelike four-vector \( x \) may be transformed to \( -x \) using a Lorentz transformation.

Condition on microcausality.

We defined operators \( \phihat(\Bx) \), which was a Hermitian operator for the real scalar field. For the complex scalar field we used \( \phihat(\Bx) = (\phihat_1 + \phihat_2)/\sqrt{2} \), where each of \( \phihat_1, \phihat_2 \) were Hermitian operators. i.e. we can think of these operators as “observables”, that is \( \phihat(\Bx) = \phihat^\dagger(\Bx) \).

We now want to show that these operators commute at spacelike separations, and see how this relates to the question of causality. In particular, we want to see that an observation of one operator, will not effect the measurement of the other.

The condition of microcausality is
\begin{equation*}
\antisymmetric{\phihat(x)}{\phihat(y)} = 0
\end{equation*}
if \( x \sim y \), that is \( (x – y)^2 < 0 \). That is, \( x, y \) are spacelike separated.

We wrote

\begin{equation}\label{eqn:qftLecture11:160}
\phihat(x)
=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{
e^{-i p \cdot x} }{p^0 = \omega_\Bp} \hat{a}_\Bp
+
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{
e^{i p \cdot x} }{p^0 = \omega_\Bp} \hat{a}^\dagger_\Bp
,
\end{equation}
or \( \phihat(x) = \phihat_{-}(x) + \phihat_{+}(x) \), where
\begin{equation}\label{eqn:qftLecture11:180}
\begin{aligned}
\phihat_{-}(x) &=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{
e^{-i p \cdot x} }{p^0 = \omega_\Bp} \hat{a}_\Bp \\
\phihat_{+}(x) &=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{
e^{i p \cdot x} }{p^0 = \omega_\Bp} \hat{a}^\dagger_\Bp
\end{aligned}
\end{equation}

Compute the commutator
\begin{equation}\label{eqn:qftLecture11:200}
\begin{aligned}
D(x)
&= \antisymmetric{\phihat_{-}(x)}{\phihat_{+}(0)} \\
&=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{ e^{-i p \cdot x} }{p^0 = \omega_\Bp}
\int \frac{d^3 k}{(2 \pi)^3 \sqrt{2 \omega_\Bk}}
\evalbar{ e^{i k \cdot 0} }{k^0 = \omega_\Bk}
\antisymmetric{\hat{a}_\Bp }{\hat{a}_\Bk^\dagger } \\
&=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{ e^{-i p \cdot x} }{p^0 = \omega_\Bp}
\int \frac{d^3 k}{(2 \pi)^3 \sqrt{2 \omega_\Bk}}
(2 \pi)^3 \delta^3(\Bp – \Bk),
\end{aligned}
\end{equation}
\begin{equation}\label{eqn:qftLecture11:800}
\boxed{
D(x)
=
\int \frac{d^3 p}{(2 \pi)^3 2 \omega_\Bp}
\evalbar{ e^{-i p \cdot x} }{p^0 = \omega_\Bp}.
}
\end{equation}

Now about the commutator at two spacetime points
\begin{equation}\label{eqn:qftLecture11:220}
\begin{aligned}
\antisymmetric{\phihat(x)}{\phihat(y)}
&=
\antisymmetric{\phihat_{-}(x) + \phihat_{+}(x)}{\phihat_{-}(y) + \phihat_{+}(y)} \\
&=
\antisymmetric{\phihat_{-}(x)}{\phihat_{+}(y)}
+
\antisymmetric{\phihat_{+}(x)}{\phihat_{-}(y)} \\
&=
-D(y – x) + D(x – y)
\end{aligned}
\end{equation}

Find
\begin{equation}\label{eqn:qftLecture11:240}
\begin{aligned}
\antisymmetric{\phihat(x)}{\phihat(y)} &= D(x – y) – D(y – x) \\
\antisymmetric{\phihat(x)}{\phihat(0)} &= D(x) – D(- x)
\end{aligned}
\end{equation}

Let’s look at \( D(x) \), \ref{eqn:qftLecture11:800}, a bit more closely.

Claim:

D(x) is Lorentz invariant (has the same value for all \( x^\mu, {x’}^\mu \)

We can see this by writing this out as
\begin{equation}\label{eqn:qftLecture11:280}
D(x)
=
\int \frac{d^3 p}{(2 \pi)^3 } dp^0
\delta( p_0^2 – \Bp^2 – m^2) \Theta(p^0)
e^{-i p \cdot x}
\end{equation}

The exponential is Lorentz invariant, and the delta function has been put into a Lorentz invariant form.

Claim 1:

\( D(x) = D(x’) \) where \( x^2 = {x’}^2 \).

Claim 2:

\( x^\mu, -x^\mu \) are related by Lorentz transformations if \( x^2 < 0 \).

From the figure, we see that \( D(x) = D(-x) \) for a spacelike point, which implies that \( \antisymmetric{\phihat(x)}{\phihat(0)} = 0 \) for a spacelike point \( x \).

We’ve shown this for free fields, but later we will see that this is the case for interacting fields too.

Harmonic oscillator.

\begin{equation}\label{eqn:qftLecture11:320}
L = \inv{2} \qdot^2 – \frac{\omega^2}{t} q^2 – j(t) q
\end{equation}

The term \( j(t) \) shifts the origin in a time dependent fashion (graphical illustration in class wiggling a hockey stick, as a sample of a harmonic oscillator).

\begin{equation}\label{eqn:qftLecture11:340}
H = \frac{p^2}{2} + \frac{\omega^2}{t} q^2 + j(t) q
\end{equation}

\begin{equation}\label{eqn:qftLecture11:360}
\begin{aligned}
i \qdot_H(t) &= \antisymmetric{q_H}{H} = i p_H \\
i \pdot_H(t) &= \antisymmetric{p_H}{H} = -i \omega^2 q_H – i j(t)
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture11:380}
\ddot{q}_H(t) = – \omega^2 q_H(t) – j(t)
\end{equation}
or
\begin{equation}\label{eqn:qftLecture11:400}
(\partial_{tt} + \omega^2 ) q_H(t) = – j(t)
\end{equation}

\begin{equation}\label{eqn:qftLecture11:420}
q_H(t) = q_H^0( t ) +
\int G_R(t – t’) j(t’) dt’
\end{equation}

This solves the equation provided \( G_R(t – t’) \) has the property that
\begin{equation}\label{eqn:qftLecture11:440}
\boxed{
(\partial_{tt} + \omega^2)
G_R(t – t’)
= – \delta(t – t’)
}
\end{equation}

That is
\begin{equation}\label{eqn:qftLecture11:460}
(\partial_{tt} + \omega^2)
q_H(t) =
(\partial_{tt} + \omega^2)
q_H^0( t )
+
(\partial_{tt} + \omega^2)
\int G_R(t – t’) j(t’) dt’
\end{equation}

This function \( G_R \) is called the retarded Green’s function. We want to find this function, and as usual, we do this by taking the Fourier transform of \ref{eqn:qftLecture11:440}

\begin{equation}\label{eqn:qftLecture11:480}
\begin{aligned}
\int dt e^{i p t}
(\partial_{tt} + \omega^2) G_R(t – t’)
&=
-\int_{-\infty}^\infty dt e^{i p t}
\delta(t – t’) \\
&= – e^{i p t’}
\end{aligned}
\end{equation}

Let
\begin{equation}\label{eqn:qftLecture11:500}
G(t – t’) = \int \frac{dp }{2 \pi} e^{- i p'(t – t’)} \tilde{G}(p’),
\end{equation}
so

\begin{equation}\label{eqn:qftLecture11:520}
\begin{aligned}
– e^{i pt’}
&=
\int dt e^{i p t}
(\partial_{tt} + \omega^2)
\int \frac{dp’}{2 \pi} e^{- i p'(t – t’)} \tilde{G}(p’) \\
&=
\int dt e^{i p t} \int
\frac{dp’}{2 \pi} \lr{ -{p’}^2 + \omega^2 } e^{- i p'(t – t’)} \tilde{G}(p’) \\
&=
\int dp’ \lr{ -{p’}^2 + \omega^2 } e^{i p’ t’} \delta(p – p’) \tilde{G}(p’) \\
&=
\lr{ -{p}^2 + \omega^2 } \tilde{G}(p) e^{i p t’}
\end{aligned}
\end{equation}

so
\begin{equation}\label{eqn:qftLecture11:540}
\tilde{G}(p)
= \inv{p^2 – \omega^2}
\end{equation}

Now

\begin{equation}\label{eqn:qftLecture11:560}
G(t)
= \int \frac{dp}{2 \pi} e^{-i p t}
\tilde{G}(p)
\end{equation}

Let’s write the momentum space Green’s function as
\begin{equation}\label{eqn:qftLecture11:580}
\tilde{G}(p)
= \inv{(p – \omega)(p + \omega)}
\end{equation}

The solution contained
\begin{equation}\label{eqn:qftLecture11:600}
\int G(t – t’) j(t’) dt’.
\end{equation}
Suppose \( j(t) = 0 \) for all \( t < t_0 \). We want the effect of \( j(t) \) to be felt in the future, for example, \(j(t) \) is an impulse starting at some time. We want \( G(t) \) to vanish at negative times.

We want the integral
\begin{equation}\label{eqn:qftLecture11:620}
G(t)
= \int \frac{dp}{2 \pi} e^{-i p t}
\inv{(p – \omega)(p + \omega)}
\end{equation}
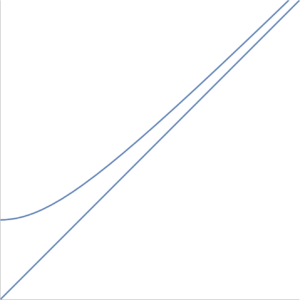
to vanish when \( t < 0 \). Start with \( t > 0 \) (that is \( t’ < t \)), so that \( e^{-i p t} = e^{-i p \Abs{t}} \) which means that we have to integrate over a lower plane contour like fig. 1, because the imaginary part of \( p \) is negative, but for \( t < 0 \) (that is \( t’ > t \)), we want an upper plane contour like fig. 2.

fig. 1. Lower plane contour.

 

fig. 2. Upper plane contour.

 

Question: since we are integrating over the real line, how can we get away with deforming the contour?
Answer: it works. If we do this we get a Green’s function that makes sense (better answer later?)

We add an infinite circle, so that we can integrate over a closed contour, and pick the contour so that it is zero for \( t < 0 \) and non-zero (enclosed poles) for \( t > 0 \).

\begin{equation}\label{eqn:qftLecture11:640}
\begin{aligned}
G_R(t > 0)
&= \int_C \frac{dp}{2 \pi} e^{-i p t}
\inv{(p – \omega)(p + \omega)} \\
&=
\inv{2 \pi} (-2 \pi i) \lr{
\frac{e^{-i \omega t}}{2 \omega}
–
\frac{e^{i \omega t}}{2 \omega}
} \\
&=
-\frac{\sin(\omega t)}{\omega}.
\end{aligned}
\end{equation}

Now we write the Green’s function for all time as
\begin{equation}\label{eqn:qftLecture11:660}
\boxed{
G_R(t) =
-\frac{\sin(\omega t)}{\omega} \Theta(t).
}
\end{equation}

The question of what contour to pick can now be justified by the result, since this satisfies fig. 3). In particular, the bumps up and down contour will be used to derive the “Feynman propagator” that we’ll use later.

fig. 3. All possible deformations around the poles.

 

Field theory (where we are going).

We will consider a massive real scalar field theory with an external source with action

\begin{equation}\label{eqn:qftLecture11:680}
S = \int d^4 x \lr{
\inv{2} \partial_\mu \phi \partial^\mu \phi – \frac{m^2}{2} \phi^2 + j(x) \phi(x)
}
\end{equation}

We don’t have examples of currents that create scalar fields, but to study such as system, recall that
in electromagnetism we added sources to the field by adding a term like
\begin{equation}\label{eqn:qftLecture11:700}
\int d^4 x A^\mu(x) j_\mu(x),
\end{equation}
to our action.

The equation of motion can be found to be
\begin{equation}\label{eqn:qftLecture11:720}
\lr{ \partial_\mu \partial^\mu + m^2 } \phi(x) = j(x).
\end{equation}

We want to study the Green’s function of this Klien-Gordon equation, defined to obey
\begin{equation}\label{eqn:qftLecture11:740}
\lr{ \partial_\mu \partial^\mu + m^2 }_x G(x – y) = -i \delta^4(x – y),
\end{equation}
where the \( -i \) factor is for convienience.
This is analogous to the Green’s function that we just studied for the QM harmonic oscillator.

Question: Compute \( D(x-y) \) from the commutator.

Generalize the derivation \ref{eqn:qftLecture11:800} by computing the commutator at two different space time points \( x, y \).

Answer

Let
\begin{equation}\label{eqn:qftLecture11:860}
\begin{aligned}
D(x – y)
&= \antisymmetric{\phihat_{-}(x)}{\phihat_{+}(y)} \\
&=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{ e^{-i p \cdot x} }{p^0 = \omega_\Bp}
\int \frac{d^3 k}{(2 \pi)^3 \sqrt{2 \omega_\Bk}}
\evalbar{ e^{i k \cdot y} }{k^0 = \omega_\Bk}
\antisymmetric{\hat{a}_\Bp }{\hat{a}_\Bk^\dagger } \\
&=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}}
\evalbar{ e^{-i p \cdot x} }{p^0 = \omega_\Bp}
\int \frac{d^3 k}{(2 \pi)^3 \sqrt{2 \omega_\Bk}}
\evalbar{ e^{i k \cdot y} }{k^0 = \omega_\Bk}
(2 \pi)^3 \delta^3(\Bp – \Bk) \\
&=
\int \frac{d^3 p}{(2 \pi)^3 2 \omega_\Bp}
\evalbar{ e^{-i p \cdot (x – y)} }{p^0 = \omega_\Bp}.
\end{aligned}
\end{equation}

Question: Verification of harmonic oscillator Green’s function.

Take the derivatives of a convolution of the Green’s function \ref{eqn:qftLecture11:660} to show that it satisifies \ref{eqn:qftLecture11:440}.

Answer

Let
\begin{equation}\label{eqn:qftLecture11:880}
\begin{aligned}
q(t)
&= \int_{-\infty}^\infty G(t – t’) j(t’) dt’ \\
&= -\inv{\omega} \int_{-\infty}^\infty \sin(\omega(t – t’)) \Theta(t – t’) j(t’) dt’.
\end{aligned}
\end{equation}
We are free to add any \( q_0(t) \) that satisfies the homogeneous wave equation \( q_0”(t) + \omega^2 q_0(t) = 0 \) to our assumed convolution solution \ref{eqn:qftLecture11:880}, but that isn’t interesting for this exersize.
Since \( \Theta(t – t’) = 0 \) for \( t – t’ < 0 \), or \( t’ > t \), the convolution can be written as
\begin{equation}\label{eqn:qftLecture11:900}
q(t)
= -\inv{\omega} \int_{-\infty}^t \sin(\omega(t – t’)) j(t’) dt’,
\end{equation}
which is now in a convient form to take derivatives. We have contributions from the boundary’s time dependence and from the integrand. In particular
\begin{equation}\label{eqn:qftLecture11:920}
\ddt{} \int_{a(t)}^{b(t)} g(x, t) dx
=
g(b(t)) b'(t) – g(a(t)) a'(t) + \int_a^b \frac{\partial}{\partial t} g(x, t) dx.
\end{equation}
Assuming that \( j(-\infty) = 0 \), this gives
\begin{equation}\label{eqn:qftLecture11:940}
\begin{aligned}
\ddt{q(t)}
&=
-\inv{\omega} \evalbar{\sin(\omega(t – t’)) j(t’) }{t’ = t}
-\int_{-\infty}^t \cos(\omega(t – t’)) j(t’) dt’ \\
&=
-\int_{-\infty}^t \cos(\omega(t – t’)) j(t’) dt’.
\end{aligned}
\end{equation}
For the second derivative we have
\begin{equation}\label{eqn:qftLecture11:960}
\begin{aligned}
q”(t)
&=
– \evalbar{ \cos(\omega(t – t’)) j(t’) }{t’ = t}
+\omega \int_{-\infty}^t \sin(\omega(t – t’)) j(t’) dt’ \\
&=
-j(t) -\omega^2
\int_{-\infty}^t \frac{-\sin(\omega(t – t’))}{\omega} j(t’) dt’,
\end{aligned}
\end{equation}
or
\begin{equation}\label{eqn:qftLecture11:980}
q”(t) = -j(t) – \omega^2 q(t),
\end{equation}
which is our forced Harmonic oscillator equation.

PHY2403H Quantum Field Theory. Lecture 10: Lorentz boosts, generator of spacetime translation, Lorentz invariant field representation. Taught by Prof. Erich Poppitz

October 16, 2018 phy2403 , , , , ,

[Click here for a PDF of this post with nicer formatting]

DISCLAIMER: Very rough notes from class, with some additional side notes.

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

Lorentz transform symmetries.

From last time, recall that an infinitesimal Lorentz transform has the form
\begin{equation}\label{eqn:qftLecture10:20}
x^\mu \rightarrow x^\mu + \omega^{\mu\nu} x_\nu,
\end{equation}
where
\begin{equation}\label{eqn:qftLecture10:40}
\omega^{\mu\nu} = -\omega^{\nu\mu}
\end{equation}

We showed last time that \( \omega^{ij} \) induces a rotation, and will show today that \( \omega^{0i} \) is a boost.

We introduced a three index current, factoring out explicit dependence on the incremental Lorentz transform tensor \( \omega^{\mu\nu} \) as follows
\begin{equation}\label{eqn:qftLecture10:80}
J^{\nu \mu\rho} = \inv{2} \lr{ x^\rho T^{\nu\mu} – x^\mu T^{\nu\rho} },
\end{equation}
and can easily show that this current has the desired zero four-divergence property
\begin{equation}\label{eqn:qftLecture10:100}
\begin{aligned}
\partial_\nu J^{\nu \mu\rho}
&= \inv{2} \lr{
(\partial_\nu x^\rho) T^{\nu\mu}
+
x^\rho {\partial_\nu T^{\nu\mu} }
– (\partial_\nu x^\mu) T^{\nu\rho}
– x^\mu {\partial_\nu T^{\nu\rho} }
} \\
&= \inv{2} \lr{
(\partial_\nu x^\rho) T^{\nu\mu}
– (\partial_\nu x^\mu) T^{\nu\rho}
} \\
&= \inv{2} \lr{
T^{\rho\mu}
+
– T^{\mu\rho}
} \\
&= 0,
\end{aligned}
\end{equation}
since the energy-momentum tensor is symmetric.

Defining charge in the usual fashion \( Q = \int d^3 x j^0 \), so we can define a charge for each pair of indexes \( \mu\nu \), and in particular
\begin{equation}\label{eqn:qftLecture10:120}
Q^{0k} = \int d^3 x J^{0 0 k} = \inv{2} \int d^3 x \lr{ x^k T^{00} – x^0 T^{0k} }
\end{equation}
\begin{equation}\label{eqn:qftLecture10:540}
\begin{aligned}
\dot{Q}^{0k}
&= \int d^3 x \dot{J}^{0 0k} \\
&= \inv{2} \int d^3 x \lr{ x^k \dot{T}^{00} – x^0 \dot{T}^{0k} }
\end{aligned}
\end{equation}

However, since \( 0 = \partial_\mu T^{\mu \nu} = \dot{T}^{0 \nu} + \partial_j T^{j \nu} \), or \( \dot{T}^{0 \nu} = -\partial_j T^{j \nu} \),
\begin{equation}\label{eqn:qftLecture10:560}
\begin{aligned}
\dot{Q}^{0k}
&= \inv{2} \int d^3 x \lr{ x^k (-\partial_j T^{j0}) – T^{0k} – x^0 (-\partial_j T^{jk}) } \\
&= \inv{2} \int d^3 x \lr{
\partial_j (-x^k T^{j0}) + (\partial_j x^k) T^{j0}
– T^{0k} + x^0 \partial_j T^{jk}
} \\
&= \inv{2} \int d^3 x \lr{
\partial_j (-x^k T^{j0}) + {T^{k0}}
– {T^{0k}} + x^0 \partial_j T^{jk}
} \\
&= \inv{2} \int d^3 x \lr{
\partial_j (-x^k T^{j0})
+ x^0 \partial_j T^{jk}
} \\
&= \inv{2} \int d^3 x
\partial_j \lr{
-x^k T^{j0}
+ x^0 T^{jk}
},
\end{aligned}
\end{equation}
which leaves just surface terms, so \( \dot{Q}^{0k} = 0 \).

Quantizing:

From our previous identification
\begin{equation}\label{eqn:qftLecture9:560}
–
{T^\nu}_\mu =
-\partial^\nu \phi \partial_\mu \phi + {\delta^{\nu}}_\mu \LL,
\end{equation}
we have
\begin{equation}\label{eqn:qftLecture10:580}
T^{\nu\mu} = \partial^\nu \phi \partial^\mu \phi – g^{\nu\mu} \LL,
\end{equation}
or
\begin{equation}\label{eqn:qftLecture10:600}
\begin{aligned}
T^{00}
&= \partial^0 \phi \partial^0 \phi – \inv{2} \lr{ \partial_0 \phi \partial^0 \phi + \partial_k \phi \partial^k \phi } \\
&= \inv{2} \partial^0 \phi \partial^0 \phi – \inv{2} (\spacegrad \phi)^2,
\end{aligned}
\end{equation}
and
\begin{equation}\label{eqn:qftLecture10:620}
T^{0k} = \partial^0 \phi \partial^k \phi,
\end{equation}
so we may quantize these energy momentum tensor components as
\begin{equation}\label{eqn:qftLecture10:640}
\begin{aligned}
\hatT^{00} &= \inv{2} \hat{\pi}^2 + \inv{2} (\spacegrad \phihat)^2 \\
\hatT^{0k} &= \inv{2} \hat{\pi} \partial^k \phihat.
\end{aligned}
\end{equation}

We can now start computing the commutators associated with the charge operator. The first of those commutators is
\begin{equation}\label{eqn:qftLecture10:140}
\antisymmetric{\hatT^{00}(\Bx)}{\phihat(\By)}
=
\inv{2}
\antisymmetric{\hat{\pi}^2(\Bx)}{\phihat(\By)},
\end{equation}
which can be evaluated using the field commutator analogue of \( \antisymmetric{F(p)}{q} = i F’ \) which is
\begin{equation}\label{eqn:qftLecture10:660}
\antisymmetric{F(\hat{\pi}(\Bx))}{\phihat(\By)} = -i \frac{dF}{d \hat{\pi}} \delta(\Bx – \By),
\end{equation}
to give
\begin{equation}\label{eqn:qftLecture10:680}
\antisymmetric{\hatT^{00}(\Bx)}{\phihat(\By)}
= -i \delta^3(\Bx – \By) \hat{\pi}(\Bx)
\end{equation}

The other required commutator is
\begin{equation}\label{eqn:qftLecture10:160}
\begin{aligned}
\antisymmetric{\hatT^{0i}(\Bx)}{\phihat(\By)}
&=
\antisymmetric{\hat{\pi}(\Bx)\partial^i \phihat(\Bx)}{\phihat(\By)} \\
&=
\partial^i \phihat(\Bx)
\antisymmetric{\hat{\pi}(\Bx)
}{\phihat(\By)} \\
&= -i \delta^3(\Bx – \By) \partial^i \phihat(\Bx),
\end{aligned}
\end{equation}

The charge commutator with the field can now be computed
\begin{equation}\label{eqn:qftLecture10:180}
\begin{aligned}
i \epsilon \antisymmetric{\hatQ^{0k}}{\phihat(\By)}
&=
i
\frac{\epsilon}{2} \int d^3 x
\lr{
x^k
\antisymmetric{\hatT^{00}}{\phihat(\By)}
–
x^0
\antisymmetric{\hatT^{0k}}{\phihat(\By)}
} \\
&=
\frac{\epsilon}{2} \lr{ y^k \hat{\pi}(\By) – y^0 \partial^k \phihat(\By) } \\
&=
\frac{\epsilon}{2} \lr{ y^k \dot{\phihat}(\By) – y^0 \partial^k \phihat(\By) },
\end{aligned}
\end{equation}
so to first order in \( \epsilon \)
\begin{equation}\label{eqn:qftLecture10:200}
e^{i \epsilon \hatQ^{0k} } \phihat(\By)
e^{-i \epsilon \hatQ^{0k} }
=
\phihat(\By)
+ \frac{\epsilon}{2} y^k \dot{\phihat}(\By)
+ \frac{\epsilon}{2} y^0 \partial_k \phihat(\By)
\end{equation}

For example, with \( k = 1 \)
\begin{equation}\label{eqn:qftLecture10:700}
\begin{aligned}
e^{i \epsilon \hatQ^{0k} } \phihat(\By)
e^{-i \epsilon \hatQ^{0k} }
&=
\phihat(\By)
+ \frac{\epsilon}{2} \lr{
y^1 \dot{\phihat}(\By)
+
y^0 \PD{y^1}{\phihat}(\By)
} \\
&=
\phihat(y^0 + \frac{\epsilon}{2} y^1,
y^1 + \frac{\epsilon}{2} y^2, y^3).
\end{aligned}
\end{equation}

This is a boost. If we compare explicitly to an infinitesimal Lorentz transformation of the coordinates
\begin{equation}\label{eqn:qftLecture10:220}
\begin{aligned}
x^0 \rightarrow x^0 + \omega^{01} x_1 &= x^0 – \omega^{01} x^1 \\
x^1 \rightarrow x^1 + \omega^{10} x_0 &= x^1 – \omega^{01} x_0 = x^1 – \omega^{01} x^0
\end{aligned}
\end{equation}
we can make the identification
\begin{equation}\label{eqn:qftLecture10:240}
\frac{\epsilon}{2} = – \omega^{01}.
\end{equation}

We now have the explicit form of the generator of a spacetime translation

\begin{equation}\label{eqn:qftLecture10:260}
\boxed{
\hatU(\Lambda) = \exp\lr{-i \omega^{0k} \int d^3 x \lr{ \hatT^{00} x^k – \hatT^{0k} x^0 }}
}
\end{equation}

An explicit boost along the x-axis has the form
\begin{equation}\label{eqn:qftLecture10:300}
\hatU(\Lambda) \phihat(t, \Bx)
\hatU^\dagger(\Lambda)
=
\phihat\lr{ \frac{t – vx}{\sqrt{1 – v^2}}, \frac{x – vt}{\sqrt{1 – v^2}}, y, z },
\end{equation}
and more generally
\begin{equation}\label{eqn:qftLecture10:320}
\hatU(\Lambda) \phihat(x) \hatU^\dagger(\Lambda) =
\phihat(\Lambda x)
\end{equation}
where \( x \) is a four vector, \( (\Lambda x)^\mu = {{\Lambda}^\mu}_\nu x^\nu \), and \(
{{\Lambda}^\mu}_\nu
\approx
{{\delta}^\mu}_\nu
+
{{\omega}^\mu}_\nu \).

Transformation of momentum states

In the momentum space representation

\begin{equation}\label{eqn:qftLecture10:340}
\begin{aligned}
\phihat(x)
&=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}} \lr{
e^{i (\omega_\Bp t – \Bp \cdot \Bx)} \hat{a}_\Bp
+
e^{-i (\omega_\Bp t – \Bp \cdot \Bx)} \hat{a}^\dagger_\Bp
} \\
&=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}} \evalbar{
\lr{
e^{i p^\mu x^\mu } \hat{a}_\Bp
+
e^{-i p^\mu x^\mu } \hat{a}^\dagger_\Bp
}
}{p_0 = \omega_\Bp}
\end{aligned}
\end{equation}
\begin{equation}\label{eqn:qftLecture10:720}
\begin{aligned}
\hatU(\Lambda) \phihat(x) \hatU^\dagger(\Lambda)
&=
\phihat(\Lambda x) \\
&=
\int \frac{d^3 p}{(2 \pi)^3 \sqrt{2 \omega_\Bp}} \evalbar{
\lr{
e^{i p^\mu {{\Lambda}^\mu}_\nu x^\nu }
\hat{a}_\Bp
+
e^{-i p^\mu {{\Lambda}^\mu}_\nu x^\nu } \hat{a}^\dagger_\Bp
}
}{p_0 = \omega_\Bp}
\end{aligned}
\end{equation}
This can be put into an explicitly Lorentz invariant form
\begin{equation}\label{eqn:qftLecture10:n}
\begin{aligned}
\phihat(\Lambda x)
&=
\int \frac{dp^0 d^3 p}{(2\pi)^3} \delta(p_0^2 – \Bp^2 – m^2) \Theta(p^0) \sqrt{2 \omega_\Bp}
e^{i p^\mu {{\Lambda}^\mu}_\nu x^\nu }
\hat{a}_\Bp + \text{h.c.} \\
&=
\int \frac{dp^0 d^3 p}{(2\pi)^3}
\lr{
\frac{\delta(p_0 – \omega_\Bp)}{2 \omega_\Bp}
+
\frac{\delta(p_0 + \omega_\Bp)}{2 \omega_\Bp}
}
\Theta(p^0) \sqrt{2 \omega_\Bp} \hat{a}_\Bp + \text{h.c.},
\end{aligned}
\end{equation}
which recovers \ref{eqn:qftLecture10:720} by making use of the delta function identity \( \delta(f(x)) = \sum_{f(x_\conj) = 0} \frac{\delta(x – x_\conj)}{f'(x_\conj)} \), since the \( \Theta(p^0) \) kills the second delta function.

We now have a more explicit Lorentz invariant structure
\begin{equation}\label{eqn:qftLecture10:380}
\phihat(\Lambda x)
=
\int \frac{dp^0 d^3 p}{(2\pi)^3} \delta(p_0^2 – \Bp^2 – m^2) \Theta(p^0) \sqrt{2 \omega_\Bp}
e^{i p^\mu {{\Lambda}^\mu}_\nu x^\nu }
\hat{a}_\Bp + \text{h.c.}
\end{equation}

Recall that a boost moves a spacetime point along a parabola, such as that of fig. 1, whereas a rotation moves along a constant “circular” trajectory of a hyper-paraboloid. In general, a Lorentz transformation may move a spacetime point along any path on a hyper-paraboloid such as the one depicted (in two spatial dimensions) in fig. 2. This paraboloid depict the surfaces of constant energy-momentum \( p^0 = \sqrt{ \Bp^2 + m^2 } \). Because a Lorentz transformation only shift points along that energy-momentum surface, but cannot change the sign of the energy coordinate \( p^0 \), this means that \( \Theta(p^0) \) is also a Lorentz invariant.

fig. 1. One dimensional spacetime surface for constant (p^0)^2 – p^2 = m^2.

 

fig. 2. Surface of constant squared four-momentum.

 

Let’s change variables
\begin{equation}\label{eqn:qftLecture10:400}
p^\lambda = {{\Lambda}^\lambda}_\rho {p’}^{\rho}
\end{equation}
so that
\begin{equation}\label{eqn:qftLecture10:420}
\begin{aligned}
p_\mu
{{\Lambda}^\mu}_\nu x^\nu
&=
{{\Lambda}^\lambda}_\rho {p’}^\rho g_{\lambda\nu} {{\Lambda}^\nu}_\sigma x^{\sigma} \\
&=
{p’}^\rho
\lr{ {{\Lambda}^\lambda}_\rho
g_{\lambda\nu} {{\Lambda}^\nu}_\sigma } x^{\sigma} \\
&=
{p’}^\rho g_{\rho\sigma} x^\sigma
\end{aligned}
\end{equation}
which gives
\begin{equation}\label{eqn:qftLecture10:440}
\begin{aligned}
\phihat(\Lambda x)
&=
\int \frac{d{p’}^0 d^3 p’}{(2\pi)^3} \delta({p’}_0^2 – {\Bp’}^2 – m^2) \Theta(p^0) \sqrt{2 \omega_{\Lambda \Bp’}} e^{i p’ \cdot x} \hat{a}_{\Lambda \Bp’} + \text{h.c.} \\
&=
\int \frac{dp^0 d^3 p}{(2\pi)^3} \delta({p}_0^2 – {\Bp}^2 – m^2) \Theta(p^0) \sqrt{2 \omega_{\Lambda \Bp}} e^{i p \cdot x} \hat{a}_{\Lambda \Bp} + \text{h.c.}
\end{aligned}
\end{equation}
Since
\begin{equation}\label{eqn:qftLecture10:460}
\phihat(x)
=
\int \frac{dp^0 d^3 p}{(2\pi)^3} \delta({p}_0^2 – {\Bp}^2 – m^2) \Theta(p^0) \sqrt{2 \omega_{\Bp}} e^{i p \cdot x} \hat{a}_{\Bp} + \text{h.c.}
\end{equation}
we can now conclude that the creation and annihilation operators transform as

\begin{equation}\label{eqn:qftLecture10:480}
\boxed{
\sqrt{2 \omega_{\Lambda \Bp}} \hat{a}_{\Lambda \Bp}
=
\hatU(\Lambda)
\sqrt{2 \omega_{ \Bp}} \hat{a}_{ \Bp}
\hatU^\dagger(\Lambda)
}
\end{equation}

In particular
\begin{equation}\label{eqn:qftLecture10:500}
\sqrt{2 \omega_{ \Bp}} \hat{a}^\dagger_{ \Bp} \ket{0} = \ket{\Bp}
\end{equation}
and noting that \( \hatU(\Lambda) \ket{0} = \ket{0} \) (i.e. the ground state is Lorentz invariant), we have
\begin{equation}\label{eqn:qftLecture10:520}
\begin{aligned}
\sqrt{2 \omega_{\Lambda \Bp}} \hat{a}^\dagger_{\Lambda \Bp} \ket{0}
&=
\hatU(\Lambda) \sqrt{ 2\omega_\Bp} \hat{a}^\dagger_\Bp \hatU^\dagger(\Lambda) \hatU(\Lambda) \ket{0} \\
&=
\hatU(\Lambda) \sqrt{ 2\omega_\Bp} \hat{a}^\dagger_\Bp \ket{0} \\
&=
\hatU(\Lambda) \ket{\Bp}.
\end{aligned}
\end{equation}

PHY2403H Quantum Field Theory. Lecture 8: 1st Noether theorem, spacetime translation current, energy momentum tensor, dilatation current. Taught by Prof. Erich Poppitz

October 14, 2018 phy2403 , , , , , , , , , , , , , ,

[Click here for a PDF of this post with nicer formatting]

DISCLAIMER: Very rough notes from class, with some additional side notes.

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

1st Noether theorem.

Recall that, given a transformation
\begin{equation}\label{eqn:qftLecture8:20}
\phi(x) \rightarrow \phi(x) + \delta \phi(x),
\end{equation}
such that the transformation of the Lagrangian is only changed by a total derivative
\begin{equation}\label{eqn:qftLecture8:40}
\LL(\phi, \partial_\mu \phi) \rightarrow
\LL(\phi, \partial_\mu \phi)
+ \partial_\mu J_\epsilon^\mu,
\end{equation}
then there is a conserved current
\begin{equation}\label{eqn:qftLecture8:60}
j^\mu = \PD{(\partial_\mu \phi)}{\LL} \delta_\epsilon \phi – J_\epsilon^\mu.
\end{equation}
Here \( \epsilon \) is an x-independent quantity (i.e. a \underline{global symmetry}).
This is in contrast to “gauge symmetries”, which can be more accurately be categorized as a redundancy in the description.

As an example, for \( \LL = (\partial_\mu \phi \partial^\mu \phi – m^2 \phi^2)/2 \), let
\begin{equation}\label{eqn:qftLecture8:80}
\phi(x) \rightarrow \phi(x) – a^\mu \partial_\mu \phi
\end{equation}
\begin{equation}\label{eqn:qftLecture8:100}
\LL(\phi, \partial_\mu \phi) \rightarrow
\LL(\phi, \partial_\mu \phi)
– a^\mu \partial_\mu \LL
=
\LL(\phi, \partial_\mu \phi)
+ \partial_\mu \lr{ -{\delta^\mu}_\nu a^\nu \LL }
\end{equation}
Here \( J^\mu_\epsilon = \evalbar{J^\mu_\epsilon}{\epsilon = a^\nu} \), and the current is
\begin{equation}\label{eqn:qftLecture8:120}
J^\mu = (\partial^\mu \phi)(-a^\nu \partial_\nu \phi) + {\delta^{\mu}}_\nu a^\nu \LL.
\end{equation}
In particular, we have one such current for each \( \nu \), and we write
\begin{equation}\label{eqn:qftLecture8:140}
{T^\mu}_\nu =
-(\partial^\mu \phi)(\partial_\nu \phi) + {\delta^{\mu}}_\nu \LL.
\end{equation}
By Noether’s theorem, we must have
\begin{equation}\label{eqn:qftLecture8:160}
\partial_\mu
{T^\mu}_\nu = 0, \quad \forall \nu.
\end{equation}

Check:

\begin{equation}\label{eqn:qftLecture8:1380}
\begin{aligned}
\partial_\mu {T^\mu}_\nu
&=
-(\partial_\mu \partial^\mu \phi)(\partial_\nu \phi)
-(\partial^\mu \phi)(\partial_\mu \partial_\nu \phi)
+ {\delta^{\mu}}_\nu
\partial_\mu \lr{
\inv{2} \partial_\alpha \phi \partial^\alpha \phi – \frac{m^2}{2} \phi^2
} \\
&=
-(\partial_\mu \partial^\mu \phi)(\partial_\nu \phi)
-(\partial^\mu \phi)(\partial_\mu \partial_\nu \phi)
+
\inv{2} (\partial_\nu \partial_\mu \phi) (\partial^\mu \phi )
+
\inv{2} (\partial_\mu \phi) (\partial_\nu \partial^\mu \phi )
– m^2 (\partial_\nu \phi) \phi \\
&=
-\lr{ \partial_\mu \partial^\mu \phi + m^2 \phi }(\partial_\nu \phi)
-(\partial_\mu \phi)(\partial^\mu \partial_\nu \phi)
+
\inv{2} (\partial_\nu \partial^\mu \phi) (\partial_\mu \phi )
+
\inv{2} (\partial_\mu \phi) (\partial_\nu \partial^\mu \phi )
&= 0.
\end{aligned}
\end{equation}

Example: our potential Lagrangian

\begin{equation}\label{eqn:qftLecture8:180}
\LL = \inv{2} \partial^\mu \phi \partial_\nu \phi – \frac{m^2}{2} \phi^2 – \frac{\lambda}{4} \phi^4
\end{equation}
Written with upper indexes
\begin{equation}\label{eqn:qftLecture8:200}
\begin{aligned}
T^{\mu\nu}
&= -(\partial^\mu \phi)(\partial^\nu \phi) + g^{\mu\nu} \LL \\
&= -(\partial^\mu \phi)(\partial^\nu \phi) + g^{\mu\nu} \lr{
\inv{2} \partial^\alpha \phi \partial_\alpha \phi – \frac{m^2}{2} \phi^2 – \frac{\lambda}{4} \phi^4
}
\end{aligned}
\end{equation}

There are 4 conserved currents \( J^{\mu(\nu)} = T^{\mu\nu} \). Observe that this is symmetric (\( T^{\mu\nu} = T^{\nu\mu} \)).

We have four associated charges
\begin{equation}\label{eqn:qftLecture8:220}
Q^\nu = \int d^3 x T^{0 \nu}.
\end{equation}
We call
\begin{equation}\label{eqn:qftLecture8:240}
Q^0 = \int d^3 x T^{0 0},
\end{equation}
the energy density, and call
\begin{equation}\label{eqn:qftLecture8:260}
P^i = \int d^3 x T^{0 i},
\end{equation}
(i = 1,2,3) the momentum density.

writing this out explicitly the energy density is
\begin{equation}\label{eqn:qftLecture8:280}
\begin{aligned}
T^{00}
&= – \dot{\phi}^2 + \inv{2} \lr{ \dot{\phi}^2 – (\spacegrad \phi)^2 – \frac{m^2}{2}\phi^2 – \frac{\lambda}{4} \phi^4} \\
&= -\lr{
\inv{2} \dot{\phi}^2 + \inv{2} (\spacegrad \phi)^2 + \frac{m^2}{2}\phi^2 + \frac{\lambda}{4} \phi^4
},
\end{aligned}
\end{equation}
and
\begin{equation}\label{eqn:qftLecture8:300}
T^{0i} = \partial^0 \phi \partial^i \phi,
\end{equation}
\begin{equation}\label{eqn:qftLecture8:320}
P^{i} = -\int d^3 x\partial^0 \phi \partial^i \phi
\end{equation}
Since the energy density is negative definite (due to an arbitrary choice of translation sign), let’s redefine \( T^{\mu\nu} \) to have a positive sign
\begin{equation}\label{eqn:qftLecture8:340}
T^{00}
\equiv
\inv{2} \dot{\phi}^2 + \inv{2} (\spacegrad \phi)^2 + \frac{m^2}{2} \phi^2 + \frac{\lambda}{4} \phi^4,
\end{equation}
and
\begin{equation}\label{eqn:qftLecture8:360}
P^{i} = \int d^3 x\partial^0 \phi \partial^i \phi
\end{equation}

As an operator we have
\begin{equation}\label{eqn:qftLecture8:380}
\hatQ = \int d^3 x \hatT^{00} =
\int d^3 x
\lr{
\inv{2} \hat{\pi}^2 + \inv{2} (\spacegrad \phihat)^2 + \frac{m^2}{2} \phihat^2 + \frac{\lambda}{4} \phihat^4
}.
\end{equation}
\begin{equation}\label{eqn:qftLecture8:400}
\hatP^{i} = \int d^3 x \hat{\pi} \partial^i \phi
\end{equation}

We showed that
\begin{equation}\label{eqn:qftLecture8:420}
\ddt{\hatO} = i \antisymmetric{\hatH}{\hatO}
\end{equation}
This implied that \( \phihat, \hat{\pi} \) obey the classical EOMs
\begin{equation}\label{eqn:qftLecture8:440}
\ddt{\phihat} = i \antisymmetric{\hat{H}}{\phihat} = \ddt{\hat{\pi}}
\end{equation}
\begin{equation}\label{eqn:qftLecture8:460}
\ddt{\hat{\pi}} = i \antisymmetric{\hatH}{\hat{\pi}} = …
\end{equation}

In terms of creation and annihilation operators (for the \( \lambda = 0 \) free field), up to a constant
\begin{equation}\label{eqn:qftLecture8:480}
\begin{aligned}
\hatH
&= \int d^3 x \hatT^{00} \\
&= \int \frac{d^3 p}{(2 \pi)^3} \omega_\Bp \hat{a}_\Bp^\dagger \hat{a}_\Bp
\end{aligned}
\end{equation}
Can show that:

\begin{equation}\label{eqn:qftLecture8:500}
\begin{aligned}
\hatP^i
&= \int d^3 x \hat{\pi} \partial^i \phihat \\
&= \cdots \\
&= \int \frac{d^3 p}{(2 \pi)^3} p^i \hat{a}_\Bp^\dagger \hat{a}_\Bp
\end{aligned}
\end{equation}
Now we see the energy and momentum as conserved quantities associated with spacetime translation.

Unitary operators

In QM we say that \( \hat{\Bp} \) “generates translations”.

With \( \hat{\Bp} \equiv -i \Hbar \spacegrad \) that translation is
\begin{equation}\label{eqn:qftLecture8:520}
\hatU = e^{i \Ba \cdot \hat{\Bp}} = e^{\Ba \cdot \spacegrad}
\end{equation}

In particular
\begin{equation}\label{eqn:qftLecture8:540}
\bra{\Bx} \hatU \ket{\psi} = e^{\Ba \cdot \hat{\Bp} } \psi(\Bx) = \psi(\Bx + \Ba).
\end{equation}

In one dimension
\begin{equation}\label{eqn:qftLecture8:560}
\begin{aligned}
\hatU \hat{x} \hatU^\dagger
&=
e^{\Ba \cdot \hat{p} } \psi(\Bx)
e^{-\Ba \cdot \hat{p} } \\
&= \hat{\Bx} + a \hat{\mathbf{1}}.
\end{aligned}
\end{equation}
This uses the Baker-Campbell-Hausdorff formula.

Theorem: Baker-Campbell-Hausdorff

\begin{equation}\label{eqn:qftLecture8:600}
e^{B} A e^{-B} = \sum_{n = 0}^\infty \inv{n!} \antisymmetric{B \cdots}{\antisymmetric{B}{A}},
\end{equation}
where the n-th commutator is denoted above

  • \( n = 1 \) : \( \antisymmetric{B}{A} \)
  • \( n = 2 \) : \( \antisymmetric{B}{\antisymmetric{B}{A}} \)
  • \( n = 3 \) : \( \antisymmetric{B}{\antisymmetric{B}{\antisymmetric{B}{A}}} \)

Proof:

\begin{equation}\label{eqn:qftLecture8:620}
\begin{aligned}
f(t)
&= e^{tB} A e^{-tB} \\
&= f(0) + t f'(0) + \frac{t^2}{2} f”(0) + \cdots \frac{t^n}{n!} f^{(n)}(0)
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture8:640}
f(0) = A
\end{equation}
\begin{equation}\label{eqn:qftLecture8:660}
\begin{aligned}
f'(t)
&=
e^{tB} B A e^{-tB}
+
e^{tB} A (-B) e^{-tB} \\
&=
e^{tB} \antisymmetric{B}{A} e^{-tB}
\end{aligned}
\end{equation}
\begin{equation}\label{eqn:qftLecture8:680}
\begin{aligned}
f”(t)
&=
e^{tB} B \antisymmetric{B}{A} e^{-tB}
+
e^{tB} \antisymmetric{B}{A} (-B) e^{-tB} \\
&=
e^{tB} \antisymmetric{B}{\antisymmetric{B}{A}} e^{-tB}.
\end{aligned}
\end{equation}
From
\begin{equation}\label{eqn:qftLecture8:700}
f(1)
= f(0) + f'(0) + \inv{2} f”(0) + \cdots \inv{n!} f^{(n)}(0)
\end{equation}
we have
\begin{equation}\label{eqn:qftLecture8:720}
e^{B} A e^{-B} = A +
\antisymmetric{B}{A} + \inv{2} \antisymmetric{B}{\antisymmetric{B}{A}} + \cdots
\end{equation}

Example:
\begin{equation}\label{eqn:qftLecture8:740}
\begin{aligned}
e^{a \partial_x} x e^{-a \partial_x }
&= x + a \antisymmetric{\partial_x}{x} + \cdots \\
&= x + a.
\end{aligned}
\end{equation}

Application:

\begin{equation}\label{eqn:qftLecture8:760}
e^{i \text{Hermitian} } = \text{unitary}
\end{equation}
\begin{equation}\label{eqn:qftLecture8:860}
e^{i \text{Hermitian} } \times
e^{-i \text{Hermitian} }
= 1
\end{equation}
So
\begin{equation}\label{eqn:qftLecture8:780}
\hatU(\Ba) =
e^{i a^j \hat{p}^j }
\end{equation}
is a unitary operator representing finite translations in a Hilbert space.

\begin{equation}\label{eqn:qftLecture8:800}
\begin{aligned}
\hatU(\Ba) \phihat(\Bx) \hatU^\dagger(\Ba)
&=
e^{i a^j \hat{p}^j }
\phihat(\Bx)
e^{-i a^k \hat{p}^k } \\
&=
\phihat(\Bx)
+ i a^j \antisymmetric{\hatP^j}{\phihat(\Bx)} + \frac{-a^{j_1} a^{j_2}}{2} \antisymmetric{\hatP^{j_1}}{\antisymmetric{\hatP^{j_2}}{\phihat(\Bx)}}
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture8:820}
\begin{aligned}
\antisymmetric{\hatP^j}{\phihat(\Bx)}
&=
\int d^3 y \antisymmetric{\hat{\pi}(\By) \partial^j \phihat(\By)}{\phihat(\Bx)} \\
&=
\int d^3 y \antisymmetric{\hat{\pi}(\By)}{\phihat(\Bx} \partial^j \phihat(\By) \\
&=
\int d^3 y (-i ) \delta^3(\By – \Bx) \partial^j \phihat(\By) \\
&=
-i \partial^j \phihat(\Bx).
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture8:840}
\begin{aligned}
\hatU(\Ba) \phihat(\Bx) \hatU^\dagger(\Ba)
&= \phihat(\Bx) + i a^j (-i) \partial^j \phihat(\Bx) + \cdots \\
&= \phihat(\Bx) + a^j \partial^j \phihat(\Bx) + \cdots \\
&= \phihat(\Bx + \Ba)
\end{aligned}
\end{equation}

Continuous symmetries

For all infinitesimal transformations, continuous symmetries lead to conserved charges \( Q \). In QFT we map these charges to Hermitian operators \( Q \rightarrow \hatQ \). We say that these charges are “generators of the corresponding symmetry” through unitary operators
\begin{equation}\label{eqn:qftLecture8:880}
\hatU = e^{i \text{parameter} \hatQ}.
\end{equation}
These represent the action of the symmetry in the Hilbert space.

Example: spatial translation

\begin{equation}\label{eqn:qftLecture8:900}
\hatU(\Ba) = e^{i \Ba \cdot \hat{\BP}}
\end{equation}

Example: time translation

\begin{equation}\label{eqn:qftLecture8:920}
\hatU(t) = e^{i t \hat{H}}.
\end{equation}

Classical scalar theory

For \( d > 2 \) let’s look at
\begin{equation}\label{eqn:qftLecture8:940}
S =
\int d^d x \lr{
\inv{2} \partial^\mu \phi \partial_\mu \phi – \frac{m^2}{2} \phi^2 – \lambda \phi^{d-2}
}
\end{equation}

Take \( m^2, \lambda \rightarrow 0 \), the free massless scalar field.

We have a shift symmetry in this case since \( \phi(x) \rightarrow \phi(x) + \text{constant} \).
The current is just
\begin{equation}\label{eqn:qftLecture8:960}
\begin{aligned}
j^\mu
&= \PD{(\partial_\mu \phi)}{\phi} \delta \phi – J^\mu \\
&= \PD{(\partial_\mu \phi)}{\phi} \delta \phi \\
&= \text{constant} \times \partial^\mu \phi \\
&= \partial^\mu \phi,
\end{aligned}
\end{equation}
where the constant factor has been set to one.
This current is clearly conserved since \( \partial_\mu J^\mu = \partial_\mu \partial^\mu \phi = 0\) (the equation of motion).

These are called “Goldstein Bosons”.

With \( m = \lambda = 0, d = 4 \) we have

NOTE: We did this in class differently with \( d \ne 4, m, \lambda \ne 0\), and then switched to \( m = \lambda = 0, d = 4\), which was confusing. I’ve reworked my notes to \( d = 4 \) like the supplemental handout that did the same.

\begin{equation}\label{eqn:qftLecture8:980}
S =
\int d^4 x \lr{
\inv{2} \partial^\mu \phi \partial_\mu \phi
}
\end{equation}
Here we have a scale or dilatation invariance
\begin{equation}\label{eqn:qftLecture8:1000}
x \rightarrow x’ = e^{\lambda} x,
\end{equation}
\begin{equation}\label{eqn:qftLecture8:1020}
\phi(x) \rightarrow \phi'(x’) = e^{-\lambda} \phi,
\end{equation}
\begin{equation}\label{eqn:qftLecture8:1040}
d^4 x \rightarrow d^4 x’ = e^{4\lambda} d^4 x,
\end{equation}

The partials transform as
\begin{equation}\label{eqn:qftLecture8:1400}
\partial^\mu \rightarrow
\PD{x’_\mu}{}
=
\PD{x’_\mu}{x_\mu}
\PD{x_\mu}{}
=
e^{-\lambda}
\PD{x_\mu}{}
\end{equation}

so the partial of the field transforms as
\begin{equation}\label{eqn:qftLecture8:1420}
\partial^\mu \phi(x) \rightarrow \PD{x’_\mu}{\phi'(x’)} = e^{-2\lambda} \partial^\mu \phi(x),
\end{equation}
and finally
\begin{equation}\label{eqn:qftLecture8:1060}
(\partial_\mu \phi)^2 \rightarrow e^{-4\lambda} \lr{ \partial_\mu \phi(x) }^2.
\end{equation}

With a \( -4 \lambda \) power in the transformed quadratic term, and \( 4 \lambda \) in the volume element, we see that the action is invariant.

To find Noether current, we need to vary the field and it’s derivatives
\begin{equation}\label{eqn:qftLecture8:1100}
\begin{aligned}
\delta_\lambda \phi
&= \phi'(x) – \phi(x) \\
&= \phi'(e^{-\lambda} x’) – \phi(x) \\
&\approx \phi'(x’ -\lambda x’) – \phi(x) \\
&\approx \phi'(x’) – \lambda {x’}^\alpha \partial_\alpha \phi'(x’) – \phi(x) \\
&\approx (1 – \lambda) \phi(x) – \lambda {x’}^\alpha \partial_\alpha \phi'(x’) – \phi(x) \\
&= – \lambda(1 + x^\alpha \partial_\alpha ) \phi,
\end{aligned}
\end{equation}
where the last step assumes that \( x’ \rightarrow x, \phi’ \rightarrow \phi \), effectively weeding out any terms that are quadratic or higher in \( \lambda \).

Now we need the variation of the derivatives of \( \phi \)
\begin{equation}\label{eqn:qftLecture8:1440}
\delta \partial_\mu \phi(x)
=
\partial_\mu’ \phi'(x) – \partial_\mu \phi(x),
\end{equation}
By \ref{eqn:qftLecture8:1420}
\begin{equation}\label{eqn:qftLecture8:1460}
\begin{aligned}
\partial_\mu’ \phi'(x’)
&=
e^{-2\lambda} \partial_\mu \phi(x) \\
&=
e^{-2\lambda} \partial_\mu \phi(e^{-\lambda} x’) \\
&\approx
e^{-2\lambda} \partial_\mu
\lr{
\phi(x’) – \lambda {x’}^\alpha \partial_\alpha \phi(x’)
} \\
&\approx
\lr{
1 – 2 \lambda
}
\partial_\mu
\lr{
\phi(x’) – \lambda {x’}^\alpha \partial_\alpha \phi(x’)
},
\end{aligned}
\end{equation}
so
\begin{equation}\label{eqn:qftLecture8:1480}
\begin{aligned}
\delta \partial_\mu \phi
&=
– \lambda {x}^\alpha \partial_\alpha \partial_\mu \phi(x)
– 2 \lambda \partial_\mu \phi(x) + O(\lambda^2) \\
&=
– \lambda \lr{
{x}^\alpha \partial_\alpha + 2
}
\partial_\mu \phi(x).
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture8:1200}
\begin{aligned}
\delta \LL
&=
(\partial^\mu \phi) \delta (\partial_\mu \phi) \\
&= – \lambda \lr{ 2
\partial_\mu \phi
+ x^\alpha \partial_\alpha
\partial_\mu \phi
}
\partial^\mu \phi,
\end{aligned}
\end{equation}
or
\begin{equation}\label{eqn:qftLecture8:1500}
\begin{aligned}
\frac{\delta \LL }{-\lambda}
&=
4 \LL + x^\alpha \lr{ \partial_\alpha \partial_\mu \phi } \partial^\mu \phi \\
&=
4 \LL + x^\alpha \partial_\alpha \lr{ \LL } \\
&=
{4 \LL} + \partial_\alpha \lr{ x^\alpha \LL } – {\LL \partial_\alpha x^\alpha} \\
&=
\partial_\alpha \lr{ x^\alpha \LL }.
\end{aligned}
\end{equation}
The variation in the Lagrangian density is thus
\begin{equation}\label{eqn:qftLecture8:1520}
\delta \LL = \partial_\mu J^\mu_\lambda = \partial_\mu \lr{ -\lambda x^\mu \LL },
\end{equation}
and the current is
\begin{equation}\label{eqn:qftLecture8:1540}
J^\mu_\lambda = -\lambda x^\mu \LL.
\end{equation}

The Noether current is
\begin{equation}\label{eqn:qftLecture8:1240}
\begin{aligned}
j^\mu
&= \PD{(\partial_\mu \phi)}{\LL} \delta \phi – J^\mu \\
&= -\partial^\mu \phi \lr{ 1 + x^\nu \partial_\nu } \phi + \inv{2} x^\mu \partial_\nu \phi \partial^\nu \phi,
\end{aligned}
\end{equation}
or after flipping signs
\begin{equation}\label{eqn:qftLecture8:1280}
\begin{aligned}
j^\mu_{\text{dil}}
&= \partial^\mu \phi \lr{ 1 + x^\nu \partial_\nu } \phi – \inv{2} x^\mu
\partial_\nu \phi \partial^\nu \phi \\
&= x_\nu \lr{ \partial^\mu \phi \partial^\nu \phi – \inv{2} {\delta^{\nu}}_\mu \partial_\lambda \phi \partial^\lambda \phi }
+ \inv{2} \partial^\mu (\phi^2),
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture8:1300}
j^\mu_{\text{dil}} = -x_\nu T^{\nu \mu} + \inv{2} \partial^\mu (\phi^2),
\end{equation}

The current and \( T^{\mu\nu} \) can both be redefined \( j^{\mu’} = j^\mu + \partial_\nu C^{\nu\mu} \) adding an antisymmetric \( C^{\mu\nu} = -C^{\nu\mu} \)

\begin{equation}\label{eqn:qftLecture8:1320}
j^\mu_{\text{dil conformal}} = – x_\nu T^{\nu\mu}_{\text{conformal}}
\end{equation}

\begin{equation}\label{eqn:qftLecture8:1340}
\partial_\mu
j^\mu_{\text{dil conformal}} = – {{T_{\text{conformal}}}^\mu}_\mu
\end{equation}

consequence: \( 0 = T^{00} – T^{11} – T^{22} – T^{33} \), which is essentially
\begin{equation}\label{eqn:qftLecture8:1360}
0 = \rho – 3 p = 0.
\end{equation}

PHY2403H Quantum Field Theory. Lecture 9: Unbroken and spontaneously broken symmetries, Higgs Lagrangian, scale invariance, Lorentz invariance, angular momentum quantization. Taught by Prof. Erich Poppitz

October 11, 2018 phy2403 , , , , , , , , , , , , , , ,

[Click here for a PDF of this post with nicer formatting (and a Mathematica listing that I didn’t include in this blog post’s latex export)]

DISCLAIMER: Very rough notes from class, with some additional side notes.

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

Last time

We followed a sequence of operations

  1. Noether’s theorem
  2. \( \rightarrow \) conserved currents
  3. \( \rightarrow \) charges (classical)
  4. \( \rightarrow \) “correspondence principle”
  5. \( \rightarrow \hatQ \)
  • Hermitian operators
  • “generators of symmetry”
    \begin{equation}\label{eqn:qftLecture9:20}
    \hatU(\alpha) = e^{i \alpha \hatQ}
    \end{equation}
    We found
    \begin{equation}\label{eqn:qftLecture9:40}
    \hatU(\alpha) \phihat \hatU^\dagger(\alpha) = \phihat + i \alpha \antisymmetric{\hatQ}{\phihat} + \cdots
    \end{equation}

Example: internal symmetries:

(non-spacetime), such as \( O(N)\) or \( U(1) \).

In QFT internal symmetries can have different “\underline{modes of realization}”.

    [I]

  1. “Wigner mode”. These are also called “unbroken symmetries”.
    \begin{equation}\label{eqn:qftLecture9:60}
    \hatQ \ket{0} = 0
    \end{equation}
    i.e. \( \hatU(\alpha) \ket{0} = 0 \).
    Ground state invariant. Formally \( :\hatQ: \) annihilates \( \ket{0} \).
    \( \antisymmetric{\hatQ}{\hatH} = 0 \) implies that all eigenstates are eigenstates of \( \hatQ \) in \( U(1) \). Example from HW 1
    \begin{equation}\label{eqn:qftLecture9:80}
    \hatQ = \text{“charge” under \( U(1) \)}.
    \end{equation}
    All states have definite charge, just live in QU.
  2. “Nambu-Goldstone mode” (Landau-ginsburg). This is also called a “spontaneously broken symmetry”\footnote{
    First encounter example (HWII, \( SU(2) \times SU(2) \rightarrow SU(2) \)). Here a \( U(1) \) spontaneous broken symmetry.}.
    \( H \) or \( L \) is invariant under symmetry, but ground state is not.

fig. 1. Mexican hat potential.

fig. 2. Degenerate Mexican hat potential ( v = 0)

Example:
\begin{equation}\label{eqn:qftLecture9:100}
\LL = \partial_\mu \phi^\conj \partial^\mu \phi – V(\Abs{\phi}),
\end{equation}
where
\begin{equation}\label{eqn:qftLecture9:120}
V(\Abs{\phi}) = m^2 \phi^\conj \phi + \frac{\lambda}{4} \lr{ \phi^\conj \phi }^2.
\end{equation}
When \( m^2 > 0 \) we have a Wigner mode, but when \( m^2 < 0 \) we have an issue: \( \phi = 0 \) is not a minimum of potential.
When \( m^2 < 0 \) we write
\begin{equation}\label{eqn:qftLecture9:140}
\begin{aligned}
V(\phi)
&= – m^2 \phi^\conj \phi + \frac{\lambda}{4} \lr{ \phi^\conj \phi}^2 \\
&= \frac{\lambda}{4} \lr{
\lr{ \phi^\conj \phi}^2 – \frac{4}{\lambda} m^2 } \\
&= \frac{\lambda}{4} \lr{
\phi^\conj \phi – \frac{2}{\lambda} m^2 }^2 – \frac{4 m^4}{\lambda^2},
\end{aligned}
\end{equation}
or simply
\begin{equation}\label{eqn:qftLecture9:780}
V(\phi)
=
\frac{\lambda}{4} \lr{ \phi^\conj \phi – v^2 }^2 + \text{const}.
\end{equation}
The potential (called the Mexican hat potential) is illustrated in fig. 1 for non-zero \( v \), and in
fig. 2 for \( v = 0 \).
We choose to expand around some point on the minimum ring (it doesn’t matter which one).
When there is no potential, we call the field massless (i.e. if we are in the minimum ring).
We expand as
\begin{equation}\label{eqn:qftLecture9:160}
\phi(x) = v \lr{ 1 + \frac{\rho(x)}{v} } e^{i \alpha(x)/v },
\end{equation}
so
\begin{equation}\label{eqn:qftLecture9:180}
\begin{aligned}
\frac{\lambda}{4}
\lr{\phi^\conj \phi – v^2}^2
&=
\lr{
v^2 \lr{ 1 + \frac{\rho(x)}{v} }^2
– v^2
}^2 \\
&=
\frac{\lambda}{4}
v^4 \lr{ \lr{ 1 + \frac{\rho(x)}{v} }^2 – 1 } \\
&=
\frac{\lambda}{4}
v^4
\lr{
\frac{2 \rho}{v} + \frac{\rho^2}{v^2}
}^2.
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:qftLecture9:200}
\partial_\mu \phi =
\lr{
v \lr{ 1 + \frac{\rho(x)}{v} } \frac{i}{v} \partial_\mu \alpha
+ \partial_\mu \rho
} e^{i \alpha}
\end{equation}

so
\begin{equation}\label{eqn:qftLecture9:220}
\begin{aligned}
\LL
&= \Abs{\partial \phi^\conj}^2 – \frac{\lambda}{4} \lr{ \Abs{\phi^\conj}^2 – v^2 }^2 \\
&=
\partial_\mu \rho \partial^\mu \rho + \partial_\mu \alpha \partial^\mu \alpha \lr{ 1 + \frac{\rho}{v} }
–
\frac{\lambda v^4}{4} \frac{ 4\rho^2}{v^2} + O(\rho^3) \\
&=
\partial_\mu \rho \partial^\mu \rho
– \lambda v^2\rho^2
+
\partial_\mu \alpha \partial^\mu \alpha \lr{ 1 + \frac{\rho}{v} }.
\end{aligned}
\end{equation}
We have two fields, \( \rho \) : a massive scalar field, the “Higgs”, and a massless field \( \alpha \) (the Goldstone Boson).

\( U(1) \) symmetry acts on \( \phi(x) \rightarrow e^{i \omega } \phi(x) \) i.t.o \( \alpha(x) \rightarrow \alpha(x) + v \omega \).
\( U(1) \) global symmetry (broken) acts on the Goldstone field \( \alpha(x) \) by a constant shift. (\(U(1)\) is still a symmetry of the Lagrangian.)

The current of the \( U(1) \) symmetry is:
\begin{equation}\label{eqn:qftLecture9:240}
j_\mu = \partial_\mu \alpha \lr{ 1 + \text{higher dimensional \( \rho \) terms} }.
\end{equation}

When we quantize
\begin{equation}\label{eqn:qftLecture9:260}
\alpha(x) =
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} e^{i \omega_p t – i \Bp \cdot \Bx} \hat{a}_\Bp^\dagger +
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} e^{-i \omega_p t + i \Bp \cdot \Bx} \hat{a}_\Bp
\end{equation}
\begin{equation}\label{eqn:qftLecture9:280}
j^\mu(x) = \partial^\mu \alpha(x) =
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} \lr{ i \omega_\Bp – i \Bp } e^{i \omega_p t – i \Bp \cdot \Bx} \hat{a}_\Bp^\dagger +
\int \frac{d^3p}{(2\pi)^3 \sqrt{ 2 \omega_p }} \lr{ -i \omega_\Bp + i \Bp } e^{-i \omega_p t + i \Bp \cdot \Bx} \hat{a}_\Bp.
\end{equation}

\begin{equation}\label{eqn:qftLecture9:300}
j^\mu(x) \ket{0} \ne 0,
\end{equation}
instead it creates a single particle state.

Examples of symmetries

In particle physics, examples of Wigner vs Nambu-Goldstone, ignoring gravity the only exact internal symmetry in the standard module is
\( (B\# – L\#) \), believed to be a \( U(1) \) symmetry in Wigner mode.

Here \(B\#\) is the Baryon number, and \( L\# \) is the Lepton number. Examples:

  • \( B(p) = 1 \), proton.
  • \( B(q) = 1/3 \), quark
  • \( B(e) = 1 \), electron
  • \( B(n) = 1 \), neutron.
  • \( L(p) = 1 \), proton.
  • \( L(q) = 0 \), quark.
  • \( L(e) = 0 \), electron.

The major use of global internal symmetries in the standard model is as “approximate” ones. They become symmetries when one neglects some effect( “terms in \( \LL \)”).
There are other approximate symmetries (use of group theory to find the Balmer series).

Example from HW2:

QCD in limit
\begin{equation}\label{eqn:qftLecture9:320}
m_u = m_d = 0.
\end{equation}
\( m_u m_d \ll m_p \) (the products of the up-quark mass and the down-quark mass are much less than a composite one (name?)).
\( SU(2)_L \times SU(2)_R \rightarrow SU(2)_V \)

EWSB (Electro-Weak-Symmetry-Breaking) sector

When the couplings \( g_2, g_1 = 0 \). (\( g_2 \in SU(2), g_1 \in U(1) \)).

Scale invariance

\begin{equation}\label{eqn:qftLecture9:340}
\begin{aligned}
x &\rightarrow e^{\lambda} x \\
\phi &\rightarrow e^{-\lambda} \phi \\
A_\mu &\rightarrow e^{-\lambda} A_\mu
\end{aligned}
\end{equation}
Any unitary theory which is scale invariant is also \underline{conformal} invariant. Conformal invariance means that angles are preserved.
The point here is that there is more than scale invariance.

We have classical internal global continuous symmetries.
These can be either

  1. “unbroken” (Wigner mode)
    \begin{equation}\label{eqn:qftLecture9:360}
    \hatQ\ket{0} = 0.
    \end{equation}
  2. “spontaneously broken”
    \begin{equation}\label{eqn:qftLecture9:380}
    j^\mu(x) \ket{0} \ne 0
    \end{equation}
    (creates Goldstone modes).
  3. “anomalous”. Classical symmetries are not a symmetry of QFT.
    Examples:

    • Scale symmetry (to be studied in QFT II), although this is not truly internal.
    • In QCD again when \( \omega_\Bq = 0 \), a \( U(1\) symmetry (chiral symmetry) becomes exact, and cannot be preserved in QFT.
    • In the standard model (E.W sector), the Baryon number and Lepton numbers are not symmetries, but their difference \( B\# – L\# \) is a symmetry.

Lorentz invariance.

We’d like to study the action of Lorentz symmetries on quantum states. We are going to “go by the book”, finding symmetries, currents, quantize, find generators, and so forth.

Under a Lorentz transformation
\begin{equation}\label{eqn:qftLecture9:400}
x^\mu \rightarrow {x’}^\mu = {\Lambda^\mu}_\nu x^\nu,
\end{equation}
We are going to consider infinitesimal Lorentz transformations
\begin{equation}\label{eqn:qftLecture9:420}
{\Lambda^\mu}_\nu \approx
{\delta^\mu}_\nu + {\omega^\mu}_\nu
,
\end{equation}
where \( {\omega^\mu}_\nu \) is small.
A Lorentz transformation \( \Lambda \) must satisfy \( \Lambda^\T G \Lambda = G \), or
\begin{equation}\label{eqn:qftLecture9:800}
g_{\mu\nu} = {{\Lambda}^\alpha}_\mu g_{\alpha \beta} {{\Lambda}^\beta}_\nu,
\end{equation}
into which we insert the infinitesimal transformation representation
\begin{equation}\label{eqn:qftLecture9:820}
\begin{aligned}
0
&=
– g_{\mu\nu} +
\lr{ {\delta^\alpha}_\mu + {\omega^\alpha}_\mu }
g_{\alpha \beta}
\lr{ {\delta^\beta}_\nu + {\omega^\beta}_\nu } \\
&=
– g_{\mu\nu} +
\lr{
g_{\mu \beta}
+
\omega_{\beta\mu}
}
\lr{ {\delta^\beta}_\nu + {\omega^\beta}_\nu } \\
&=
– g_{\mu\nu} +
g_{\mu \nu}
+
\omega_{\nu\mu}
+
\omega_{\mu\nu}
+
\omega_{\beta\mu}
{\omega^\beta}_\nu.
\end{aligned}
\end{equation}
The quadratic term can be ignored, leaving just
\begin{equation}\label{eqn:qftLecture9:840}
0 =
\omega_{\nu\mu}
+
\omega_{\mu\nu},
\end{equation}
or
\begin{equation}\label{eqn:qftLecture9:860}
\omega_{\nu\mu} = – \omega_{\mu\nu}.
\end{equation}
Note that \( \omega \) is a completely antisymmetric tensor, and like \( F_{\mu\nu} \) this has only 6 elements.
This means that the
infinitesimal transformation of the coordinates is
\begin{equation}\label{eqn:qftLecture9:440}
x^\mu \rightarrow {x’}^\mu \approx x^\mu + \omega^{\mu\nu} x_\nu,
\end{equation}
the field transforms as
\begin{equation}\label{eqn:qftLecture9:460}
\phi(x) \rightarrow \phi'(x’) = \phi(x)
\end{equation}
or
\begin{equation}\label{eqn:qftLecture9:760}
\phi'(x^\mu + \omega^{\mu\nu} x_\nu) =
\phi'(x) + \omega^{\mu\nu} x_\nu \partial_\mu\phi(x) = \phi(x),
\end{equation}
so
\begin{equation}\label{eqn:qftLecture9:480}
\delta \phi = \phi'(x) – \phi(x) =
-\omega^{\mu\nu} x_\nu \partial_\mu \phi.
\end{equation}

Since \( \LL \) is a scalar
\begin{equation}\label{eqn:qftLecture9:500}
\begin{aligned}
\delta \LL
&=
-\omega^{\mu\nu} x_\nu \partial_\mu \LL \\
&=
–
\partial_\mu \lr{
\omega^{\mu\nu} x_\nu \LL
}
+
(\partial_\mu x_\nu) \omega^{\mu\nu} \LL \\
&=
\partial_\mu \lr{
–
\omega^{\mu\nu} x_\nu \LL
},
\end{aligned}
\end{equation}
since \( \partial_\nu x_\mu = g_{\nu\mu} \) is symmetric, and \( \omega \) is antisymmetric.
Our current is
\begin{equation}\label{eqn:qftLecture9:520}
J^\mu_\omega
=
–
\omega^{\mu\nu} x_\mu \LL
.
\end{equation}
Our Noether current is
\begin{equation}\label{eqn:qftLecture9:540}
\begin{aligned}
j^\nu_{\omega^{\mu\rho}}
&= \PD{\phi_{,\nu}}{\LL} \delta \phi – J^\mu_\omega \\
&=
\partial^\nu \phi\lr{ – \omega^{\mu\rho} x_\rho \partial_\mu \phi } + \omega^{\nu \rho} x_\rho \LL \\
&=
\omega^{\mu\rho}
\lr{
\partial^\nu \phi\lr{ – x_\rho \partial_\mu \phi } + {\delta^{\nu}}_\mu x_\rho \LL
} \\
&=
\omega^{\mu\rho} x_\rho
\lr{
-\partial^\nu \phi \partial_\mu \phi + {\delta^{\nu}}_\mu \LL
}
\end{aligned}
\end{equation}
We identify
\begin{equation}\label{eqn:qftLecture9:560}
–
{T^\nu}_\mu =
-\partial^\nu \phi \partial_\mu \phi + {\delta^{\nu}}_\mu \LL,
\end{equation}
so the current is
\begin{equation}\label{eqn:qftLecture9:580}
\begin{aligned}
j^\nu_{\omega_{\mu\rho}}
&=
-\omega^{\mu\rho} x_\rho
{T^\nu}_\mu \\
&=
-\omega_{\mu\rho} x^\rho
T^{\nu\mu}
.
\end{aligned}
\end{equation}
Define
\begin{equation}\label{eqn:qftLecture9:600}
j^{\nu\mu\rho} = \inv{2} \lr{ x^\rho T^{\nu\mu} – x^{\mu} T^{\nu\rho} },
\end{equation}
which retains the antisymmetry in \( \mu \rho \) yet still drops the parameter \( \omega^{\mu\rho} \).
To check that this makes sense, we can contract
\( j^{\nu\mu\rho} \) with \( \omega_{\rho\mu} \)
\begin{equation}\label{eqn:qftLecture9:880}
\begin{aligned}
j^{\nu\mu\rho} \omega_{\rho\mu}
&= -\inv{2} \lr{ x^\rho T^{\nu\mu} – x^{\mu} T^{\nu\rho} }
\omega_{\mu\rho} \\
&=
-\inv{2} x^\rho T^{\nu\mu}
\omega_{\mu\rho}
– \inv{2} x^{\mu} T^{\nu\rho}
\omega_{\rho\mu} \\
&=
-\inv{2} x^\rho T^{\nu\mu}
\omega_{\mu\rho}
– \inv{2} x^{\rho} T^{\nu\mu}
\omega_{\mu\rho} \\
&=
– x^{\rho} T^{\nu\mu}
\omega_{\mu\rho},
\end{aligned}
\end{equation}
which matches \ref{eqn:qftLecture9:580} as desired.

Example. Rotations \( \mu\rho = ij \)

\begin{equation}\label{eqn:qftLecture9:620}
\begin{aligned}
J^{0 i j} \epsilon_{ijk}
&=
\inv{2} \lr{ x^i T^{0j} – x^{j} T^{0i} } \epsilon_{ijk} \\
&=
x^i T^{0j} \epsilon_{ijk}.
\end{aligned}
\end{equation}
Observe that this has the structure of \( (\Bx \cross \Bp)_k \), where \( \Bp \) is the momentum density of the field.
Let
\begin{equation}\label{eqn:qftLecture9:640}
L_k \equiv Q_k = \int d^3 x J^{0ij} \epsilon_{ijk}.
\end{equation}
We can now quantize and build a generator
\begin{equation}\label{eqn:qftLecture9:660}
\begin{aligned}
\hatU(\Balpha)
&= e^{i \Balpha \cdot \hat{\BL}} \\
&= \exp\lr{i \alpha_k
\int d^3 x x^i \hat{T}^{0j} \epsilon_{ijk}
}
\end{aligned}
\end{equation}
From \ref{eqn:qftLecture9:560} we can quantize with \( T^{0j} = \partial^0 \phi \partial^j \phi \rightarrow \hat{\pi} \lr{\spacegrad \phihat}_j\), or
\begin{equation}\label{eqn:qftLecture9:900}
\begin{aligned}
\hatU(\Balpha)
&=
\exp\lr{i \alpha_k
\int d^3 x x^i \hat{\pi} (\spacegrad \phihat)_j \epsilon_{ijk}
} \\
&=
\exp\lr{i \Balpha \cdot
\int d^3 x \hat{\pi} \spacegrad \phihat \cross \Bx
}
\end{aligned}
\end{equation}
(up to a sign in the exponent which doesn’t matter)
\begin{equation}\label{eqn:qftLecture9:680}
\begin{aligned}
\phihat(\By) \rightarrow \hatU(\alpha) \phihat(\By) \hatU^\dagger(\alpha)
&\approx
\phihat(\By) +
i \Balpha \cdot
\antisymmetric{
\int d^3 x \hat{\pi}(\Bx) \spacegrad \phihat(\Bx) \cross \Bx
}
{
\phihat(\By)
} \\
&=
\phihat(\By) +
i \Balpha \cdot
\int d^3 x
(-i) \delta^3(\Bx – \By)
\spacegrad \phihat(\Bx) \cross \Bx \\
&=
\phihat(\By) +
\Balpha \cdot \lr{ \spacegrad \phihat(\By ) \cross \By}
\end{aligned}
\end{equation}
Explicitly, in coordinates, this is
\begin{equation}\label{eqn:qftLecture9:700}
\begin{aligned}
\phihat(\By)
&\rightarrow
\phihat(\By) +
\alpha^i
\lr{
\partial^j \phihat(\By) y^k \epsilon_{jki}
} \\
&=
\phihat(\By) –
\epsilon_{ikj} \alpha^i y^k \partial^j \phihat \\
&=
\phihat( y^j – \epsilon^{ikj} \alpha^i y^k ).
\end{aligned}
\end{equation}
This is a rotation. To illustrate, pick \( \Balpha = (0, 0, \alpha) \), so \( y^j \rightarrow y^j – \epsilon^{ikj} \alpha y^k \delta_{i3} = y^j – \epsilon^{3kj} \alpha y^k \), or
\begin{equation}\label{eqn:qftLecture9:n}
\begin{aligned}
y^1 &\rightarrow y^1 – \epsilon^{3k1} \alpha y^k = y^1 + \alpha y^2 \\
y^2 &\rightarrow y^2 – \epsilon^{3k2} \alpha y^k = y^2 – \alpha y^1 \\
y^3 &\rightarrow y^3 – \epsilon^{3k3} \alpha y^k = y^3,
\end{aligned}
\end{equation}
or in matrix form
\begin{equation}\label{eqn:qftLecture9:720}
\begin{bmatrix}
y^1 \\
y^2 \\
y^3 \\
\end{bmatrix}
\rightarrow
\begin{bmatrix}
1 & \alpha & 0 \\
-\alpha & 1 & 0 \\
0 & 0 & 1
\end{bmatrix}
\begin{bmatrix}
y^1 \\
y^2 \\
y^3 \\
\end{bmatrix}.
\end{equation}

PHY2403H Quantum Field Theory. Lecture 7: Symmetries, translation currents, energy momentum tensor. Taught by Prof. Erich Poppitz

October 3, 2018 phy2403 , , , , , ,

[Click here for a PDF of this post with nicer formatting]

DISCLAIMER: Very rough notes from class, with some additional side notes.

These are notes for the UofT course PHY2403H, Quantum Field Theory I, taught by Prof. Erich Poppitz fall 2018.

Symmetries

Given the complexities of the non-linear systems we want to investigate, examination of symmetries gives us simpler problems that we can solve.

  • “internal” symmetries. This means that the symmetries do not act on space time \( (\Bx, t) \). An example is
    \begin{equation}\label{eqn:qftLecture7:20}
    \phi^i =
    \begin{bmatrix}
    \psi_1 \\
    \psi_2 \\
    \vdots \\
    \psi_N \\
    \end{bmatrix}
    \end{equation}
    If we map \( \phi^i \rightarrow O^i_j \phi^j \) where \( O^\T O = 1 \), then we call this an internal symmetry.
    The corresponding Lagrangian density might be something like
    \begin{equation}\label{eqn:qftLecture7:40}
    \LL = \inv{2} \partial_\mu \Bphi \cdot \partial^\mu \Bphi – \frac{m^2}{2} \Bphi \cdot \Bphi – V(\Bphi \cdot \Bphi)
    \end{equation}

  • spacetime symmetries: Translations, rotations, boosts, dilatations. We will consider continuous symmetries, which can be defined as a succession of infinitesimal transformations.
    An example from \(O(2)\) is a rotation
    \begin{equation}\label{eqn:qftLecture7:60}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2 \\
    \end{bmatrix}
    \rightarrow
    \begin{bmatrix}
    \cos\alpha & \sin\alpha \\
    -\sin\alpha & \cos\alpha \\
    \end{bmatrix}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2
    \end{bmatrix},
    \end{equation}
    or if \( \alpha \sim 0 \)
    \begin{equation}\label{eqn:qftLecture7:80}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2 \\
    \end{bmatrix}
    \rightarrow
    \begin{bmatrix}
    1 & \alpha \\
    -\alpha & 1\\
    \end{bmatrix}
    \begin{bmatrix}
    \phi^1 \\
    \phi^2
    \end{bmatrix}
    =
    \begin{bmatrix}
    \phi^1 \\
    \phi^2
    \end{bmatrix}
    +
    \alpha
    \begin{bmatrix}
    \phi^2 \\
    -\phi^1
    \end{bmatrix}
    \end{equation}
    In index notation we write
    \begin{equation}\label{eqn:qftLecture7:100}
    \phi^i \rightarrow \phi^i + \alpha e^{ij} \phi^j,
    \end{equation}
    where \( \epsilon^{12} = +1, \epsilon^{21} = -1 \) is the completely antisymmetric tensor. This can be written in more general form as
    \begin{equation}\label{eqn:qftLecture7:120}
    \phi^i \rightarrow \phi^i + \delta \phi^i,
    \end{equation}
    where \( \delta \phi^i \) is considered to be an infinitesimal transformation.

Definition: Symmetry

A symmetry means that there is some transformation
\begin{equation*}
\phi^i \rightarrow \phi^i + \delta \phi^i,
\end{equation*}
where \( \delta \phi^i \) is an infinitesimal transformation, and the equations of motion are invariant under this transformation.

Theorem: Noether’s theorem (1st).

If the equations of motion re invariant under \( \phi^\mu \rightarrow \phi^\mu + \delta \phi^\mu \), then there exists a conserved current \( j^\mu \) such that \( \partial_\mu j^\mu = 0 \).

Noether’s first theorem applies to global symmetries, where the parameters are the same for all \( (\Bx, t)\). Gauge symmetries are not examples of such global symmetries.

Given a Lagrangian density \( \LL(\phi(x), \phi_{,\mu}(x)) \), where \( \phi_{,\mu} \equiv \partial_\mu \phi \). The action is
\begin{equation}\label{eqn:qftLecture7:160}
S = \int d^d x \LL.
\end{equation}
EOMs are invariant if under \( \phi(x) \rightarrow \phi'(x) = \phi(x) + \delta_\epsilon \phi(x)\), we have
\begin{equation}\label{eqn:qftLecture7:180}
\LL(\phi) \rightarrow \LL'(\phi’) = \LL(\phi) + \partial_\mu J_\epsilon^\mu(\phi) + O(\epsilon^2).
\end{equation}
Then there exists a conserved current. In QFT we say that the E.O.M’s are “on shell”. Note that \ref{eqn:qftLecture7:180} is a symmetry since we have added a total derivative to the Lagrangian which leaves the equations of motion of unchanged.

In general, the change of action under arbitrary variation of \( \delta \phi\) of the fields is
\begin{equation}\label{eqn:qftLecture7:200}
\begin{aligned}
\delta S
&=
\int d^d x \delta \LL(\phi, \partial_\mu \phi) \\
&=
\int d^d x \lr{
\PD{\phi}{\LL} \delta \phi
+
\PD{(\partial_\mu \phi)}{\LL} \delta \partial_\mu \phi
} \\
&=
\int d^d x \lr{
\partial_\mu \lr{ \PD{(\partial_\mu \phi)}{\LL} } \delta \phi
+
\PD{(\partial_\mu \phi)}{\LL} \partial_\mu \delta \phi
} \\
&=
\int d^d x
\partial_\mu \lr{ \frac{\delta \LL}{\delta(\partial_\mu \phi)} \delta \phi }
\end{aligned}
\end{equation}
However from \ref{eqn:qftLecture7:180}
\begin{equation}\label{eqn:qftLecture7:220}
\delta_\epsilon \LL = \partial_\mu J_\epsilon^\mu(\phi, \partial_\mu \phi),
\end{equation}
so after equating these variations we fine that
\begin{equation}\label{eqn:qftLecture7:240}
\delta S = \int d^d x \delta_\epsilon \LL = \int d^d x \partial_\mu J_\epsilon^\mu,
\end{equation}
or
\begin{equation}\label{eqn:qftLecture7:260}
0 = \int d^d x
\partial_\mu \lr{ \frac{\delta \LL}{\delta(\partial_\mu \phi)} \delta \phi – J_\epsilon^\mu },
\end{equation}
or \( \partial_\mu j^\mu = 0 \) provided
\begin{equation}\label{eqn:qftLecture7:280}
\boxed{
j^\mu =
\frac{\delta \LL}{\delta(\partial_\mu \phi)} \delta_\epsilon \phi – J_\epsilon^\mu.
}
\end{equation}

Integrating the divergence of the current over a space time volume, perhaps that of cylinder (time up, space out) is also zero. That is
\begin{equation}\label{eqn:qftLecture7:300}
\begin{aligned}
0
&=
\int d^4 x \, \partial_\mu j^\mu \\
&=
\int d^3 \Bx dt \, \partial_\mu j^\mu \\
&=
\int d^3 \Bx dt \, \partial_t j^0 –
\int d^3 \Bx dt \spacegrad \cdot \Bj \\
&=
\int d^3 \Bx dt \, \partial_t j^0 ,
\end{aligned}
\end{equation}
where the spatial divergence is zero assuming there’s no current leaving the volume on the infinite boundary
(no \(\Bj\) at spatial infinity.)

We write
\begin{equation}\label{eqn:qftLecture7:560}
Q = \int d^3x \partial_t j^0,
\end{equation}
and call this the on-shell charge associated with the symmetry.

Spacetime translation.

A spacetime translation has the form
\begin{equation}\label{eqn:qftLecture7:320}
x^\mu \rightarrow {x’}^\mu = x^\mu + a^\mu,
\end{equation}
\begin{equation}\label{eqn:qftLecture7:340}
\phi(x) \rightarrow \phi'(x’) = \phi(x)
\end{equation}
(contrast this to a Lorentz transformation that had the form \( x^\mu \rightarrow {x’}^\mu = {\Lambda^\mu}_\nu x^\nu \)).

If \(\phi'(x + a) = \phi(x) \), then
\begin{equation}\label{eqn:qftLecture7:360}
\phi'(x) + a^\mu \partial_\mu \phi'(x) =
\phi'(x) + a^\mu \partial_\mu \phi(x) =
\phi(x),
\end{equation}
so
\begin{equation}\label{eqn:qftLecture7:380}
\phi'(x)
= \phi(x) – a^\mu \partial_\mu \phi'(x)
= \phi(x) + \delta_a \phi(x),
\end{equation}
or
\begin{equation}\label{eqn:qftLecture7:580}
\delta_a \phi(x) = – a^\mu \partial_\mu \phi(x).
\end{equation}
Under \( \phi \rightarrow \phi – a^\mu \partial_\mu \phi \), we have
\begin{equation}\label{eqn:qftLecture7:400}
\LL(\phi) \rightarrow \LL(\phi) – a^\mu \partial_\mu \LL.
\end{equation}
Let’s calculate this with our scalar theory Lagrangian
\begin{equation}\label{eqn:qftLecture7:420}
\LL = \inv{2} \partial_\mu \phi \partial^\mu \phi – \frac{m^2}{2} \phi^2 – V(\phi)
\end{equation}
The Lagrangian variation is
\begin{equation}\label{eqn:qftLecture7:440}
\begin{aligned}
\evalbar{\delta \LL}{\phi \rightarrow \phi + \delta \phi, \delta\phi = – a^\mu \partial_\mu \phi}
&=
(\partial_\mu \phi) \delta (\partial^\mu \phi) – m^2 \phi \delta \phi – \PD{\phi}{V} \delta \phi \\
&=
(\partial_\mu \phi)(-a^\nu \partial_\nu \phi \partial^\mu \phi) + m^2 \phi a^\nu \partial_\nu \phi + \PD{\phi}{V} a^\nu \partial_\nu \phi \\
&=
– a^\nu \partial_\nu \lr{ \inv{2} \partial_\mu \partial^\mu \phi – \frac{m^2}{2} \phi^2 – V(\phi) } \\
&=
– a^\nu \partial_\nu \LL.
\end{aligned}
\end{equation}

So the current is
\begin{equation}\label{eqn:qftLecture7:600}
\begin{aligned}
j^\mu
&=
(\partial^\mu \phi) (-a^\nu \partial_\nu \phi) + a^\nu \LL \\
&=
-a^\nu \lr{ \partial^\mu \phi \partial_\nu \phi – \LL }
\end{aligned}
\end{equation}

We really have a current for each \( \nu \) direction and can make that explicit writing
\begin{equation}\label{eqn:qftLecture7:460}
\begin{aligned}
\delta_\nu \LL
&= -\partial_\nu \LL \\
&= – \partial_\mu \lr{ {\delta^\mu}_\nu \LL } \\
&= \partial_\mu {J^\mu}_\nu
\end{aligned}
\end{equation}
we write
\begin{equation}\label{eqn:qftLecture7:480}
{j^\mu}_\nu = \PD{x_\mu}{\phi} \lr{ – \PD{x^\nu}{\phi} } + {\delta^\mu}_\nu \LL,
\end{equation}
where \( \nu \) are labels which coordinates are translated:
\begin{equation}\label{eqn:qftLecture7:500}
\begin{aligned}
\partial_\nu \phi &= – \partial_\nu \phi \\
\partial_\nu \LL &= – \partial_\nu \LL.
\end{aligned}
\end{equation}
We call the conserved quantities elements of the energy-momentum tensor, and write it as
\begin{equation}\label{eqn:qftLecture7:520}
\boxed{
{T^\mu}_\nu = -\PD{x_\mu}{\phi} \PD{x^\nu}{\phi} + {\delta^\mu}_\nu \LL.
}
\end{equation}

Incidentally, we picked a non-standard sign convention for the tensor, as an explicit expansion of \( T^{00} \), the energy density component, shows
\begin{equation}\label{eqn:qftLecture7:540}
\begin{aligned}
{T^0}_0
&=
-\PD{t}{\phi}
\PD{t}{\phi}
+\inv{2}
\PD{t}{\phi}
\PD{t}{\phi}
– \inv{2} (\spacegrad \phi) \cdot (\spacegrad \phi)
– \frac{m^2}{2} \phi^2 – V(\phi) \\
&=
-\inv{2} \PD{t}{\phi} \PD{t}{\phi}
– \inv{2} (\spacegrad \phi) \cdot (\spacegrad \phi)
– \frac{m^2}{2} \phi^2 – V(\phi).
\end{aligned}
\end{equation}
Had we translated by \( -a^\mu \) we’d have a positive definite tensor instead.