cylindrical coordinates

Angular momentum bivector in cylindrical and spherical bases.

September 15, 2022 math and physics play , , , , , , ,

[Click here for a PDF version of this post]

Motivation

In a discord thread on the bivector group (a geometric algebra group chat), MoneyKills posts about trouble he has calculating the correct expression for the angular momentum bivector or it’s dual.

This blog post is a more long winded answer than my bivector response and includes this calculation using both cylindrical and spherical coordinates.

Cylindrical coordinates.

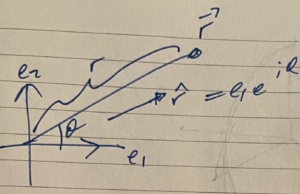
The position vector for any point on a plane can be expressed as
\begin{equation}\label{eqn:amomentum:20}
\Br = r \rcap,
\end{equation}
where \( \rcap = \rcap(\phi) \) encodes all the angular dependence of the position vector, and \( r \) is the length along that direction to our point, as illustrated in fig. 1.

fig. 1. Cylindrical coordinates position vector.

The radial unit vector has a compact GA representation
\begin{equation}\label{eqn:amomentum:40}
\rcap = \Be_1 e^{i\phi},
\end{equation}
where \( i = \Be_1 \Be_2 \).

The velocity (or momentum) will have both \( \rcap \) and \( \phicap \) dependence. By chain rule, that velocity is
\begin{equation}\label{eqn:amomentum:60}
\Bv = \dot{r} \rcap + r \dot{\rcap},
\end{equation}
where
\begin{equation}\label{eqn:amomentum:80}
\begin{aligned}
\dot{\rcap}
&= \Be_1 i e^{i\phi} \dot{\phi} \\
&= \Be_2 e^{i\phi} \dot{\phi} \\
&= \phicap \dot{\phi}.
\end{aligned}
\end{equation}
It is left to the reader to show that the vector designated \( \phicap \), is a unit vector and perpendicular to \( \rcap \) (Hint: compute the grade-0 selection of the product of the two to show that they are perpendicular.)

We can now compute the momentum, which is
\begin{equation}\label{eqn:amomentum:100}
\Bp = m \Bv = m \lr{ \dot{r} \rcap + r \dot{\phi} \phicap },
\end{equation}
and the angular momentum bivector
\begin{equation}\label{eqn:amomentum:120}
\begin{aligned}
L
&= \Br \wedge \Bp \\
&= m \lr{ r \rcap } \wedge \lr{ \dot{r} \rcap + r \dot{\phi} \phicap } \\
&= m r^2 \dot{\phi} \rcap \phicap.
\end{aligned}
\end{equation}

This has the \( m r^2 \dot{\phi} \) magnitude that the OP was seeking.

Spherical coordinates.

In spherical coordinates, our position vector is
\begin{equation}\label{eqn:amomentum:140}
\Br = r \lr{ \Be_1 \sin\theta \cos\phi + \Be_2 \sin\theta \sin\phi + \Be_3 \cos\theta },
\end{equation}
as sketched in fig. 2.

fig. 2. Spherical coordinates.

We can factor this into a more compact representation
\begin{equation}\label{eqn:amomentum:160}
\begin{aligned}
\Br
&= r \lr{ \sin\theta \Be_1 (\cos\phi + \Be_{12} \sin\phi ) + \Be_3 \cos\theta } \\
&= r \lr{ \sin\theta \Be_1 e^{\Be_{12} \phi } + \Be_3 \cos\theta } \\
&= r \Be_3 \lr{ \cos\theta + \sin\theta \Be_3 \Be_1 e^{\Be_{12} \phi } }.
\end{aligned}
\end{equation}

It is useful to name two of the bivector terms above, first, we write \( i \) for the azimuthal plane bivector sketched in fig. 3.

Spherical coordinates, azimuthal plane.

\begin{equation}\label{eqn:amomentum:180}
i = \Be_{12},
\end{equation}
and introduce a bivector \( j \) that encodes the \( \Be_3, \rcap \) plane as sketched in fig. 4.

Spherical coordinates, “j-plane”.

\begin{equation}\label{eqn:amomentum:200}
j = \Be_{31} e^{i \phi}.
\end{equation}

Having done so, we now have a compact representation for our position vector
\begin{equation}\label{eqn:amomentum:220}
\begin{aligned}
\Br
&= r \Be_3 \lr{ \cos\theta + j \sin\theta } \\
&= r \Be_3 e^{j \theta}.
\end{aligned}
\end{equation}

This provides us with a nice compact representation of the radial unit vector
\begin{equation}\label{eqn:amomentum:240}
\rcap = \Be_3 e^{j \theta}.
\end{equation}

Just as was the case in cylindrical coordinates, our azimuthal plane unit vector is
\begin{equation}\label{eqn:amomentum:280}
\phicap = \Be_2 e^{i\phi}.
\end{equation}

Now we want to compute the velocity vector. As was the case in cylindrical coordinates, we have
\begin{equation}\label{eqn:amomentum:300}
\Bv = \dot{r} \rcap + r \dot{\rcap},
\end{equation}
but now we need the spherical representation for the \( \rcap \) derivative, which is
\begin{equation}\label{eqn:amomentum:320}
\begin{aligned}
\dot{\rcap}
&=
\PD{\theta}{\rcap} \dot{\theta} + \PD{\phi}{\rcap} \dot{\phi} \\
&=
\Be_3 e^{j\theta} j \dot{\theta} + \Be_3 \sin\theta \PD{\phi}{j} \dot{\phi} \\
&=
\rcap j \dot{\theta} + \Be_3 \sin\theta j i \dot{\phi}.
\end{aligned}
\end{equation}
We can reduce the second multivector term without too much work
\begin{equation}\label{eqn:amomentum:340}
\begin{aligned}
\Be_3 j i
&=
\Be_3 \Be_{31} e^{i\phi} i \\
&=
\Be_3 \Be_{31} i e^{i\phi} \\
&=
\Be_{33112} e^{i\phi} \\
&=
\Be_{2} e^{i\phi} \\
&= \phicap,
\end{aligned}
\end{equation}
so we have
\begin{equation}\label{eqn:amomentum:360}
\dot{\rcap}
=
\rcap j \dot{\theta} + \sin\theta \phicap \dot{\phi}.
\end{equation}

The velocity is
\begin{equation}\label{eqn:amomentum:380}
\Bv = \dot{r} \rcap + r \lr{ \rcap j \dot{\theta} + \sin\theta \phicap \dot{\phi} }.
\end{equation}

Now we can finally compute the angular momentum bivector, which is
\begin{equation}\label{eqn:amomentum:400}
\begin{aligned}
L &=
\Br \wedge \Bp \\
&=
m r \rcap \wedge \lr{ \dot{r} \rcap + r \lr{ \rcap j \dot{\theta} + \sin\theta \phicap \dot{\phi} } } \\
&=
m r^2 \rcap \wedge \lr{ \rcap j \dot{\theta} + \sin\theta \phicap \dot{\phi} } \\
&=
m r^2 \gpgradetwo{ \rcap \lr{ \rcap j \dot{\theta} + \sin\theta \phicap \dot{\phi} } },
\end{aligned}
\end{equation}
which is just
\begin{equation}\label{eqn:amomentum:420}
L =
m r^2 \lr{ j \dot{\theta} + \sin\theta \rcap \phicap \dot{\phi} }.
\end{equation}

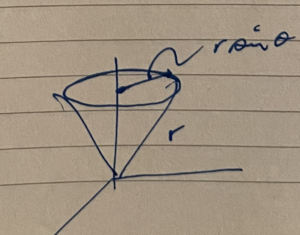
I was slightly surprised by this result, as I naively expected the cylindrical coordinate result. We have a \( m r^2 \rcap \phicap \dot{\phi} \) term, as was the case in cylindrical coordinates, but scaled down with a \( \sin\theta \) factor. However, this result does make sense. Consider for example, some fixed circular motion with \( \theta = \mathrm{constant} \), as sketched in fig. 5.

fig. 5. Circular motion for constant theta

The radius of this circle is actually \( r \sin\theta \), so the total angular momentum for that motion is scaled down to \( m r^2 \sin\theta \dot{\phi} \), smaller than the maximum circular angular momentum of \( m r^2 \dot{\phi} \) which occurs in the \( \theta = \pi/2 \) azimuthal plane. Similarly, if we have circular motion in the “j-plane”, sketched in fig. 6.

fig. 6. Circular motion for constant phi.

where \( \phi = \mathrm{constant} \), then our angular momentum is \( L = m r^2 j \dot{\theta} \).

Gradient, divergence, curl and Laplacian in cylindrical coordinates

November 6, 2016 math and physics play , , , , , , , , , , , ,

[Click here for a PDF of this post with nicer formatting]

In class it was suggested that the identity

\begin{equation}\label{eqn:laplacianCylindrical:20}
\spacegrad^2 \BA =
\spacegrad \lr{ \spacegrad \cdot \BA }
-\spacegrad \cross \lr{ \spacegrad \cross \BA },
\end{equation}

can be used to compute the Laplacian in non-rectangular coordinates. Is that the easiest way to do this?

How about just sequential applications of the gradient on the vector? Let’s start with the vector product of the gradient and the vector. First recall that the cylindrical representation of the gradient is

\begin{equation}\label{eqn:laplacianCylindrical:80}
\spacegrad = \rhocap \partial_\rho + \frac{\phicap}{\rho} \partial_\phi + \zcap \partial_z,
\end{equation}

where
\begin{equation}\label{eqn:laplacianCylindrical:100}
\begin{aligned}
\rhocap &= \Be_1 e^{\Be_1 \Be_2 \phi} \\
\phicap &= \Be_2 e^{\Be_1 \Be_2 \phi} \\
\end{aligned}
\end{equation}

Taking \( \phi \) derivatives of \ref{eqn:laplacianCylindrical:100}, we have

\begin{equation}\label{eqn:laplacianCylindrical:120}
\begin{aligned}
\partial_\phi \rhocap &= \Be_1 \Be_1 \Be_2 e^{\Be_1 \Be_2 \phi} = \Be_2 e^{\Be_1 \Be_2 \phi} = \phicap \\
\partial_\phi \phicap &= \Be_2 \Be_1 \Be_2 e^{\Be_1 \Be_2 \phi} = -\Be_1 e^{\Be_1 \Be_2 \phi} = -\rhocap.
\end{aligned}
\end{equation}

The gradient of a vector \( \BA = \rhocap A_\rho + \phicap A_\phi + \zcap A_z \) is

\begin{equation}\label{eqn:laplacianCylindrical:60}
\begin{aligned}
\spacegrad \BA
&=
\lr{ \rhocap \partial_\rho + \frac{\phicap}{\rho} \partial_\phi + \zcap \partial_z }
\lr{ \rhocap A_\rho + \phicap A_\phi + \zcap A_z } \\
&=
\quad \rhocap \partial_\rho \lr{ \rhocap A_\rho + \phicap A_\phi + \zcap A_z } \\
&\quad + \frac{\phicap}{\rho} \partial_\phi \lr{ \rhocap A_\rho + \phicap A_\phi + \zcap A_z } \\
&\quad + \zcap \partial_z \lr{ \rhocap A_\rho + \phicap A_\phi + \zcap A_z } \\
&=
\quad \rhocap \lr{ \rhocap \partial_\rho A_\rho + \phicap \partial_\rho A_\phi + \zcap \partial_\rho A_z } \\
&\quad + \frac{\phicap}{\rho} \lr{ \partial_\phi(\rhocap A_\rho) + \partial_\phi(\phicap A_\phi) + \zcap \partial_\phi A_z } \\
&\quad + \zcap \lr{ \rhocap \partial_z A_\rho + \phicap \partial_z A_\phi + \zcap \partial_z A_z } \\
&=
\quad \partial_\rho A_\rho + \rhocap \phicap \partial_\rho A_\phi + \rhocap \zcap \partial_\rho A_z \\
&\quad +\frac{1}{\rho} \lr{ A_\rho + \phicap \rhocap \partial_\phi A_\rho – \phicap \rhocap A_\phi + \partial_\phi A_\phi + \phicap \zcap \partial_\phi A_z } \\
&\quad + \zcap \rhocap \partial_z A_\rho + \zcap \phicap \partial_z A_\phi + \partial_z A_z \\
&=
\quad \partial_\rho A_\rho + \frac{1}{\rho} \lr{ A_\rho + \partial_\phi A_\phi } + \partial_z A_z \\
&\quad +
\zcap \rhocap \lr{
\partial_z A_\rho
-\partial_\rho A_z
} \\
&\quad +
\phicap \zcap \lr{
\inv{\rho} \partial_\phi A_z
– \partial_z A_\phi
} \\
&\quad +
\rhocap \phicap \lr{
\partial_\rho A_\phi
– \inv{\rho} \lr{ \partial_\phi A_\rho – A_\phi }
},
\end{aligned}
\end{equation}

As expected, we see that the gradient splits nicely into a dot and curl

\begin{equation}\label{eqn:laplacianCylindrical:160}
\begin{aligned}
\spacegrad \BA
&= \spacegrad \cdot \BA + \spacegrad \wedge \BA \\
&= \spacegrad \cdot \BA + \rhocap \phicap \zcap (\spacegrad \cross \BA ),
\end{aligned}
\end{equation}

where the cylindrical representation of the divergence is seen to be

\begin{equation}\label{eqn:laplacianCylindrical:140}
\spacegrad \cdot \BA
=
\inv{\rho} \partial_\rho (\rho A_\rho) + \frac{1}{\rho} \partial_\phi A_\phi + \partial_z A_z,
\end{equation}

and the cylindrical representation of the curl is

\begin{equation}\label{eqn:laplacianCylindrical:180}
\spacegrad \cross \BA
=
\rhocap
\lr{
\inv{\rho} \partial_\phi A_z
– \partial_z A_\phi
}
+
\phicap
\lr{
\partial_z A_\rho
-\partial_\rho A_z
}
+
\inv{\rho} \zcap \lr{
\partial_\rho ( \rho A_\phi )
– \partial_\phi A_\rho
}.
\end{equation}

Should we want to, it is now possible to evaluate the Laplacian of \( \BA \) using
\ref{eqn:laplacianCylindrical:20}
, which will have the following components

\begin{equation}\label{eqn:laplacianCylindrical:220}
\begin{aligned}
\rhocap \cdot \lr{ \spacegrad^2 \BA }
&=
\partial_\rho
\lr{
\inv{\rho} \partial_\rho (\rho A_\rho) + \frac{1}{\rho} \partial_\phi A_\phi + \partial_z A_z
}
–
\lr{
\inv{\rho} \partial_\phi \lr{
\inv{\rho} \lr{
\partial_\rho ( \rho A_\phi ) – \partial_\phi A_\rho
}
}
– \partial_z \lr{
\partial_z A_\rho -\partial_\rho A_z
}
} \\
&=
\partial_\rho \lr{ \inv{\rho} \partial_\rho (\rho A_\rho)}
+ \partial_\rho \lr{ \frac{1}{\rho} \partial_\phi A_\phi}
+ \partial_{\rho z} A_z
– \inv{\rho^2}\partial_{\phi \rho} ( \rho A_\phi )
+ \inv{\rho^2}\partial_{\phi\phi} A_\rho
+ \partial_{zz} A_\rho
– \partial_{z\rho} A_z \\
&=
\partial_\rho \lr{ \inv{\rho} \partial_\rho (\rho A_\rho)}
+ \inv{\rho^2}\partial_{\phi\phi} A_\rho
+ \partial_{zz} A_\rho
– \frac{1}{\rho^2} \partial_\phi A_\phi
+ \frac{1}{\rho} \partial_{\rho\phi} A_\phi
– \inv{\rho^2}\partial_{\phi} A_\phi
– \inv{\rho}\partial_{\phi\rho} A_\phi \\
&=
\partial_\rho \lr{ \inv{\rho} \partial_\rho (\rho A_\rho)}
+ \inv{\rho^2}\partial_{\phi\phi} A_\rho
+ \partial_{zz} A_\rho
– \frac{2}{\rho^2} \partial_\phi A_\phi \\
&=
\inv{\rho} \partial_\rho \lr{ \rho \partial_\rho A_\rho}
+ \inv{\rho^2}\partial_{\phi\phi} A_\rho
+ \partial_{zz} A_\rho
– \frac{A_\rho}{\rho^2}
– \frac{2}{\rho^2} \partial_\phi A_\phi,
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:laplacianCylindrical:240}
\begin{aligned}
\phicap \cdot \lr{ \spacegrad^2 \BA }
&=
\inv{\rho} \partial_\phi
\lr{
\inv{\rho} \partial_\rho (\rho A_\rho) + \frac{1}{\rho} \partial_\phi A_\phi + \partial_z A_z
}
–
\lr{
\lr{
\partial_z \lr{
\inv{\rho} \partial_\phi A_z – \partial_z A_\phi
}
-\partial_\rho \lr{
\inv{\rho} \lr{ \partial_\rho ( \rho A_\phi ) – \partial_\phi A_\rho}
}
}
} \\
&=
\inv{\rho^2} \partial_{\phi\rho} (\rho A_\rho)
+ \frac{1}{\rho^2} \partial_{\phi\phi} A_\phi
+ \inv{\rho}\partial_{\phi z} A_z
– \inv{\rho} \partial_{z\phi} A_z
+ \partial_{z z} A_\phi
+\partial_\rho \lr{ \inv{\rho} \partial_\rho ( \rho A_\phi ) }
– \partial_\rho \lr{ \inv{\rho} \partial_\phi A_\rho} \\
&=
\partial_\rho \lr{ \inv{\rho} \partial_\rho ( \rho A_\phi ) }
+ \frac{1}{\rho^2} \partial_{\phi\phi} A_\phi
+ \partial_{z z} A_\phi
+ \inv{\rho^2} \partial_{\phi\rho} (\rho A_\rho)
+ \inv{\rho}\partial_{\phi z} A_z
– \inv{\rho} \partial_{z\phi} A_z
– \partial_\rho \lr{ \inv{\rho} \partial_\phi A_\rho} \\
&=
\partial_\rho \lr{ \inv{\rho} \partial_\rho ( \rho A_\phi ) }
+ \frac{1}{\rho^2} \partial_{\phi\phi} A_\phi
+ \partial_{z z} A_\phi
+ \inv{\rho^2} \partial_{\phi} A_\rho
+ \inv{\rho} \partial_{\phi\rho} A_\rho
+ \inv{\rho^2} \partial_\phi A_\rho
– \inv{\rho} \partial_{\rho\phi} A_\rho \\
&=
\partial_\rho \lr{ \inv{\rho} \partial_\rho ( \rho A_\phi ) }
+ \frac{1}{\rho^2} \partial_{\phi\phi} A_\phi
+ \partial_{z z} A_\phi
+ \frac{2}{\rho^2} \partial_{\phi} A_\rho \\
&=
\inv{\rho} \partial_\rho \lr{ \rho \partial_\rho A_\phi }
+ \frac{1}{\rho^2} \partial_{\phi\phi} A_\phi
+ \partial_{z z} A_\phi
+ \frac{2}{\rho^2} \partial_{\phi} A_\rho
– \frac{A_\phi}{\rho^2},
\end{aligned}
\end{equation}

\begin{equation}\label{eqn:laplacianCylindrical:260}
\begin{aligned}
\zcap \cdot \lr{ \spacegrad^2 \BA }
&=
\partial_z
\lr{
\inv{\rho} \partial_\rho (\rho A_\rho) + \frac{1}{\rho} \partial_\phi A_\phi + \partial_z A_z
}
–
\inv{\rho} \lr{
\partial_\rho \lr{ \rho \lr{
\partial_z A_\rho -\partial_\rho A_z
}
}
– \partial_\phi \lr{
\inv{\rho} \partial_\phi A_z – \partial_z A_\phi
}
} \\
&=
\inv{\rho} \partial_{z\rho} (\rho A_\rho)
+ \frac{1}{\rho} \partial_{z\phi} A_\phi
+ \partial_{zz} A_z
– \inv{\rho}\partial_\rho \lr{ \rho \partial_z A_\rho }
+ \inv{\rho}\partial_\rho \lr{ \rho \partial_\rho A_z }
+ \inv{\rho^2} \partial_{\phi\phi} A_z
– \inv{\rho} \partial_{\phi z} A_\phi \\
&=
\inv{\rho}\partial_\rho \lr{ \rho \partial_\rho A_z }
+ \inv{\rho^2} \partial_{\phi\phi} A_z
+ \partial_{zz} A_z
+ \inv{\rho} \partial_{z} A_\rho
+\partial_{z\rho} A_\rho
+ \frac{1}{\rho} \partial_{z\phi} A_\phi
– \inv{\rho}\partial_z A_\rho
– \partial_{\rho z} A_\rho
– \inv{\rho} \partial_{\phi z} A_\phi \\
&=
\inv{\rho}\partial_\rho \lr{ \rho \partial_\rho A_z }
+ \inv{\rho^2} \partial_{\phi\phi} A_z
+ \partial_{zz} A_z
\end{aligned}
\end{equation}

Evaluating these was a fairly tedious and mechanical job, and would have been better suited to a computer algebra system than by hand as done here.

Explicit cylindrical Laplacian

Let’s try this a different way. The most obvious potential strategy is to just apply the Laplacian to the vector itself, but we need to include the unit vectors in such an operation

\begin{equation}\label{eqn:laplacianCylindrical:280}
\spacegrad^2 \BA =
\spacegrad^2 \lr{ \rhocap A_\rho + \phicap A_\phi + \zcap A_z }.
\end{equation}

First we need to know the explicit form of the cylindrical Laplacian. From the painful expansion, we can guess that it is

\begin{equation}\label{eqn:laplacianCylindrical:300}
\spacegrad^2 \psi
=
\inv{\rho}\partial_\rho \lr{ \rho \partial_\rho \psi }
+ \inv{\rho^2} \partial_{\phi\phi} \psi
+ \partial_{zz} \psi.
\end{equation}

Let’s check that explicitly. Here I use the vector product where \( \rhocap^2 = \phicap^2 = \zcap^2 = 1 \), and these vectors anticommute when different

\begin{equation}\label{eqn:laplacianCylindrical:320}
\begin{aligned}
\spacegrad^2 \psi
&=
\lr{ \rhocap \partial_\rho + \frac{\phicap}{\rho} \partial_\phi + \zcap \partial_z }
\lr{ \rhocap \partial_\rho \psi + \frac{\phicap}{\rho} \partial_\phi \psi + \zcap \partial_z \psi } \\
&=
\rhocap \partial_\rho
\lr{ \rhocap \partial_\rho \psi + \frac{\phicap}{\rho} \partial_\phi \psi + \zcap \partial_z \psi }
+ \frac{\phicap}{\rho} \partial_\phi
\lr{ \rhocap \partial_\rho \psi + \frac{\phicap}{\rho} \partial_\phi \psi + \zcap \partial_z \psi }
+ \zcap \partial_z
\lr{ \rhocap \partial_\rho \psi + \frac{\phicap}{\rho} \partial_\phi \psi + \zcap \partial_z \psi } \\
&=
\partial_{\rho\rho} \psi
+ \rhocap \phicap \partial_\rho \lr{ \frac{1}{\rho} \partial_\phi \psi}
+ \rhocap \zcap \partial_{\rho z} \psi
+ \frac{\phicap}{\rho} \partial_\phi \lr{ \rhocap \partial_\rho \psi }
+ \frac{\phicap}{\rho} \partial_\phi \lr{ \frac{\phicap}{\rho} \partial_\phi \psi }
+ \frac{\phicap \zcap }{\rho} \partial_{\phi z} \psi
+ \zcap \rhocap \partial_{z\rho} \psi
+ \frac{\zcap \phicap}{\rho} \partial_{z\phi} \psi
+ \partial_{zz} \psi \\
&=
\partial_{\rho\rho} \psi
+ \inv{\rho} \partial_\rho \psi
+ \frac{1}{\rho^2} \partial_{\phi \phi} \psi
+ \partial_{zz} \psi
+ \rhocap \phicap
\lr{
-\frac{1}{\rho^2} \partial_\phi \psi
+\frac{1}{\rho} \partial_{\rho \phi} \psi
-\inv{\rho} \partial_{\phi \rho} \psi
+ \frac{1}{\rho^2} \partial_\phi \psi
}
+ \zcap \rhocap \lr{
-\partial_{\rho z} \psi
+ \partial_{z\rho} \psi
}
+ \phicap \zcap \lr{
\inv{\rho} \partial_{\phi z} \psi
– \inv{\rho} \partial_{z\phi} \psi
} \\
&=
\partial_{\rho\rho} \psi
+ \inv{\rho} \partial_\rho \psi
+ \frac{1}{\rho^2} \partial_{\phi \phi} \psi
+ \partial_{zz} \psi,
\end{aligned}
\end{equation}

so the Laplacian operator is

\begin{equation}\label{eqn:laplacianCylindrical:340}
\boxed{
\spacegrad^2
=
\inv{\rho} \PD{\rho}{} \lr{ \rho \PD{\rho}{} }
+ \frac{1}{\rho^2} \PDSq{\phi}{}
+ \PDSq{z}{}.
}
\end{equation}

All the bivector grades of the Laplacian operator are seen to explicitly cancel, regardless of the grade of \( \psi \), just as if we had expanded the scalar Laplacian as a dot product
\( \spacegrad^2 \psi = \spacegrad \cdot \lr{ \spacegrad \psi} \).
Unlike such a scalar expansion, this derivation is seen to be valid for any grade \( \psi \). We know now that we can trust this result when \( \psi \) is a scalar, a vector, a bivector, a trivector, or even a multivector.

Vector Laplacian

Now that we trust that the typical scalar form of the Laplacian applies equally well to multivectors as it does to scalars, that cylindrical coordinate operator can now be applied to a
vector. Consider the projections onto each of the directions in turn

\begin{equation}\label{eqn:laplacianCylindrical:360}
\spacegrad^2 \lr{ \rhocap A_\rho }
=
\rhocap \inv{\rho} \partial_\rho \lr{ \rho \partial_\rho A_\rho }
+ \frac{1}{\rho^2} \partial_{\phi\phi} \lr{\rhocap A_\rho}
+ \rhocap \partial_{zz} A_\rho
\end{equation}

\begin{equation}\label{eqn:laplacianCylindrical:380}
\begin{aligned}
\partial_{\phi\phi} \lr{\rhocap A_\rho}
&=
\partial_\phi \lr{ \phicap A_\rho + \rhocap \partial_\phi A_\rho } \\
&=
-\rhocap A_\rho
+\phicap \partial_\phi A_\rho
+ \phicap \partial_\phi A_\rho
+ \rhocap \partial_{\phi\phi} A_\rho \\
&=
\rhocap \lr{ \partial_{\phi\phi} A_\rho -A_\rho }
+ 2 \phicap \partial_\phi A_\rho
\end{aligned}
\end{equation}

so this component of the vector Laplacian is

\begin{equation}\label{eqn:laplacianCylindrical:400}
\begin{aligned}
\spacegrad^2 \lr{ \rhocap A_\rho }
&=
\rhocap
\lr{
\inv{\rho} \partial_\rho \lr{ \rho \partial_\rho A_\rho }
+ \inv{\rho^2} \partial_{\phi\phi} A_\rho
– \inv{\rho^2} A_\rho
+ \partial_{zz} A_\rho
}
+
\phicap
\lr{
2 \inv{\rho^2} \partial_\phi A_\rho
} \\
&=
\rhocap \lr{
\spacegrad^2 A_\rho
– \inv{\rho^2} A_\rho
}
+
\phicap
\frac{2}{\rho^2} \partial_\phi A_\rho
.
\end{aligned}
\end{equation}

The Laplacian for the projection of the vector onto the \( \phicap \) direction is

\begin{equation}\label{eqn:laplacianCylindrical:420}
\spacegrad^2 \lr{ \phicap A_\phi }
=
\phicap \inv{\rho} \partial_\rho \lr{ \rho \partial_\rho A_\phi }
+ \frac{1}{\rho^2} \partial_{\phi\phi} \lr{\phicap A_\phi}
+ \phicap \partial_{zz} A_\phi,
\end{equation}

Again, since the unit vectors are \( \phi \) dependent, the \( \phi \) derivatives have to be treated carefully

\begin{equation}\label{eqn:laplacianCylindrical:440}
\begin{aligned}
\partial_{\phi\phi} \lr{\phicap A_\phi}
&=
\partial_{\phi} \lr{-\rhocap A_\phi + \phicap \partial_\phi A_\phi} \\
&=
-\phicap A_\phi
-\rhocap \partial_\phi A_\phi
– \rhocap \partial_\phi A_\phi
+ \phicap \partial_{\phi \phi} A_\phi \\
&=
– 2 \rhocap \partial_\phi A_\phi
+
\phicap
\lr{
\partial_{\phi \phi} A_\phi
– A_\phi
},
\end{aligned}
\end{equation}

so the Laplacian of this projection is
\begin{equation}\label{eqn:laplacianCylindrical:460}
\begin{aligned}
\spacegrad^2 \lr{ \phicap A_\phi }
&=
\phicap
\lr{
\inv{\rho} \partial_\rho \lr{ \rho \partial_\rho A_\phi }
+ \phicap \partial_{zz} A_\phi,
\inv{\rho^2} \partial_{\phi \phi} A_\phi
– \frac{A_\phi }{\rho^2}
}
– \rhocap \frac{2}{\rho^2} \partial_\phi A_\phi \\
&=
\phicap \lr{
\spacegrad^2 A_\phi
– \frac{A_\phi}{\rho^2}
}
– \rhocap \frac{2}{\rho^2} \partial_\phi A_\phi.
\end{aligned}
\end{equation}

Since \( \zcap \) is fixed we have

\begin{equation}\label{eqn:laplacianCylindrical:480}
\spacegrad^2 \zcap A_z
=
\zcap \spacegrad^2 A_z.
\end{equation}

Putting all the pieces together we have
\begin{equation}\label{eqn:laplacianCylindrical:500}
\boxed{
\spacegrad^2 \BA
=
\rhocap \lr{
\spacegrad^2 A_\rho
– \inv{\rho^2} A_\rho
– \frac{2}{\rho^2} \partial_\phi A_\phi
}
+\phicap \lr{
\spacegrad^2 A_\phi
– \frac{A_\phi}{\rho^2}
+ \frac{2}{\rho^2} \partial_\phi A_\rho
}
+
\zcap \spacegrad^2 A_z.
}
\end{equation}

This matches the results of \ref{eqn:laplacianCylindrical:220}, …, from the painful expansion of
\( \spacegrad \lr{ \spacegrad \cdot \BA } – \spacegrad \cross \lr{ \spacegrad \cross \BA } \).

Line charge field and potential.

October 26, 2016 math and physics play , , , , , , , , , ,

[Click here for a PDF of this post with nicer formatting]

When computing the most general solution of the electrostatic potential in a plane, Jackson [1] mentions that \( -2 \lambda_0 \ln \rho \) is the well known potential for an infinite line charge (up to the unit specific factor). Checking that statement, since I didn’t recall what that potential was offhand, I encountered some inconsistencies and non-convergent integrals, and thought it was worthwhile to explore those a bit more carefully. This will be done here.

Using Gauss’s law.

For an infinite length line charge, we can find the radial field contribution using Gauss’s law, imagining a cylinder of length \( \Delta l \) of radius \( \rho \) surrounding this charge with the midpoint at the origin. Ignoring any non-radial field contribution, we have

\begin{equation}\label{eqn:lineCharge:20}
\int_{-\Delta l/2}^{\Delta l/2} \ncap \cdot \BE (2 \pi \rho) dl = \frac{\lambda_0}{\epsilon_0} \Delta l,
\end{equation}

or

\begin{equation}\label{eqn:lineCharge:40}
\BE = \frac{\lambda_0}{2 \pi \epsilon_0} \frac{\rhocap}{\rho}.
\end{equation}

Since

\begin{equation}\label{eqn:lineCharge:60}
\frac{\rhocap}{\rho} = \spacegrad \ln \rho,
\end{equation}

this means that the potential is

\begin{equation}\label{eqn:lineCharge:80}
\phi = -\frac{2 \lambda_0}{4 \pi \epsilon_0} \ln \rho.
\end{equation}

Finite line charge potential.

Let’s try both these calculations for a finite charge distribution. Gauss’s law looses its usefulness, but we can evaluate the integrals directly. For the electric field

\begin{equation}\label{eqn:lineCharge:100}
\BE
= \frac{\lambda_0}{4 \pi \epsilon_0} \int \frac{(\Bx – \Bx’)}{\Abs{\Bx – \Bx’}^3} dl’.
\end{equation}

Using cylindrical coordinates with the field point \( \Bx = \rho \rhocap \) for convience, the charge point \( \Bx’ = z’ \zcap \), and a the charge distributed over \( [a,b] \) this is

\begin{equation}\label{eqn:lineCharge:120}
\BE
= \frac{\lambda_0}{4 \pi \epsilon_0} \int_a^b \frac{(\rho \rhocap – z’ \zcap)}{\lr{\rho^2 + (z’)^2}^{3/2}} dz’.
\end{equation}

When the charge is uniformly distributed around the origin \( [a,b] = b[-1,1] \) the \( \zcap \) component of this field is killed because the integrand is odd. This justifies ignoring such contributions in the Gaussing cylinder analysis above. The general solution to this integral is found to be

\begin{equation}\label{eqn:lineCharge:140}
\BE
=
\frac{\lambda_0}{4 \pi \epsilon_0}
\evalrange{
\lr{
\frac{z’ \rhocap }{\rho \sqrt{ \rho^2 + (z’)^2 } }
+\frac{\zcap}{ \sqrt{ \rho^2 + (z’)^2 } }
}
}{a}{b},
\end{equation}

or
\begin{equation}\label{eqn:lineCharge:240}
\boxed{
\BE
=
\frac{\lambda_0}{4 \pi \epsilon_0}
\lr{
\frac{\rhocap }{\rho}
\lr{
\frac{b}{\sqrt{ \rho^2 + b^2 } }
-\frac{a}{\sqrt{ \rho^2 + a^2 } }
}
+ \zcap
\lr{
\frac{1}{ \sqrt{ \rho^2 + b^2 } }
-\frac{1}{ \sqrt{ \rho^2 + a^2 } }
}
}.
}
\end{equation}

When \( b = -a = \Delta l/2 \), this reduces to

\begin{equation}\label{eqn:lineCharge:160}
\BE
=
\frac{\lambda_0}{4 \pi \epsilon_0}
\frac{\rhocap }{\rho}
\frac{\Delta l}{\sqrt{ \rho^2 + (\Delta l/2)^2 } },
\end{equation}

which further reduces to \ref{eqn:lineCharge:40} when \( \Delta l \gg \rho \).

Finite line charge potential. Wrong but illuminating.

Again, putting the field point at \( z’ = 0 \), we have

\begin{equation}\label{eqn:lineCharge:180}
\phi(\rho)
= \frac{\lambda_0}{4 \pi \epsilon_0} \int_a^b \frac{dz’}{\lr{\rho^2 + (z’)^2}^{1/2}},
\end{equation}

which integrates to
\begin{equation}\label{eqn:lineCharge:260}
\phi(\rho)
= \frac{\lambda_0}{4 \pi \epsilon_0 }
\ln \frac{ b + \sqrt{ \rho^2 + b^2 }}{ a + \sqrt{\rho^2 + a^2}}.
\end{equation}

With \( b = -a = \Delta l/2 \), this approaches

\begin{equation}\label{eqn:lineCharge:200}
\phi
\approx
\frac{\lambda_0}{4 \pi \epsilon_0 }
\ln \frac{ (\Delta l/2) }{ \rho^2/2\Abs{\Delta l/2}}
=
\frac{-2 \lambda_0}{4 \pi \epsilon_0 } \ln \rho
+
\frac{\lambda_0}{4 \pi \epsilon_0 }
\ln \lr{ (\Delta l)^2/2 }.
\end{equation}

Before \( \Delta l \) is allowed to tend to infinity, this is identical (up to a difference in the reference potential) to \ref{eqn:lineCharge:80} found using Gauss’s law. It is, strictly speaking, singular when \( \Delta l \rightarrow \infty \), so it does not seem right to infinity as a reference point for the potential.

There’s another weird thing about this result. Since this has no \( z \) dependence, it is not obvious how we would recover the non-radial portion of the electric field from this potential using \( \BE = -\spacegrad \phi \)? Let’s calculate the elecric field from \ref{eqn:lineCharge:180} explicitly

\begin{equation}\label{eqn:lineCharge:220}
\begin{aligned}
\BE
&=
-\frac{\lambda_0}{4 \pi \epsilon_0}
\spacegrad
\ln \frac{ b + \sqrt{ \rho^2 + b^2 }}{ a + \sqrt{\rho^2 + a^2}} \\
&=
-\frac{\lambda_0 \rhocap}{4 \pi \epsilon_0 }
\PD{\rho}{}
\ln \frac{ b + \sqrt{ \rho^2 + b^2 }}{ a + \sqrt{\rho^2 + a^2}} \\
&=
-\frac{\lambda_0 \rhocap}{4 \pi \epsilon_0}
\lr{
\inv{ b + \sqrt{ \rho^2 + b^2 }} \frac{ \rho }{\sqrt{ \rho^2 + b^2 }}
-\inv{ a + \sqrt{ \rho^2 + a^2 }} \frac{ \rho }{\sqrt{ \rho^2 + a^2 }}
} \\
&=
-\frac{\lambda_0 \rhocap}{4 \pi \epsilon_0 \rho}
\lr{
\frac{ -b + \sqrt{ \rho^2 + b^2 }}{\sqrt{ \rho^2 + b^2 }}
-\frac{ -a + \sqrt{ \rho^2 + a^2 }}{\sqrt{ \rho^2 + a^2 }}
} \\
&=
\frac{\lambda_0 \rhocap}{4 \pi \epsilon_0 \rho}
\lr{
\frac{ b }{\sqrt{ \rho^2 + b^2 }}
-\frac{ a }{\sqrt{ \rho^2 + a^2 }}
}.
\end{aligned}
\end{equation}

This recovers the radial component of the field from \ref{eqn:lineCharge:240}, but where did the \( \zcap \) component go? The required potential appears to be

\begin{equation}\label{eqn:lineCharge:340}
\phi(\rho, z)
=
\frac{\lambda_0}{4 \pi \epsilon_0 }
\ln \frac{ b + \sqrt{ \rho^2 + b^2 }}{ a + \sqrt{\rho^2 + a^2}}
–
\frac{z \lambda_0}{4 \pi \epsilon_0 }
\lr{ \frac{1}{\sqrt{\rho^2 + b^2}}
-\frac{1}{\sqrt{\rho^2 + a^2}}
}.
\end{equation}

When computing the electric field \( \BE(\rho, \theta, z) \), it was convienent to pick the coordinate system so that \( z = 0 \). Doing this with the potential gives the wrong answers. The reason for this appears to be that this kills the potential term that is linear in \( z \) before taking its gradient, and we need that term to have the \( \zcap \) field component that is expected for a charge distribution that is non-symmetric about the origin on the z-axis!

Finite line charge potential. Take II.

Let the point at which the potential is evaluated be

\begin{equation}\label{eqn:lineCharge:360}
\Bx = \rho \rhocap + z \zcap,
\end{equation}

and the charge point be
\begin{equation}\label{eqn:lineCharge:380}
\Bx’ = z’ \zcap.
\end{equation}

This gives

\begin{equation}\label{eqn:lineCharge:400}
\begin{aligned}
\phi(\rho, z)
&= \frac{\lambda_0}{4\pi \epsilon_0} \int_a^b \frac{dz’}{\Abs{\rho^2 + (z – z’)^2 }} \\
&= \frac{\lambda_0}{4\pi \epsilon_0} \int_{a-z}^{b-z} \frac{du}{ \Abs{\rho^2 + u^2} } \\
&= \frac{\lambda_0}{4\pi \epsilon_0}
\evalrange{\ln \lr{ u + \sqrt{ \rho^2 + u^2 }}}{b-z}{a-z} \\
&=
\frac{\lambda_0}{4\pi \epsilon_0}
\ln \frac
{ b-z + \sqrt{ \rho^2 + (b-z)^2 }}
{ a-z + \sqrt{ \rho^2 + (a-z)^2 }}.
\end{aligned}
\end{equation}

The limit of this potential \( a = -\Delta/2 \rightarrow -\infty, b = \Delta/2 \rightarrow \infty \) doesn’t exist in any strict sense. If we are cavilier about the limits, as in \ref{eqn:lineCharge:200}, this can be evaluated as

\begin{equation}\label{eqn:lineCharge:n}
\phi \approx
\frac{\lambda_0}{4\pi \epsilon_0} \lr{ -2 \ln \rho + \textrm{constant} }.
\end{equation}

however, the constant (\( \ln \Delta^2/2 \)) is infinite, so there isn’t really a good justification for using that constant as the potential reference point directly.

It seems that the “right” way to calculate the potential for the infinite distribution, is to

  • Calculate the field from the potential.
  • Take the PV limit of that field with the charge distribution extending to infinity.
  • Compute the corresponding potential from this limiting value of the field.

Doing that doesn’t blow up. That field calculation, for the finite case, should include a \( \zcap \) component. To verify, let’s take the respective derivatives

\begin{equation}\label{eqn:lineCharge:420}
\begin{aligned}
-\PD{z}{} \phi
&=
-\frac{\lambda_0}{4\pi \epsilon_0}
\lr{
\frac{ -1 + \frac{z – b}{\sqrt{ \rho^2 + (b-z)^2 }} }{
b-z + \sqrt{ \rho^2 + (b-z)^2 }
}
–
\frac{ -1 + \frac{z – a}{\sqrt{ \rho^2 + (a-z)^2 }} }{
a-z + \sqrt{ \rho^2 + (a-z)^2 }
}
} \\
&=
\frac{\lambda_0}{4\pi \epsilon_0}
\lr{
\frac{ 1 + \frac{b – z}{\sqrt{ \rho^2 + (b-z)^2 }} }{
b-z + \sqrt{ \rho^2 + (b-z)^2 }
}
–
\frac{ 1 + \frac{a – z}{\sqrt{ \rho^2 + (a-z)^2 }} }{
a-z + \sqrt{ \rho^2 + (a-z)^2 }
}
} \\
&=
\frac{\lambda_0}{4\pi \epsilon_0}
\lr{
\inv{\sqrt{ \rho^2 + (b-z)^2 }}
-\inv{\sqrt{ \rho^2 + (a-z)^2 }}
},
\end{aligned}
\end{equation}

and

\begin{equation}\label{eqn:lineCharge:440}
\begin{aligned}
-\PD{\rho}{} \phi
&=
-\frac{\lambda_0}{4\pi \epsilon_0}
\lr{
\frac{ \frac{\rho}{\sqrt{ \rho^2 + (b-z)^2 }} }{
b-z + \sqrt{ \rho^2 + (b-z)^2 }
}
–
\frac{ \frac{\rho}{\sqrt{ \rho^2 + (a-z)^2 }} }{
a-z + \sqrt{ \rho^2 + (a-z)^2 }
}
} \\
&=
-\frac{\lambda_0}{4\pi \epsilon_0}
\lr{
\frac{\rho \lr{
-(b-z) + \sqrt{ \rho^2 + (b-z)^2 }
}}{ \rho^2 \sqrt{ \rho^2 + (b-z)^2 } }
–
\frac{\rho \lr{
-(a-z) + \sqrt{ \rho^2 + (a-z)^2 }
}}{ \rho^2 \sqrt{ \rho^2 + (a-z)^2 } }
} \\
&=
\frac{\lambda_0}{4\pi \epsilon_0 \rho}
\lr{
\frac{b-z}{\sqrt{ \rho^2 + (b-z)^2 }}
-\frac{a-z}{\sqrt{ \rho^2 + (a-z)^2 }}
}
.
\end{aligned}
\end{equation}

Putting the pieces together, the electric field is
\begin{equation}\label{eqn:lineCharge:460}
\BE =
\frac{\lambda_0}{4\pi \epsilon_0}
\lr{
\frac{\rhocap}{\rho} \lr{
\frac{b-z}{\sqrt{ \rho^2 + (b-z)^2 }}
-\frac{a-z}{\sqrt{ \rho^2 + (a-z)^2 }}
}
+
\zcap \lr{
\inv{\sqrt{ \rho^2 + (b-z)^2 }}
-\inv{\sqrt{ \rho^2 + (a-z)^2 }}
}
}.
\end{equation}

For has a PV limit of \ref{eqn:lineCharge:40} at \( z = 0 \), and also for the finite case, has the \( \zcap \) field component that was obtained when the field was obtained by direct integration.

Conclusions

  • We have to evaluate the potential at all points in space, not just on the axis that we evaluate the field on (should we choose to do so).
  • In this case, we found that it was not directly meaningful to take the limit of a potential distribution. We can, however, compute the field from a potential for a finite charge distribution,
    take the limit of that field, and then calculate the corresponding potential for the infinite distribution.

Is there a more robust mechanism that can be used to directly calculate the potential for an infinite charge distribution, instead of calculating the potential from the field of such an infinite distribution?

I think that were things go wrong is that the integral of \ref{eqn:lineCharge:180} does not apply to charge distributions that are not finite on the infinite range \( z \in [-\infty, \infty] \). That solution was obtained by utilizing an all-space Green’s function, and the boundary term in that Green’s analysis was assumed to tend to zero. That isn’t the case when the charge distribution is \( \lambda_0 \delta( z ) \).

References

[1] JD Jackson. Classical Electrodynamics. John Wiley and Sons, 2nd edition, 1975.

Coupled wave equation in cylindrical coordinates

May 5, 2015 math and physics play , ,

[Click here for a PDF of this post with nicer formatting]

In [1], for a sourceless configuration, it is noted that the electric field equations \( \spacegrad^2 \BE = -\beta^2 \BE \) have the form

\begin{equation}\label{eqn:cylindricalFieldSolution:20}
\spacegrad^2 E_\rho – \frac{E_\rho}{\rho^2} – \frac{2}{\rho^2} \PD{\phi}{E_\phi} = -\beta^2 E_\rho
\end{equation}
\begin{equation}\label{eqn:cylindricalFieldSolution:60}
\spacegrad^2 E_\phi – \frac{E_\phi}{\rho^2} + \frac{2}{\rho^2} \PD{\phi}{E_\rho} = -\beta^2 E_\phi
\end{equation}
\begin{equation}\label{eqn:cylindricalFieldSolution:80}
\spacegrad^2 E_z = -\beta^2 E_z,
\end{equation}

where

\begin{equation}\label{eqn:cylindricalFieldSolution:100}
\spacegrad^2 \psi =
\inv{\rho} \PD{\rho}{} \lr{ \rho \PD{\rho}{\psi}} + \inv{\rho^2}\PDSq{\phi}{\psi} + \PDSq{z}{\psi}.
\end{equation}

He applies separation of variables to the last equation, ending up with the usual Bessel function solution, but the first two coupled equations are dismissed as coupled and difficult. It looks like separation of variables works for this too, but we have to prep the system slightly by writing \( \psi = E_\rho + j E_\phi \), which gives

\begin{equation}\label{eqn:cylindricalFieldSolution:120}
\spacegrad^2 \psi – \frac{\psi}{\rho^2} + \frac{2 j}{\rho^2} \PD{\phi}{\psi} = -\beta^2 \psi,
\end{equation}

or

\begin{equation}\label{eqn:cylindricalFieldSolution:140}
\inv{\rho} \PD{\rho}{} \lr{ \rho \PD{\rho}{\psi}} + \inv{\rho^2}\PDSq{\phi}{\psi} + \PDSq{z}{\psi}
– \frac{\psi}{\rho^2} + \frac{2 j}{\rho^2} \PD{\phi}{\psi} = -\beta^2 \psi.
\end{equation}

With a separation of variables substitution \( \psi = f(\rho) g(\phi) h(z) \) this gives

\begin{equation}\label{eqn:cylindricalFieldSolution:160}
\inv{\rho f} \PD{\rho}{} \lr{ \rho \PD{\rho}{f}}
+ \inv{\rho^2 g}\PDSq{\phi}{g}
+ \inv{z} \PDSq{z}{h}
– \frac{1}{\rho^2} + \frac{2 j}{\rho^2 g} \PD{\phi}{g} = -\beta^2.
\end{equation}

Assuming a solution for the function \( h \) of

\begin{equation}\label{eqn:cylindricalFieldSolution:180}
\inv{z} \PDSq{z}{h} = -\alpha^2,
\end{equation}

the PDE is reduced to an equation in two functions

\begin{equation}\label{eqn:cylindricalFieldSolution:200}
\inv{\rho f} \PD{\rho}{} \lr{ \rho \PD{\rho}{f}}
+ \inv{\rho^2 g}\PD{\phi}{} \lr{ g + 2 j g}
+ \beta^2 -\alpha^2
– \frac{1}{\rho^2}
= 0,
\end{equation}

or

\begin{equation}\label{eqn:cylindricalFieldSolution:220}
\frac{\rho}{f} \PD{\rho}{} \lr{ \rho \PD{\rho}{f}}
+ \inv{g}\PD{\phi}{} \lr{ g + 2 j g}
+ \lr{ \beta^2 -\alpha^2 }\rho^2
= 1.
\end{equation}

With the term in \( g \) having only \( \phi \) dependence, we can assume

\begin{equation}\label{eqn:cylindricalFieldSolution:240}
\inv{g}\PD{\phi}{} \lr{ g + 2 j g} = 1 – \gamma^2,
\end{equation}

for

\begin{equation}\label{eqn:cylindricalFieldSolution:260}
\frac{\rho}{f} \PD{\rho}{} \lr{ \rho \PD{\rho}{f}}
– \gamma^2
+ \lr{ \beta^2 -\alpha^2 }\rho^2
= 0.
\end{equation}

I’m not sure off hand if these can be solved in known special functions, especially since the constants in the mix are complex.

References

[1] Constantine A Balanis. Advanced engineering electromagnetics, volume 20. Wiley New York, 1989.