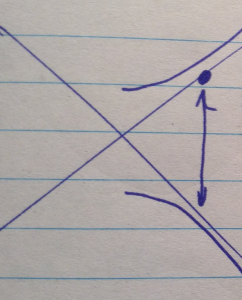continuity equation

PHY1520H Graduate Quantum Mechanics. Lecture 9: Dirac equation (cont.). Taught by Prof. Arun Paramekanti

October 15, 2015 phy1520 , , , , , , , ,

[Click here for a PDF of this post with nicer formatting]

Disclaimer

Peeter’s lecture notes from class. These may be incoherent and rough.

These are notes for the UofT course PHY1520, Graduate Quantum Mechanics, taught by Prof. Paramekanti.

Where we left off

\begin{equation}\label{eqn:qmLecture9:20}
-i \Hbar \PD{t}{}
\begin{bmatrix}
\psi_1 \\
\psi_2
\end{bmatrix}
=
\begin{bmatrix}
-i \Hbar c \PD{x}{} & m c^2 \\
m c^2 & i \Hbar c \PD{x}{} \\
\end{bmatrix}.
\end{equation}

With a potential this would be

\begin{equation}\label{eqn:qmLecture9:40}
-i \Hbar \PD{t}{}
\begin{bmatrix}
\psi_1 \\
\psi_2
\end{bmatrix}
=
\begin{bmatrix}
-i \Hbar c \PD{x}{} + V(x) & m c^2 \\
m c^2 & i \Hbar c \PD{x}{} + V(x) \\
\end{bmatrix}.
\end{equation}

This means that the potential is raising the energy eigenvalue of the system.

Free Particle

Assuming a form

\begin{equation}\label{eqn:qmLecture9:60}
\begin{bmatrix}
\psi_1(x,t) \\
\psi_2(x,t)
\end{bmatrix}
=
e^{i k x}
\begin{bmatrix}
f_1(t) \\
f_2(t) \\
\end{bmatrix},
\end{equation}

and plugging back into the Dirac equation we have

\begin{equation}\label{eqn:qmLecture9:80}
-i \Hbar \PD{t}{}
\begin{bmatrix}
f_1 \\
f_2
\end{bmatrix}
=
\begin{bmatrix}
k \Hbar c & m c^2 \\
m c^2 & – \Hbar k c \\
\end{bmatrix}
\begin{bmatrix}
f_1 \\
f_2
\end{bmatrix}.
\end{equation}

We can use a diagonalizing rotation

\begin{equation}\label{eqn:qmLecture9:100}
\begin{bmatrix}
f_1 \\
f_2
\end{bmatrix}
=
\begin{bmatrix}
\cos\theta_k & -\sin\theta_k \\
\sin\theta_k & \cos\theta_k \\
\end{bmatrix}
\begin{bmatrix}
f_{+} \\
f_{-} \\
\end{bmatrix}.
\end{equation}

Plugging this in reduces the system to the form

\begin{equation}\label{eqn:qmLecture9:140}
-i \Hbar \PD{t}{}
\begin{bmatrix}
f_{+} \\
f_{-} \\
\end{bmatrix}
=
\begin{bmatrix}
E_k & 0 \\
0 & -E_k
\end{bmatrix}
\begin{bmatrix}
f_{+} \\
f_{-} \\
\end{bmatrix}.
\end{equation}

Where the rotation angle is found to be given by

\begin{equation}\label{eqn:qmLecture9:160}
\begin{aligned}
\sin(2 \theta_k) &= \frac{m c^2}{\sqrt{(\Hbar k c)^2 + m^2 c^4}} \\
\cos(2 \theta_k) &= \frac{\Hbar k c}{\sqrt{(\Hbar k c)^2 + m^2 c^4}} \\
E_k &= \sqrt{(\Hbar k c)^2 + m^2 c^4}
\end{aligned}
\end{equation}

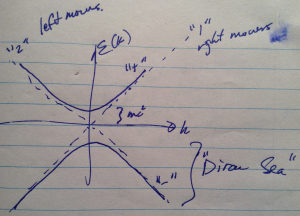
See fig. 1 for a sketch of energy vs momentum. The asymptotes are the limiting cases when \( m c^2 \rightarrow 0 \). The \( + \) branch is what we usually associate with particles. What about the other energy states. For Fermions Dirac argued that the lower energy states could be thought of as “filled up”, using the Pauli principle to leave only the positive energy states available. This was called the “Dirac Sea”. This isn’t a good solution, and won’t work for example for Bosons.

fig. 1. Dirac equation solution space

fig. 1. Dirac equation solution space

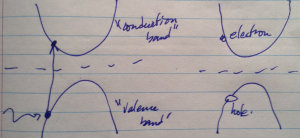
Another way to rationalize this is to employ ideas from solid state theory. For example consider a semiconductor with a valence and conduction band as sketched in fig. 2.

fig. 2. Solid state valence and conduction band transition

fig. 2. Solid state valence and conduction band transition

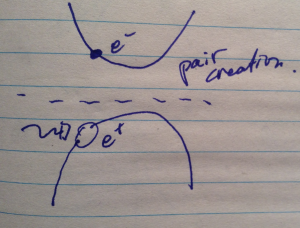
A photon can excite an electron from the valence band to the conduction band, leaving all the valence band states filled except for one (a hole). For an electron we can use almost the same picture, as sketched in fig. 3.

fig. 3. Pair creation

fig. 3. Pair creation

A photon with energy \( E_k – (-E_k) \) can create a positron-electron pair from the vacuum, where the energy of the electron and positron pair is \( E_k \).

At high enough energies, we can see this pair creation occur.

Zitterbewegung

If a particle is created at a non-eigenstate such as one on the asymptotes, then oscillations between the positive and negative branches are possible as sketched in fig. 4.

fig. 4. Zitterbewegung oscillation

fig. 4. Zitterbewegung oscillation

Only “vertical” oscillations between the positive and negative locations on these branches is possible since those are the points that match the particle momentum. Examining this will be the aim of one of the problem set problems.

Probability and current density

If we define a probability density

\begin{equation}\label{eqn:qmLecture9:180}
\rho(x, t) = \Abs{\psi_1}^2 + \Abs{\psi_2}^2,
\end{equation}

does this satisfy a probability conservation relation

\begin{equation}\label{eqn:qmLecture9:200}
\PD{t}{\rho} + \PD{x}{j} = 0,
\end{equation}

where \( j \) is the probability current. Plugging in the density, we have

\begin{equation}\label{eqn:qmLecture9:220}
\PD{t}{\rho}
=
\PD{t}{\psi_1^\conj} \psi_1
+
\psi_1^\conj \PD{t}{\psi_1}
+
\PD{t}{\psi_2^\conj} \psi_2
+
\psi_2^\conj \PD{t}{\psi_2}.
\end{equation}

It turns out that the probability current has the form

\begin{equation}\label{eqn:qmLecture9:240}
j(x,t) = c \lr{ \psi_1^\conj \psi_1 + \psi_2^\conj \psi_2 }.
\end{equation}

Here the speed of light \( c \) is the slope of the line in the plots above. We can think of this current density as right movers minus the left movers. Any state that is given can be thought of as a combination of right moving and left moving states, neither of which are eigenstates of the free particle Hamiltonian.

Potential step

The next logical thing to think about, as in non-relativistic quantum mechanics, is to think about what occurs when the particle hits a potential step, as in fig. 5.

fig. 5. Reflection off a potential barrier

fig. 5. Reflection off a potential barrier

The approach is the same. We write down the wave functions for the \( V = 0 \) region (I), and the higher potential region (II).

The eigenstates are found on the solid lines above the asymptotes on the right hand movers side as sketched in fig. 6. The right and left moving designations are based on the phase velocity \( \PDi{k}{E} \) (approaching \( \pm c \) on the top-right and top-left quadrants respectively).

fig. 6. Right movers and left movers

fig. 6. Right movers and left movers

For \( k > 0 \), an eigenstate for the incident wave is

\begin{equation}\label{eqn:qmLecture9:261}
\Bpsi_{\textrm{inc}}(x) =
\begin{bmatrix}
\cos\theta_k \\
\sin\theta_k
\end{bmatrix}
e^{i k x},
\end{equation}

For the reflected wave function, we pick a function on the left moving side of the positive energy branch.

\begin{equation}\label{eqn:qmLecture9:260}
\Bpsi_{\textrm{ref}}(x) =
\begin{bmatrix}
? \\
?
\end{bmatrix}
e^{-i k x},
\end{equation}

We’ll go through this in more detail next time.

Question: Calculate the right going diagonalization

Prove (7).

Answer

To determine the relations for \( \theta_k \) we have to solve

\begin{equation}\label{eqn:qmLecture9:280}
\begin{bmatrix}
E_k & 0 \\
0 & -E_k
\end{bmatrix}
= R^{-1} H R.
\end{equation}

Working with \( \Hbar = c = 1 \) temporarily, and \( C = \cos\theta_k, S = \sin\theta_k \), that is

\begin{equation}\label{eqn:qmLecture9:300}
\begin{aligned}
\begin{bmatrix}
E_k & 0 \\
0 & -E_k
\end{bmatrix}
&=
\begin{bmatrix}
C & S \\
-S & C
\end{bmatrix}
\begin{bmatrix}
k & m \\
m & -k
\end{bmatrix}
\begin{bmatrix}
C & -S \\
S & C
\end{bmatrix} \\
&=
\begin{bmatrix}
C & S \\
-S & C
\end{bmatrix}
\begin{bmatrix}
k C + m S & -k S + m C \\
m C – k S & -m S – k C
\end{bmatrix} \\
&=
\begin{bmatrix}
k C^2 + m S C + m C S – k S^2 & -k S C + m C^2 -m S^2 – k C S \\
-k C S – m S^2 + m C^2 – k S C & k S^2 – m C S -m S C – k C^2
\end{bmatrix} \\
&=
\begin{bmatrix}
k \cos(2 \theta_k) + m \sin(2 \theta_k) & m \cos(2 \theta_k) – k \sin(2 \theta_k) \\
m \cos(2 \theta_k) – k \sin(2 \theta_k) & -k \cos(2 \theta_k) – m \sin(2 \theta_k) \\
\end{bmatrix}.
\end{aligned}
\end{equation}

This gives

\begin{equation}\label{eqn:qmLecture9:320}
\begin{aligned}
E_k
\begin{bmatrix}
1 \\
0
\end{bmatrix}
&=
\begin{bmatrix}
k \cos(2 \theta_k) + m \sin(2 \theta_k) \\
m \cos(2 \theta_k) – k \sin(2 \theta_k) \\
\end{bmatrix} \\
&=
\begin{bmatrix}
k & m \\
m & -k
\end{bmatrix}
\begin{bmatrix}
\cos(2 \theta_k) \\
\sin(2 \theta_k) \\
\end{bmatrix}.
\end{aligned}
\end{equation}

Adding back in the \(\Hbar\)’s and \(c\)’s this is

\begin{equation}\label{eqn:qmLecture9:340}
\begin{aligned}
\begin{bmatrix}
\cos(2 \theta_k) \\
\sin(2 \theta_k) \\
\end{bmatrix}
&=
\frac{E_k}{-(\Hbar k c)^2 -(m c^2)^2}
\begin{bmatrix}
– \Hbar k c & – m c^2 \\
– m c^2 & \Hbar k c
\end{bmatrix}
\begin{bmatrix}
1 \\
0
\end{bmatrix} \\
&=
\inv{E_k}
\begin{bmatrix}
\Hbar k c \\
m c^2
\end{bmatrix}.
\end{aligned}
\end{equation}

Question: Verify the Dirac current relationship.

Prove \ref{eqn:qmLecture9:240}.

Answer

The components of the Schrodinger equation are

\begin{equation}\label{eqn:qmLecture9:360}
\begin{aligned}
-i \Hbar \PD{t}{\psi_1} &= -i \Hbar c \PD{x}{\psi_1} + m c^2 \psi_2 \\
-i \Hbar \PD{t}{\psi_2} &= m c^2 \psi_1 + i \Hbar c \PD{x}{\psi_2},
\end{aligned}
\end{equation}

The conjugates of these are
\begin{equation}\label{eqn:qmLecture9:380}
\begin{aligned}
i \Hbar \PD{t}{\psi_1^\conj} &= i \Hbar c \PD{x}{\psi_1^\conj} + m c^2 \psi_2^\conj \\
i \Hbar \PD{t}{\psi_2^\conj} &= m c^2 \psi_1^\conj – i \Hbar c \PD{x}{\psi_2^\conj}.
\end{aligned}
\end{equation}

This gives
\begin{equation}\label{eqn:qmLecture9:400}
\begin{aligned}
i \Hbar \PD{t}{\rho}
&=
\lr{ i \Hbar c \PD{x}{\psi_1^\conj} + m c^2 \psi_2^\conj } \psi_1 \\
&+ \psi_1^\conj \lr{ i \Hbar c \PD{x}{\psi_1} – m c^2 \psi_2 } \\
&+ \lr{ m c^2 \psi_1^\conj – i \Hbar c \PD{x}{\psi_2^\conj} } \psi_2 \\
&+ \psi_2^\conj \lr{ -m c^2 \psi_1 – i \Hbar c \PD{x}{\psi_2} }.
\end{aligned}
\end{equation}

All the non-derivative terms cancel leaving

\begin{equation}\label{eqn:qmLecture9:420}
\inv{c} \PD{t}{\rho}
=
\PD{x}{\psi_1^\conj} \psi_1
+ \psi_1^\conj \PD{x}{\psi_1}
– \PD{x}{\psi_2^\conj} \psi_2
– \psi_2^\conj \PD{x}{\psi_2}
=
\PD{x}{}
\lr{
\psi_1^\conj \psi_1 –
\psi_2^\conj \psi_2
}.
\end{equation}

Gauge transformed probability current

September 17, 2015 math and physics play , , , ,

[Click here for a PDF of this post with nicer formatting]

Question: Gauge transformed probability current ([1] pr. 2.37 (b))

For the gauge transformed Schrodinger equation

\begin{equation}\label{eqn:gaugeTxCurrent:20}
\inv{2m} \BPi(\Bx) \cdot \BPi(\Bx) \psi(\Bx, t) + e \phi(\Bx) \psi(\Bx, t) = i \Hbar \PD{t}{}\psi(\Bx, t),
\end{equation}

where

\begin{equation}\label{eqn:gaugeTxCurrent:40}
\BPi(\Bx) = -i \Hbar \spacegrad – \frac{e}{c} \BA(\Bx),
\end{equation}

find the probability current defined by

\begin{equation}\label{eqn:gaugeTxCurrent:60}
\PD{t}{\psi} + \spacegrad \cdot \Bj.
\end{equation}

Answer

Equation \ref{eqn:gaugeTxCurrent:20} and its conjugate are

\begin{equation}\label{eqn:gaugeTxCurrent:22}
\begin{aligned}
\inv{2m} \BPi \cdot \BPi \psi + e \phi \psi &= i \Hbar \PD{t}{\psi} \\
\inv{2m} \BPi^\conj \cdot \BPi^\conj \psi^\conj + e \phi \psi^\conj &= -i \Hbar \PD{t}{\psi^\conj}
\end{aligned}
\end{equation}

which can be used immediately in a chain rule expansion of the probability time derivative

\begin{equation}\label{eqn:gaugeTxCurrent:80}
\begin{aligned}
i \Hbar \PD{t}{\rho}
&=
i \Hbar \psi^\conj \PD{t}{\psi} +
i \Hbar \psi \PD{t}{\psi^\conj} \\
&=
\psi^\conj \lr{ \inv{2m} \BPi \cdot \BPi \psi + e \phi \psi } –
\psi \lr{ \inv{2m} \BPi^\conj \cdot \BPi^\conj \psi^\conj + e \phi \psi^\conj
} \\
&=
\inv{2m} \lr{
\psi^\conj \BPi \cdot \BPi \psi
-\psi \BPi^\conj \cdot \BPi^\conj \psi^\conj
}.
\end{aligned}
\end{equation}

We have a difference of conjugates, so can get away with expanding just the first term

\begin{equation}\label{eqn:gaugeTxCurrent:100}
\begin{aligned}
\psi^\conj \BPi \cdot \BPi \psi
&=
\psi^\conj
\psi \\
&=
\psi^\conj
\lr{ -i \Hbar \spacegrad – \frac{e}{c} \BA } \cdot \lr{ -i \Hbar \spacegrad – \frac{e}{c} \BA }
\psi \\
&=
\psi^\conj
\lr{
-\Hbar^2 \spacegrad^2 + \frac{i \Hbar e}{c} \lr{ \BA \cdot \spacegrad + \spacegrad \cdot \BA }
+ \frac{e^2}{c^2} \BA^2
}
\psi.
\end{aligned}
\end{equation}

Note that in the directional derivative terms, the gradient operates on everything to its right, including \( \BA \). Also note that the last term has no imaginary component, so it will not contribute to the difference of conjugates.

This gives

\begin{equation}\label{eqn:gaugeTxCurrent:120}
\begin{aligned}
\psi^\conj \BPi \cdot \BPi \psi – \psi \BPi^\conj \cdot \BPi^\conj \psi^\conj
&=
\psi^\conj
\lr{
-\Hbar^2 \spacegrad^2 \psi + \frac{i \Hbar e}{c} \lr{ \BA \cdot \spacegrad \psi + \spacegrad \cdot (\BA \psi) }
} \\
&\quad –
\psi
\lr{
-\Hbar^2 \spacegrad^2 \psi^\conj – \frac{i \Hbar e}{c} \lr{ \BA \cdot \spacegrad \psi^\conj + \spacegrad \cdot (\BA \psi^\conj) }
} \\
&=
-\Hbar^2 \lr{ \psi^\conj \spacegrad^2 \psi – \psi \spacegrad^2 \psi^\conj } \\
&\quad +
\frac{i \Hbar e}{c}
\lr{
\psi^\conj
\BA \cdot \spacegrad \psi + \psi^\conj \spacegrad \cdot (\BA \psi)
+
\psi
\BA \cdot \spacegrad \psi^\conj + \psi \spacegrad \cdot (\BA \psi^\conj)
}
\end{aligned}
\end{equation}

The first term is recognized as a divergence

\begin{equation}\label{eqn:gaugeTxCurrent:140}
\begin{aligned}
\spacegrad \cdot \lr{ \psi^\conj \spacegrad \psi – \psi \spacegrad \psi^\conj }
&=
\psi^\conj \spacegrad \cdot \spacegrad \psi
+
\spacegrad \psi \cdot \spacegrad \psi^\conj
–
\psi \spacegrad \cdot \spacegrad \psi^\conj
–
\spacegrad \psi^\conj \cdot \spacegrad \psi \\
&= \psi^\conj \spacegrad^2 \psi – \psi \spacegrad^2 \psi^\conj.
\end{aligned}
\end{equation}

The second term can also be factored into a divergence operation

\begin{equation}\label{eqn:gaugeTxCurrent:160}
\begin{aligned}
\psi^\conj
\BA \cdot \spacegrad \psi &+ \psi^\conj \spacegrad \cdot (\BA \psi)
+
\psi
\BA \cdot \spacegrad \psi^\conj + \psi \spacegrad \cdot (\BA \psi^\conj) \\
&=
\lr{ \psi^\conj\BA \cdot \spacegrad \psi
+\psi \spacegrad \cdot (\BA \psi^\conj)
}
+\lr{
\psi \BA \cdot \spacegrad \psi^\conj
+\psi^\conj \spacegrad \cdot (\BA \psi)
} \\
&= 2 \spacegrad \cdot \lr{ \BA \psi \psi^\conj } \\
\end{aligned}
\end{equation}

Putting all the pieces back together we have

\begin{equation}\label{eqn:gaugeTxCurrent:180}
\begin{aligned}
\PD{t}{\rho}
&=
\inv{2m i \Hbar} \lr{
\psi^\conj \BPi \cdot \BPi \psi
-\psi \BPi^\conj \cdot \BPi^\conj \psi^\conj
} \\
&=
\spacegrad \cdot
\inv{2m i \Hbar} \lr{
-\Hbar^2
\lr{ \psi^\conj \spacegrad \psi – \psi \spacegrad \psi^\conj }
+ \frac{ i \Hbar e}{c} 2 \BA \psi \psi^\conj
} \\
&=
\spacegrad \cdot
\lr{
\frac{i \Hbar}{2 m} \lr{ \psi^\conj \spacegrad \psi – \psi \spacegrad \psi^\conj }
+ \frac{e}{m c} \BA \psi \psi^\conj
}.
\end{aligned}
\end{equation}

From \ref{eqn:gaugeTxCurrent:60}, the probability current must be

\begin{equation}\label{eqn:gaugeTxCurrent:200}
\Bj
=
\frac{\Hbar}{2 i m} \lr{ \psi^\conj \spacegrad \psi – \psi \spacegrad \psi^\conj }
– \frac{e}{m c} \BA \psi \psi^\conj,
\end{equation}

or
\begin{equation}\label{eqn:gaugeTxCurrent:220}
\boxed{
\Bj
=
\frac{\Hbar}{m} \textrm{Im} \lr{ \psi^\conj \spacegrad \psi }
– \frac{e}{m c} \BA \psi \psi^\conj.
}
\end{equation}

References

[1] Jun John Sakurai and Jim J Napolitano. Modern quantum mechanics. Pearson Higher Ed, 2014.

Updated notes for ece1229 antenna theory

March 16, 2015 ece1229 , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , ,

I’ve now posted a first update of my notes for the antenna theory course that I am taking this term at UofT.

Unlike most of the other classes I have taken, I am not attempting to take comprehensive notes for this class. The class is taught on slides which go by faster than I can easily take notes for (and some of which match the textbook closely). In class I have annotated my copy of textbook with little details instead. This set of notes contains musings of details that were unclear, or in some cases, details that were provided in class, but are not in the text (and too long to pencil into my book), as well as some notes Geometric Algebra formalism for Maxwell’s equations with magnetic sources (something I’ve encountered for the first time in any real detail in this class).

The notes compilation linked above includes all of the following separate notes, some of which have been posted separately on this blog:

Notes for ece1229 antenna theory

February 4, 2015 ece1229 , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , , ,

I’ve now posted a first set of notes for the antenna theory course that I am taking this term at UofT.

Unlike most of the other classes I have taken, I am not attempting to take comprehensive notes for this class. The class is taught on slides that match the textbook so closely, there is little value to me taking notes that just replicate the text. Instead, I am annotating my copy of textbook with little details instead. My usual notes collection for the class will contain musings of details that were unclear, or in some cases, details that were provided in class, but are not in the text (and too long to pencil into my book.)

The notes linked above include:

  • Reading notes for chapter 2 (Fundamental Parameters of Antennas) and chapter 3 (Radiation Integrals and Auxiliary Potential Functions) of the class text.
  • Geometric Algebra musings.  How to do formulate Maxwell’s equations when magnetic sources are also included (those modeling magnetic dipoles).
  • Some problems for chapter 2 content.

Maxwell’s equations review (plus magnetic sources and currents)

January 28, 2015 ece1229 , , , , , , , , , , ,

[Click here for a PDF of this post with nicer formatting]

These are notes for the UofT course ECE1229, Advanced Antenna Theory, taught by Prof. Eleftheriades, covering ch. 3 [1] content.

Unlike most of the other classes I have taken, I am not attempting to take comprehensive notes for this class. The class is taught on slides that match the textbook so closely, there is little value to me taking notes that just replicate the text. Instead, I am annotating my copy of textbook with little details instead. My usual notes collection for the class will contain musings of details that were unclear, or in some cases, details that were provided in class, but are not in the text (and too long to pencil into my book.)

Maxwell’s equation review

For reasons that are yet to be seen (and justified), we work with a generalization of Maxwell’s equations to include
electric AND magnetic charge densities.

\begin{equation}\label{eqn:chapter3Notes:20}
\spacegrad \cross \boldsymbol{\mathcal{E}} = – \boldsymbol{\mathcal{M}} – \PD{t}{\boldsymbol{\mathcal{B}}}
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:40}
\spacegrad \cross \boldsymbol{\mathcal{H}} = \boldsymbol{\mathcal{J}} + \PD{t}{\boldsymbol{\mathcal{D}}}
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:60}
\spacegrad \cdot \boldsymbol{\mathcal{D}} = \rho
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:80}
\spacegrad \cdot \boldsymbol{\mathcal{B}} = \rho_m.
\end{equation}

Assuming a phasor relationships of the form \( \boldsymbol{\mathcal{E}} =
\text{Real} \lr{ \BE(\Br) e^{j \omega t}} \) for the fields and the currents, these reduce to

\begin{equation}\label{eqn:chapter3Notes:100}
\spacegrad \cross \BE = – \BM – j \omega \BB
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:120}
\spacegrad \cross \BH = \BJ + j \omega \BD
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:140}
\spacegrad \cdot \BD = \rho
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:160}
\spacegrad \cdot \BB = \rho_m.
\end{equation}

In engineering the fields

  • \( \BE \) : Electric field intensity (V/m, Volts/meter).
  • \( \BH \) : Magnetic field intensity (A/m, Amperes/meter).

are designated primary fields, whereas

  • \( \BD \) : Electric flux density (or displacement vector) (C/m, {Coulombs/meter).
  • \( \BB \) : Magnetic flux density (W/m, Webers/meter).

are designated the induced fields. The currents and charges are

  • \( \BJ \) : Electric current density (A/m).
  • \( \BM \) : Magnetic current density (V/m).
  • \( \rho \) : Electric charge density (C/m^3).
  • \( \rho_m \) : Magnetic charge density (W/m^3).

Because \( \spacegrad \cdot \lr{ \spacegrad \cross \Bf } = 0 \) for any
(sufficiently continuous) vector \( \Bf \), divergence relations between the
currents and the charges follow from \ref{eqn:chapter3Notes:100}…

\begin{equation}\label{eqn:chapter3Notes:180}
0
= -\spacegrad \cdot \BM – j \omega \spacegrad \cdot \BB
= -\spacegrad \cdot \BM – j \omega \rho_m,
\end{equation}

and

\begin{equation}\label{eqn:chapter3Notes:200}
0
= \spacegrad \cdot \BJ + j \omega \spacegrad \cdot \BD
= \spacegrad \cdot \BJ + j \omega \rho,
\end{equation}

These are the phasor forms of the continuity equations

\begin{equation}\label{eqn:chapter3Notes:220}
\spacegrad \cdot \BM = – j \omega \rho_m
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:240}
\spacegrad \cdot \BJ = -j \omega \rho.
\end{equation}

Integral forms

The integral forms of Maxwell’s equations follow from Stokes’ theorem and the divergence theorems. Stokes’ theorem is a relation between the integral of the curl and the outwards normal differential area element of a surface, to the boundary of that surface, and applies to any surface with that boundary

\begin{equation}\label{eqn:chapter3Notes:260}
\iint
d\BA \cdot \lr{\spacegrad \cross \Bf}
= \oint \Bf \cdot d\Bl.
\end{equation}

The divergence theorem, a special case of the general Stokes’ theorem is

\begin{equation}\label{eqn:chapter3Notes:280}
\iiint_{V} \spacegrad \cdot \Bf dV
= \iint_{\partial V} \Bf \cdot d\BA,
\end{equation}

where the integral is over the surface of the volume, and the area element of the bounding integral has an outwards normal orientation.

See [5] for a derivation of this and various generalizations.

Applying these to Maxwell’s equations gives

\begin{equation}\label{eqn:chapter3Notes:320}
\oint d\Bl \cdot \BE = –
\iint d\BA \cdot \lr{
\BM + j \omega \BB
}
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:340}
\oint d\Bl \cdot \BH =
\iint d\BA \cdot \lr{
\BJ + j \omega \BD
}
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:360}
\iint_{\partial V} d\BA \cdot \BD = \iiint \rho dV
\end{equation}
\begin{equation}\label{eqn:chapter3Notes:380}
\iint_{\partial V} d\BA \cdot \BB = \iiint \rho_m dV
\end{equation}

Constitutive relations

For linear isotropic homogeneous materials, the following constitutive relations apply

  • \( \BD = \epsilon \BE \)
  • \( \BB = \mu \BH \)
  • \( \BJ = \sigma \BE \), Ohm’s law.

where

  • \( \epsilon = \epsilon_r \epsilon_0\), is the permutivity (F/m, Farads/meter ).
  • \( \mu = \mu_r \mu_0 \), is the permeability (H/m, Henries/meter), \( \mu_0 = 4 \pi \times 10^{-7} \).
  • \( \sigma \), is the conductivity (\( \inv{\Omega m}\), where \( 1/\Omega \) is a Siemens.)

In AM radio, will see ferrite cores with the inductors, which introduces non-unit \( \mu_r \). This is to increase the radiation resistance.

Boundary conditions

For good electric conductor \( \BE = 0 \).
For good magnetic conductor \( \BB = 0 \).

(more on class slides)

Linear time invariant

Linear time invariant meant that the impulse response \( h(t,t’) \) was a function of just the difference in times \( h(t,t’) = h(t-t’) \).

Green’s functions

For electromagnetic problems the impulse function sources \( \delta(\Br – \Br’) \) also has a direction, and can yield any of \( E_x, E_y, E_z \). A tensor impulse response is required.

Some overview of an approach that uses such tensor Green’s functions is outlined on the slides. It gets really messy since we require four tensor Green’s functions to handle electric and magnetic current and charges. Because of this complexity, we don’t go down this path, and use potentials instead.

In \S 3.5 [1] and the class notes, a verification of the spherical wave form for the Helmholtz Green’s function was developed. This was much simpler than the same verification I did in [4]. Part of the reason for that was that I worked in Cartesian coordinates, which made things much messier. The other part of the reason, for treating a neighbourhood of \( \Abs{\Br – \Br’} \sim 0 \), I verified the convolution, whereas Prof. Eleftheriades argues that a verification that \( \int \lr{\spacegrad^2 + k^2} G(\Br, \Br’) dV’ = 1\) is sufficient. Balanis, on the other hand, argues that knowing the solution for \( k \ne 0 \) must just be the solution for \( k = 0 \) (i.e. the Poisson solution) provided it is multiplied by the \( e^{-j k r} \) factor.

Note that back when I did that derivation, I used a different sign convention for the Green’s function, and in QM we used a positive sign instead of the negative in \( e^{-j k r } \).

Notation

  • Phasor frequency terms are written as \( e^{j \omega t} \), not \( e^{-j \omega t} \), as done in physics. I didn’t recall that this was always the case in physics, and wouldn’t have assumed it. This is the case in both [3] and [2]. The latter however, also uses \( \cos(\omega t – k r) \) for spherical waves possibly implying an alternate phasor sign convention in that content, so I’d be wary about trusting any absolute “engineering” vs. physics sign convention without checking carefully.
  • In Green’s functions \( G(\Br, \Br’) \), \( \Br \) is the point of observation, and \( \Br’ \) is the point in the convolution integration space.
  • Both \( \BM \) and \( \BJ_m \) are used for magnetic current sources in the class notes.

References

[1] Constantine A Balanis. Antenna theory: analysis and design. John Wiley \& Sons, 3rd edition, 2005.

[2] David Jeffrey Griffiths and Reed College. Introduction to electrodynamics, chapter {Electromagnetic Waves}. Prentice hall Upper Saddle River, NJ, 3rd edition, 1999.

[3] JD Jackson. Classical Electrodynamics, chapter {Simple Radiating Systems, Scattering, and Diffraction}. John Wiley and Sons, 2nd edition, 1975.

[4] Peeter Joot. Quantum Mechanics II., chapter {Verifying the Helmholtz Green’s function.} peeterjoot.com, 2011. URL https://peeterjoot.com/archives/math2011/phy456.pdf. [Online; accessed 28-January-2015].

[5] Peeter Joot. Exploring physics with Geometric Algebra, chapter {Stokes theorem}. peeterjoot.com, 2014. URL https://peeterjoot.com/archives/math2009/gabook.pdf. [Online; accessed 28-January-2015].